
Chapter 8 Ab Initio Treatments of Polyatomic Molecules 8.1 Ab Initio Methods andand Semiemprical Methods Semiempirical and Ab initio Methods Molecular quantum-mechanical methods are classified as either ab initio or semiempirical. Semiempirical methods use a simple Hamiltonian than the correct molecular Hamiltionian and use parameters whose values are adjusted to fit experimental data or the results of ab initio calculations. HMO,EHMO,CNDO,... An ab initio calculation uses the correct Hamiltonian and does not use experimental data other than the values of the fundamental physical constants.(Ab initio is Latin for"from the beginning"and indicates a calculation based on fundamental principles.) Hartree-Fock SCF calculation: Basis set→Atomic orbital→Molecular orbital→Slater determinant →Iterative solution. Classification of Electronic Terms of Polyatomic Molecules Linear Molecules: The operator L:for the axial component of the total electronic orbital momentum commutes with the electronic Hamitionian,and the same classifications are used as for diatomic molecules;we have such possibilities as'∑*,l∑,3Σ,l'L,and so on.For linear polyatomic molecules with a center of symmetry,the g.u classification is added. Nonlinear polyatomic molecules: There is no orbital angular-momentum operator that commutes with the electronic Hamiltionian,and the angular momentum classification of electronic terms cannot be used.Operators that do commute with the electronic Hamiltionian are the symmetry operators OR of the molecule. and the electronic states of polyatomic molecules are classified according
Chapter 8 Ab Initio Treatments of Polyatomic Molecules 8.1 Ab Initio Methods and and Semiemprical Methods Semiempirical and Ab initio Methods Molecular quantum-mechanical methods are classified as either ab initio or semiempirical. Semiempirical methods use a simple Hamiltonian than the correct molecular Hamiltionian and use parameters whose values are adjusted to fit experimental data or the results of ab initio calculations. HMO, EHMO, CNDO, … An ab initio calculation uses the correct Hamiltonian and does not use experimental data other than the values of the fundamental physical constants. (Ab initio is Latin for “from the beginning” and indicates a calculation based on fundamental principles.) Hartree-Fock SCF calculation: Basis set → Atomic orbital → Molecular orbital → Slater determinant → Iterative solution … Classification of Electronic Terms of Polyatomic Molecules Linear Molecules: The operator Lz for the axial component of the total electronic orbital momentum commutes with the electronic Hamitionian, and the same classifications are used as for diatomic molecules; we have such possibilities as 1 Σ+ , 1 Σ- , 3 Σ+ , 1 Π, and so on. For linear polyatomic molecules with a center of symmetry, the g, u classification is added. Nonlinear polyatomic molecules: There is no orbital angular-momentum operator that commutes with the electronic Hamiltionian, and the angular momentum classification of electronic terms cannot be used. Operators that do commute with the electronic Hamiltionian are the symmetry operators OR of the molecule, and the electronic states of polyatomic molecules are classified according

to the behavior of the electronic wave function on application of these operators Consider H2O as an example. In its equilibrium configuration,water belongs to group Car with the symmetry operators EC2(z)o,(Xz)oyz) E C2(z)(xZ)(yz) N 11 1 1 1 1 -1 1 -1 1 -1 B2 -1 -1 1 Consider an operator R that commutes with the molecular Hamiltonian H that does not involve spin;we have RH=HR RHΨ=REΨ HR)=E(RΨ) (8.1) so that R'is an eigenfunction of H with eigenvalue E.We have RΨ=λΨ (8.2) Here must be an eigenfunction of the symmetry operator R.Thus,the electronic states of polyatomic molecules can be classified according to the symmetry of the electronic wave function associated with the molecular point group.For the term classification of H2O,we have such possibilities as A,A2.B,B2,A,etc. As an example,the possible symmetry species of a Dh molecule to be
to the behavior of the electronic wave function on application of these operators. Consider H2O as an example. In its equilibrium configuration, water belongs to group C2V with the symmetry operators E C2(z) σv(xz) σv(yz) H1 H2 O y z E C2(z) σv(xz) σv(yz) A1 A2 B1 B2 1 1 1 1 1 1 1 1 -1 -1 -1 1 -1 -1 -1 1 Consider an operator R that commutes with the molecular Hamiltonian H that does not involve spin; we have RH = HR RHΨ = REΨ H(RΨ) = E(RΨ) (8.1) so that RΨ is an eigenfunction of H with eigenvalue E. We have RΨ = λΨ (8.2) Here Ψ must be an eigenfunction of the symmetry operator R. Thus, the electronic states of polyatomic molecules can be classified according to the symmetry of the electronic wave function associated with the molecular point group. For the term classification of H2O, we have such possibilities as 1 A1, 1A2, 1 B1, 1 B2, 3 A1, etc. As an example, the possible symmetry species of a D6h molecule to be

Alg,A2g Big,B2g,Eig E2g Alu A2u Blu B2us Elu E2u 8.2 The SCF MO Treatment of Polyatomic Molecules The purely electronic nonrelativistic Hamiltonian for a polyatomic molecule is(in atomic units): 户=-Σ:-∑Σ2+2Σ1 (8.3) Best possible variation function-Hartree-Fock SCF function:The form of an antisymmetrized product of spin-orbitals.The MOs are usually expressed as linear combinations of basis functions,the coefficients being found by solution of the Roothaan equations. A sufficiently large basis set-Accurate approximations to the Hartree-Fock MO's. A minimal basis set->only rough approximations to the Hartree-Fock MO's,still referred to as SCF molecular orbitals. Classification of Molecular Orbitals As might be expected,the MOs of a polyatomic molecule show the same kinks of possible symmetry behavior as the electronic wavefunction does.The MOs are therefore classified according to the symmetry species of the molecular point group. For example,the MOs of H2O have the possible symmetry species a,a2, b1,and b2.Lowercase letters are used for MO symmetry species.To distinguish MOs of the same symmetry species,we number them in order of increasing energy.1a,2a,3a,1b,2b1,. For benzene C6H6,there are some doubly degenerate symmetry species, so some of the benzene MOs occur in having the same energy. Specification of the number of electrons in each MO specifies the
A1g, A2g, B1g, B2g, E1g, E2g A1u, A2u, B1u, B2u, E1u, E2u 8.2 The SCF MO Treatment of Polyatomic Molecules The purely electronic nonrelativistic Hamiltonian for a polyatomic molecule is (in atomic units): ∑ ∑∑ ∑∑ > ∧ = − ∇ − + i i i i i j ij i el r r Z H 1 2 1 2 α α α (8.3) Best possible variation function — Hartree-Fock SCF function: The form of an antisymmetrized product of spin-orbitals. The MOs are usually expressed as linear combinations of basis functions, the coefficients being found by solution of the Roothaan equations. A sufficiently large basis set → Accurate approximations to the Hartree-Fock MO’s. A minimal basis set → only rough approximations to the Hartree-Fock MO’s, still referred to as SCF molecular orbitals. Classification of Molecular Orbitals As might be expected, the MOs of a polyatomic molecule show the same kinks of possible symmetry behavior as the electronic wavefunction does. The MOs are therefore classified according to the symmetry species of the molecular point group. For example, the MOs of H2O have the possible symmetry species a1, a2, b1, and b2. Lowercase letters are used for MO symmetry species. To distinguish MOs of the same symmetry species, we number them in order of increasing energy. 1a1, 2a1, 3a1, …; 1b1, 2b1, … For benzene C6H6, there are some doubly degenerate symmetry species, so some of the benzene MOs occur in having the same energy. Specification of the number of electrons in each MO specifies the

molecular electronic configuration.For example,an (eg)configuration of Doh molecule gives the termsAg E2g,andA2g. A closed-shell configuration gives rise to a single nondegenerate term whose multiplicity is I and whose symmetry species is the totally symmetric. SCF-MO Wave Functions for Open-Shell States. For SCF MO calculations of closed-shell states of molecules and atoms,electrons paired with each other almost given precisely the same spatial orbital function.A Hartree-Fock wave function in which electrons whose spins are paired occupy the same spatial orbital is called a restricted Hartree-Fock(RHF)wave function For open-shell states,two different approaches are widely used. Restricted Open-Shell Hartree-Fock(ROHF)Method In the ROHF method,electrons that are paired with each other are given the same spatial orbital function.For example,the ROHF wave function of the Li ground state is |sls2s1,where the two Is electrons occupy the same spatial MO.The 2s electron in this ROHF function has been given spin a. Restricted Open-Shell Hartree-Fock(ROHF)Method Since electron with the same spin tend to keep away from each other (Pauli repulsion),the interaction between the 2sa and Isa electrons differs from the interaction between the 2sa and IsB electrons,and it seems reasonable to give the two Is electrons slightly different spatial orbitals,which we call Is and Is'.This gives the unrestricted Hartree-Fock (UHF)wave function for the Li ground state as |1s1s'2s, where Is Is'.In a UHF wave function,the spatial orbitals of spin-a electrons are allowed to differ from those of spin-B electrons Comparison of ROHF with UHF The UHF wave function gives a slightly lower energy than the ROHF wave function and is much more useful in predicting
molecular electronic configuration. For example, an (e1g) 2 configuration of D6h molecule gives the terms 1 A1g, 1 E2g, and 3 A2g. A closed-shell configuration gives rise to a single nondegenerate term whose multiplicity is 1 and whose symmetry species is the totally symmetric. SCF-MO Wave Functions for Open-Shell States. For SCF MO calculations of closed-shell states of molecules and atoms, electrons paired with each other almost given precisely the same spatial orbital function. A Hartree-Fock wave function in which electrons whose spins are paired occupy the same spatial orbital is called a restricted Hartree-Fock (RHF) wave function. For open-shell states, two different approaches are widely used. Restricted Open-Shell Hartree-Fock (ROHF) Method In the ROHF method, electrons that are paired with each other are given the same spatial orbital function. For example, the ROHF wave function of the Li ground state is |1s1s2s |, where the two 1s electrons occupy the same spatial MO. The 2s electron in this ROHF function has been given spin α. Restricted Open-Shell Hartree-Fock (ROHF) Method Since electron with the same spin tend to keep away from each other (Pauli repulsion), the interaction between the 2sα and 1sα electrons differs from the interaction between the 2sα and 1sβ electrons, and it seems reasonable to give the two 1s electrons slightly different spatial orbitals, which we call 1s and 1s′. This gives the unrestricted Hartree-Fock (UHF) wave function for the Li ground state as |1s1s'2s | , where 1s ≠ 1s′. In a UHF wave function, the spatial orbitals of spin-α electrons are allowed to differ from those of spin-β electrons. Comparison of ROHF with UHF The UHF wave function gives a slightly lower energy than the ROHF wave function and is much more useful in predicting
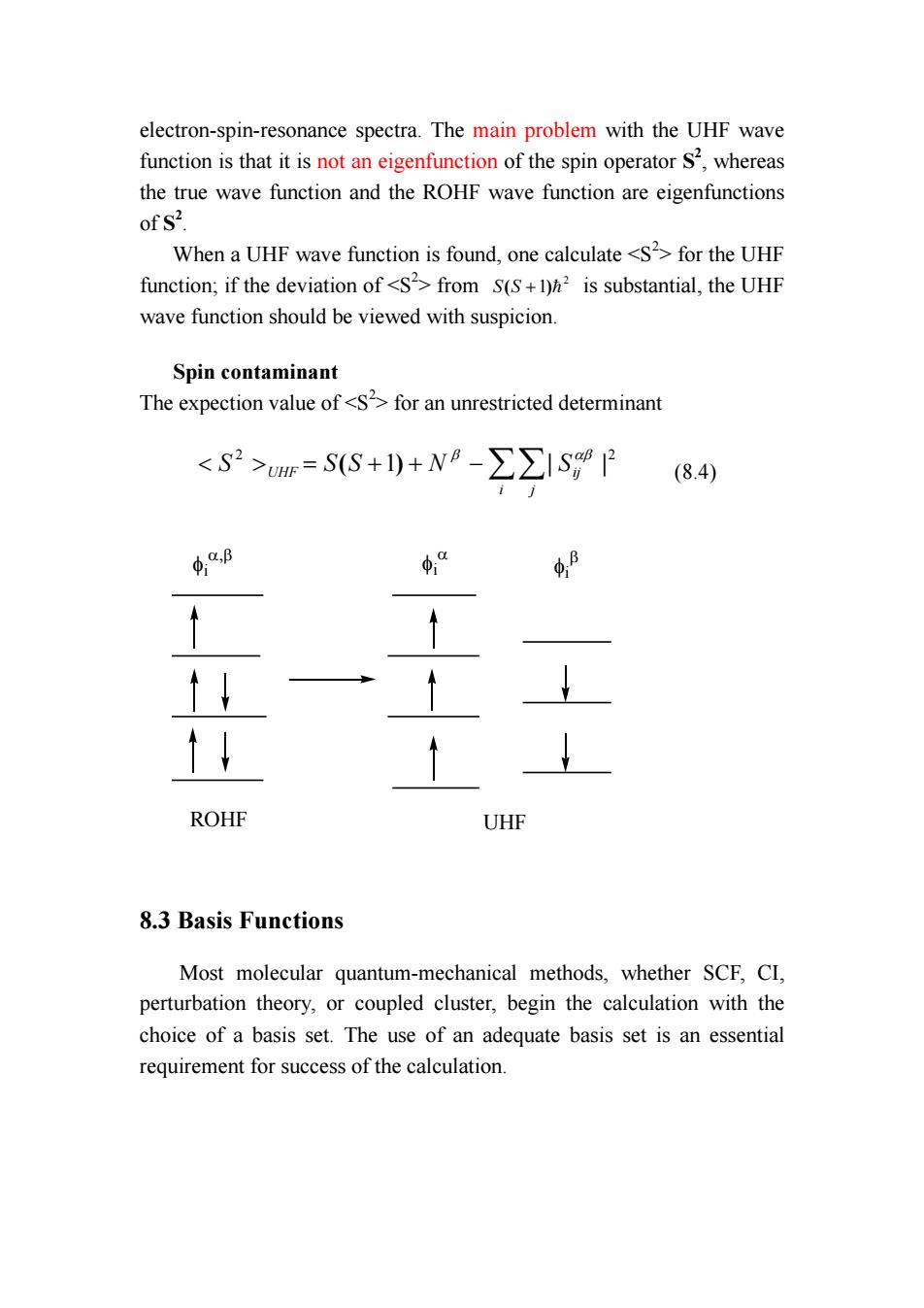
electron-spin-resonance spectra.The main problem with the UHF wave function is that it is not an eigenfunction of the spin operator S2,whereas the true wave function and the ROHF wave function are eigenfunctions of S2. When a UHF wave function is found,one calculate <S2>for the UHF function;if the deviation of <S2>from S(S+)h is substantial,the UHF wave function should be viewed with suspicion Spin contaminant The expection value of<Sfor an unrestricted determinant <S2>=SS+1)+N8-∑∑ISP84 4.B φ“ ↑↓ ROHF UHF 8.3 Basis Functions Most molecular quantum-mechanical methods,whether SCF,CI, perturbation theory,or coupled cluster,begin the calculation with the choice of a basis set.The use of an adequate basis set is an essential requirement for success of the calculation
electron-spin-resonance spectra. The main problem with the UHF wave function is that it is not an eigenfunction of the spin operator S2 , whereas the true wave function and the ROHF wave function are eigenfunctions of S2 . When a UHF wave function is found, one calculate <S2 > for the UHF function; if the deviation of <S2 > from 2 S(S +1)= is substantial, the UHF wave function should be viewed with suspicion. Spin contaminant The expection value of <S2 > for an unrestricted determinant 2 2 < > = ( +1) + −∑∑| | i j S UHF S S N Sij β αβ (8.4) ROHF UHF φi α,β φi α φi β 8.3 Basis Functions Most molecular quantum-mechanical methods, whether SCF, CI, perturbation theory, or coupled cluster, begin the calculation with the choice of a basis set. The use of an adequate basis set is an essential requirement for success of the calculation