
How many different vibrations with different k's? For travelling waves,use periodic boundary condition PBC:Uo(t)=uN(t) Consider a short 1-dim lattice with only 8 atoms w+m=儿n 1eto-]eta-mg) =2 e-iNag =1 2π a.a是2 The value of k,like x,is discretized △k=2/Na→0asN→∞ Each k describes a normal mode of vibration(i.e.a vibration with a specific frequency)

q is restricted to be some separated values.In the q axis,the distance between neighboring q should be 2/Na.Every q occupies the space of 2x/Na in the q axis So,the distribution density of q should be p(9)= Na L L=Na the length of the chain 2π2π The number of The total number of lattice vibration modes 2z p(q)-2 πNa=N a 2π primitive cells 12f 1.0 q→0,elastic waves 0.8 4B 0.6 m m 0.4 02F sing≈ 0 2 2 第 布里渊区 Equal to elastic waves Ba 0三 -aq 0口 m
q is restricted to be some separated values. In the q axis, the distance between neighboring q should be 2π/Na.Every q occupies the space of 2π/Na in the q axis So, the distribution density of q should be ( ) 2 2 Na L q = = L=Na the length of the chain 2 2 ( ) 2 Na q N a a = = The number of primitive cells The total number of lattice vibration modes q q → 0 , elastic waves sin 2 2 qa qa aq m = s Y a v m a = = Equal to elastic waves 4 m sin 2 qa

4.1.2 Diatomic Chain of Two Different Atom Types with nearest-neighbor,Hooke's Law type forces(F=-kx)between the atoms.This is equivalent to a force-spring model with two different types of atoms of masses,M&m connected by identical springs of spring constant K. 2) -1) (n (止1) ①+2) MwM一金VWMM MM—MM 2a -M Up1 U*2 This is the simplest possible model of a diatomic crystal 2a is the repeat distance,so,the nearest-neighbor separation is a
• This is the simplest possible model of a diatomic crystal. • 2a is the repeat distance, so, the nearest-neighbor separation is a 4.1.2 Diatomic Chain of Two Different Atom Types with nearest-neighbor, Hooke’s Law type forces (F = - kx) between the atoms. This is equivalent to a force-spring model with two different types of atoms of masses, M & m connected by identical springs of spring constant K

This model is complicated due to the presence of 2 different atom types,which,in general,move in opposite directions. M d=B(4nl+4xl-2,) dr2 dat=p(n+4x2-2n.l) Assume: un Aei(or-2nag) ② 4n+1=Be[o-(2n+1)ag] [(Mo2-2B)A+2Bcos(aq)B=0 ③ 2Bcos(aq)A+(mo2-2B)B=0
1 1 ( 2 ) = − K u u u n n n + − + • This model is complicated due to the presence of 2 different atom types, which, in general, move in opposite directions. ( ) 2 2 2 2 1 2 1 d 2 d n n n n u M u u u t = + − + − ( ) 2 2 1 2 2 2 2 2 1 d 2 d n n n n u m u u u t + = + − + + ① ( ) ( ) 2 M A aq B − + = 2 2 cos 0 ( ) ( ) 2 2 cos 2 0 aq A m B + − = ③ ( 2 ) 2 i t naq u Ae n − = (2 1) 2 1 i t n aq u Be n − + + = ② Assume:
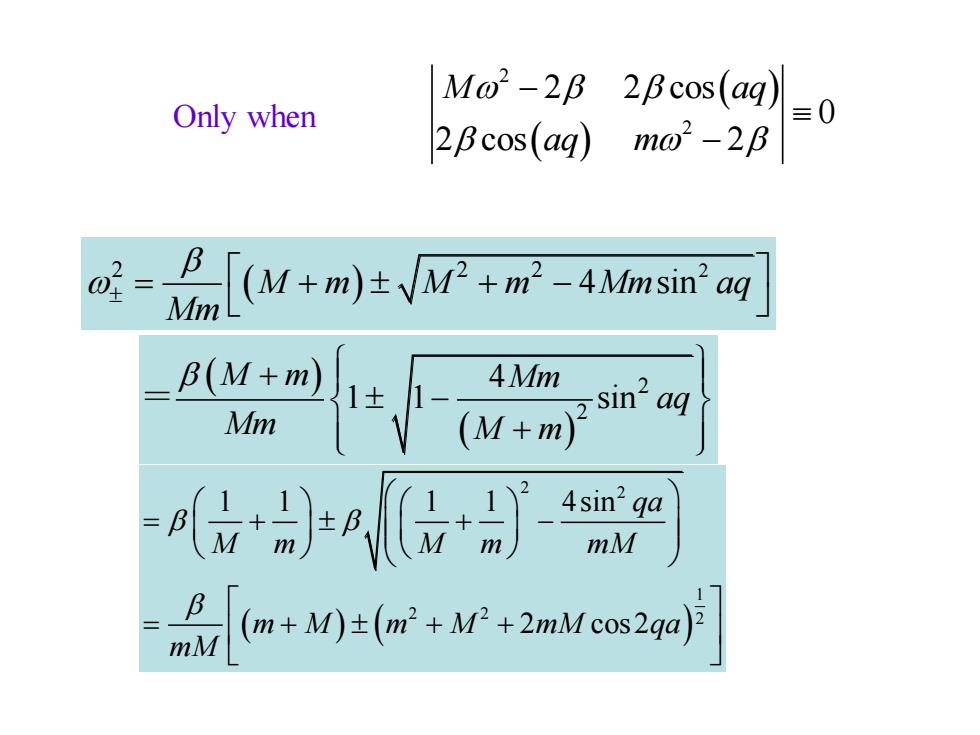
Mo2-2B 2B cos(aq) Only when =0 2Bcos(ag)mo2-2B (M+m)M2+m2 -4Mmsin'ag 4Mm Mm 位a 4sin'qa mM (m+M0)±(m+M+2 mMcos2qa) 2 mM
( ) ( ) 2 2 2 2 cos 0 2 cos 2 M aq aq m − − ( ) 2 2 2 2 M m M m Mm aq 4 sin Mm = + + − ( ) ( ) 2 2 4 1 1 sin M m Mm aq Mm M m + − + = ( ) ( ) 2 2 1 2 2 2 1 1 1 1 4sin 2 cos2 qa M m M m mM m M m M mM qa mM = + + − = + + + Only when