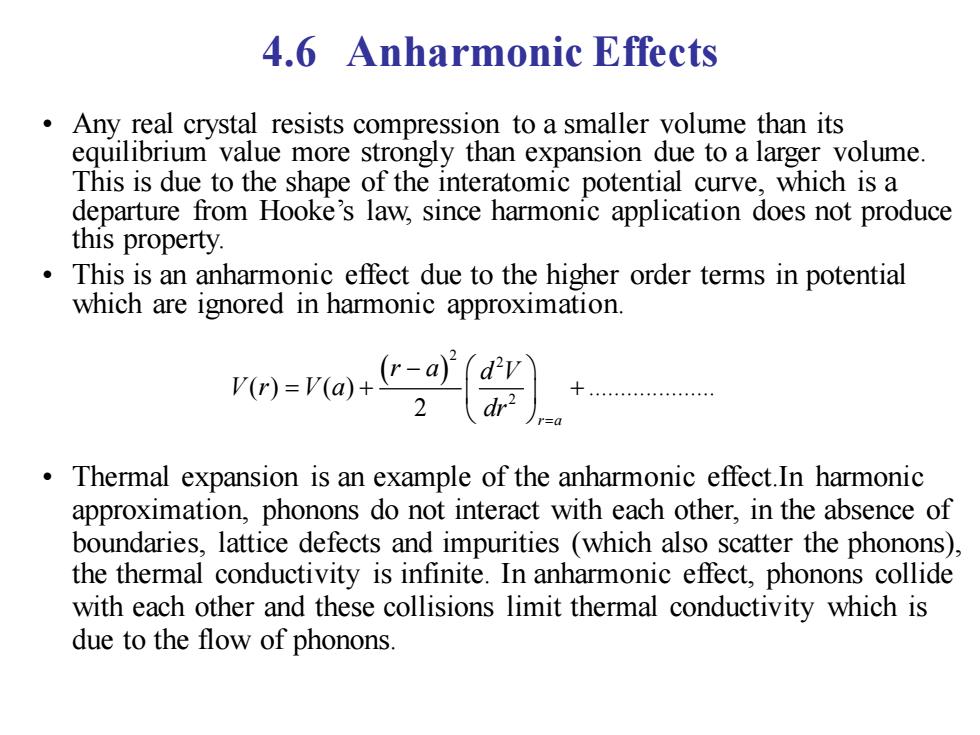
4.6 Anharmonic Effects Any real crystal resists compression to a smaller volume than its equilibrium value more strongly than expansion due to a larger volume. This is due to the shape of the interatomic potential curve,which is a departure from Hooke's law,since harmonic application does not produce this property. This is an anharmonic effect due to the higher order terms in potential which are ignored in harmonic approximation. o=ra+-a() Thermal expansion is an example of the anharmonic effect.In harmonic approximation,phonons do not interact with each other,in the absence of boundaries,lattice defects and impurities (which also scatter the phonons), the thermal conductivity is infinite.In anharmonic effect,phonons collide with each other and these collisions limit thermal conductivity which is due to the flow of phonons
4.6 Anharmonic Effects • Any real crystal resists compression to a smaller volume than its equilibrium value more strongly than expansion due to a larger volume. This is due to the shape of the interatomic potential curve, which is a departure from Hooke’s law, since harmonic application does not produce this property. • This is an anharmonic effect due to the higher order terms in potential which are ignored in harmonic approximation. • Thermal expansion is an example of the anharmonic effect.In harmonic approximation, phonons do not interact with each other, in the absence of boundaries, lattice defects and impurities (which also scatter the phonons), the thermal conductivity is infinite. In anharmonic effect, phonons collide with each other and these collisions limit thermal conductivity which is due to the flow of phonons.( ) 2 2 2 ( ) ( ) . 2 r a r a d V V r V a dr = − = + +
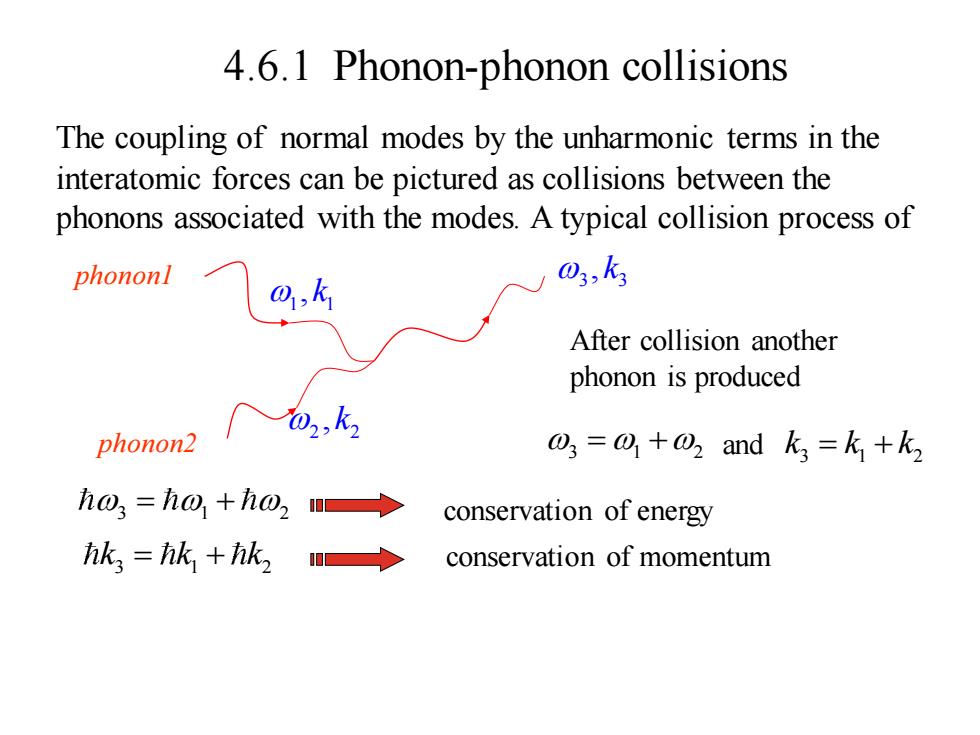
4.6.1 Phonon-phonon collisions The coupling of normal modes by the unharmonic terms in the interatomic forces can be pictured as collisions between the phonons associated with the modes.A typical collision process of phononl 03,k3 After collision another phonon is produced 2,k2 phonon2 o3=01+02andk3=k+k2 ho,=ho,+hw2→ conservation of energy hks =hk +hk conservation of momentum
4.6.1 Phonon-phonon collisions The coupling of normal modes by the unharmonic terms in the interatomic forces can be pictured as collisions between the phonons associated with the modes. A typical collision process of phonon1 phonon2 1 1 , k 2 2 , k 3 3 , k After collision another phonon is produced 3 1 2 k k k = + 3 1 2 k k k = + 3 1 2 = + 3 1 2 = + and conservation of energy conservation of momentum

Phonons are represented by wavenumbers with -亚≤k<匹 a Ifk lies outside this range add a suitable multible of to bring it back within the range of.Then,=becomes This phonon is indistinguishable from a phonon with wavevector k π=k+k3 a where k,k,and k are all in the above range. Longitudinal Transverse d a n≠0→Umklapp process n=0→Normal process (due to anharmonic effects) Phonon3has Phonon3has k> -and Phonon3=Phonon3' a
Phonon3 has k a ; Phonon3 has k and Phonon3=Phonon3’ a 1 2 k a − 0 a 3' Umklapp process (due to anharmonic effects) 3 1 2 k a − 0 a Normal process Longitudinal 3 Transverse n = 0 n 0 Phonons are represented by wavenumbers with k a a − If lies outside this range add a suitable multible of to bring it back within the range of . Then, becomes 3 k 3 1 2 n2 k k k a = + where , , and are all in the above range. 2 a 3 1 2 k k k k = + a a − 2 k 3 k 1 k This phonon is indistinguishable from a phonon with wavevector 3 k
4.6.2 Thermal conduction by phonons A flow of heat takes place from a hotter region to a cooler region when there is a temperature gradient in a solid.The most important contribution to thermal conduction comes from the flow of phonons in an electrically insulating solid. Transport property is an example of thermal conduction. 。 Transport property is the process in which the flow of some quantity occurs. Thermal conductivity is a transport coefficient and it describes the flow. The thermal conductivity of a phonon gas in a solid will be calculated by means of the elementary kinetic theory of the transport coefficients of gases
4.6.2 Thermal conduction by phonons • A flow of heat takes place from a hotter region to a cooler region when there is a temperature gradient in a solid. The most important contribution to thermal conduction comes from the flow of phonons in an electrically insulating solid. • Transport property is an example of thermal conduction. • Transport property is the process in which the flow of some quantity occurs. • Thermal conductivity is a transport coefficient and it describes the flow. • The thermal conductivity of a phonon gas in a solid will be calculated by means of the elementary kinetic theory of the transport coefficients of gases
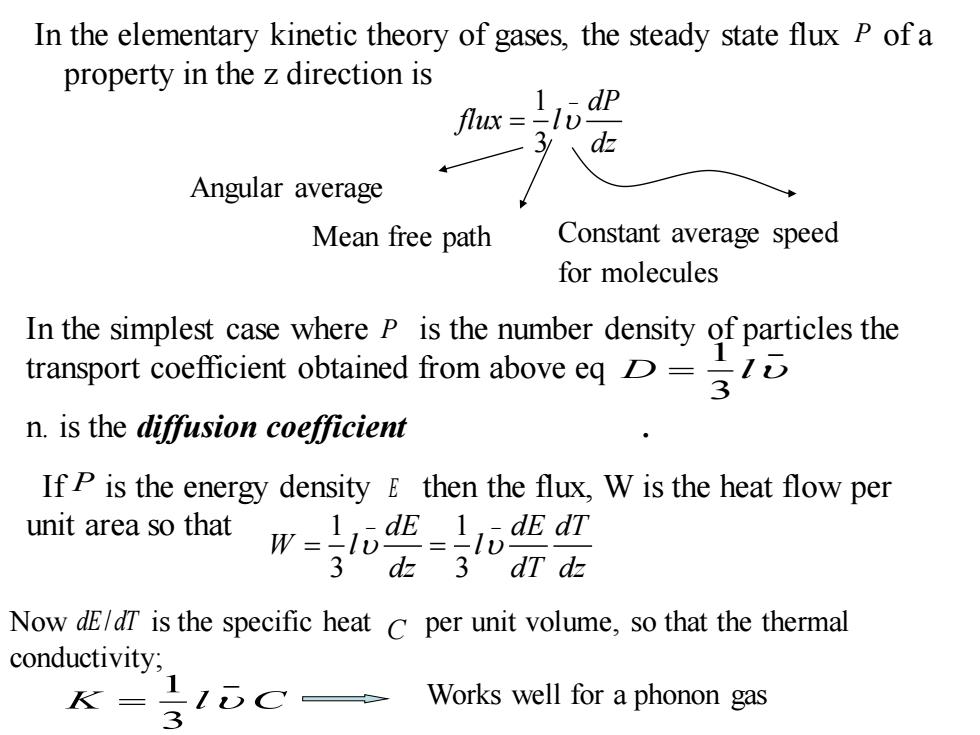
In the elementary kinetic theory of gases,the steady state flux P of a property in the z direction is =118 dp z Angular average Mean free path Constant average speed for molecules In the simplest case where P is the number density of particles the transport coefficient obtained from above q n.is the diffusion coefficient If P is the energy density E then the flux,W is the heat flow per unit area so that m=ln-=l元aedr 3 dz 3 dT dz Now dE/dT is the specific heat c per unit volume,so that the thermal conductivity; K- 1C Works well for a phonon gas 3
In the elementary kinetic theory of gases, the steady state flux of a property in the z direction is P _ 1 3 dP flux l dz = Mean free path Angular average Constant average speed for molecules In the simplest case where is the number density of particles the transport coefficient obtained from above eq n. is the diffusion coefficient . If is the energy density then the flux, W is the heat flow per unit area so that _ _ 1 1 3 3 dE dE dT W l l dz dT dz = = Now is the specific heat per unit volume, so that the thermal conductivity; 1 _ 3 K l C = P 1 _ 3 D l = P E dE dT / C Works well for a phonon gas