
Chapter 5 band theory Objectives At the end of this Chapter,you should: 1.Be able to understand the Bloch's Theorem. 2.Know how to calculate the 1D band gap using NFE model 3.Be able to calculate the band gap and band width of a crystal using the TBA model. 4.Have established the concept of band symmetry based on the crystal structure symmetry. 5.Know how to calculate the density of states 6.Understand the concept of Fermi surface
Objectives At the end of this Chapter, you should: 1.Be able to understand the Bloch’s Theorem. 2. Know how to calculate the 1D band gap using NFE model. 3. Be able to calculate the band gap and band width of a crystal using the TBA model. 4. Have established the concept of band symmetry based on the crystal structure symmetry. 5. Know how to calculate the density of states. 6. Understand the concept of Fermi surface. Chapter 5 band theory

5.1 general background and approximations Band theory is to study the solid state electronics,and is the most important theoretical basis for solids,which is the most direct and important results of the quantum mechanics and quantum statistics when applied to the solid.Band theory not only succeeds in solving many problems which is unsolved by the classical electron theory and Sommerfeld free-electron theory,and explain all the crystal properties History: 1)First proposed in 1928(23y),in his doctoral dissertation"on the quantum mechanics in lattice",Bloch first put forward the concept. 2)In 1931,Wilson illustrates the difference between insulators and metal using the band theory and lay the foundation of theory of semiconductor physics. 3)improve the band. The band theory is stricter than the free electrons theory,but it is still an approximate theory
Band theory is to study the solid state electronics, and is the most important theoretical basis for solids, which is the most direct and important results of the quantum mechanics and quantum statistics when applied to the solid. Band theory not only succeeds in solving many problems which is unsolved by the classical electron theory and Sommerfeld free-electron theory, and explain all the crystal properties History: 1) First proposed in 1928(23y), in his doctoral dissertation "on the quantum mechanics in lattice " , Bloch first put forward the concept. 2) In 1931, Wilson illustrates the difference between insulators and metal using the band theory and lay the foundation of theory of semiconductor physics. 3) improve the band . The band theory is stricter than the free electrons theory, but it is still an approximate theory 5.1 general background and approximations
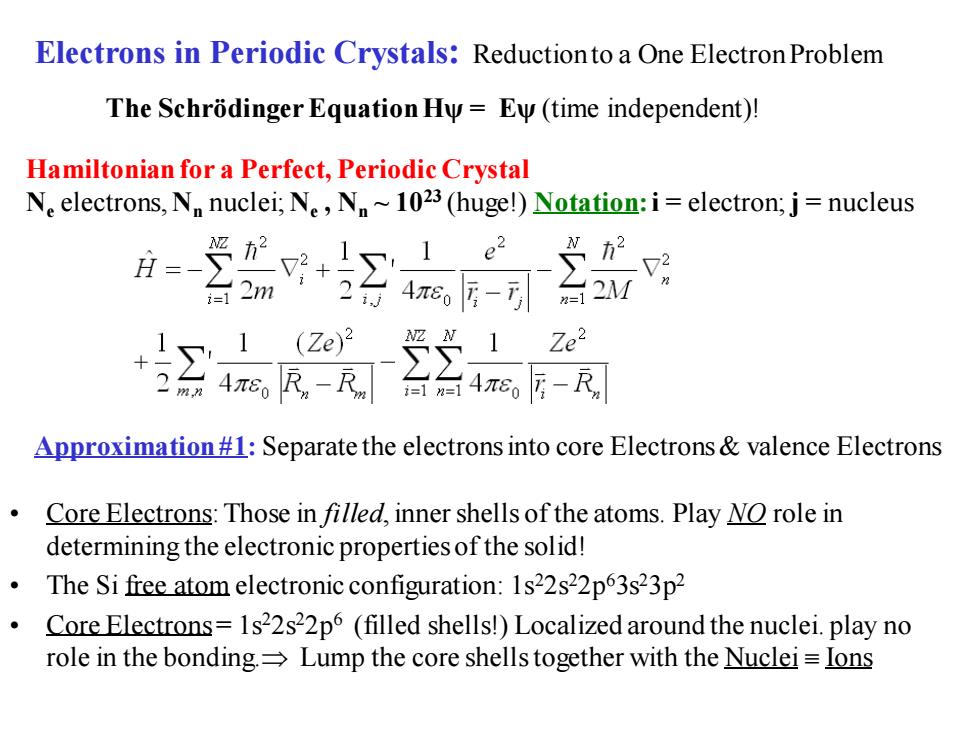
Electrons in Periodic Crystals:Reductionto a One Electron Problem The Schrodinger Equation Hy=Ey(time independent)! Hamiltonian for a Perfect,Periodic Crystal Ne electrons,Nn nuclei;Ne,N~1023 (huge!)Notation:i=electron;j=nucleus 月-+Σ e2 台2m 248,后-3 1 Ze2 4R-R台4心 Approximation#1:Separate the electrons into core Electrons valence Electrons Core Electrons:Those in filled,inner shells of the atoms.Play NO role in determining the electronic properties of the solid! The Si free atom electronic configuration:1s22s22p63s23p2 Core Electrons=1s22s22p6 (filled shells!)Localized around the nuclei.play no role in the bonding.=Lump the core shells together with the Nuclei=Ions
Electrons in Periodic Crystals: Reduction to a One Electron Problem The SchrödingerEquation Hψ = Eψ (time independent)! Hamiltonian for a Perfect, Periodic Crystal Ne electrons, Nn nuclei; Ne , Nn ~ 1023 (huge!) Notation:i = electron; j = nucleus Approximation #1: Separate the electrons into core Electrons & valence Electrons • Core Electrons: Those in filled, inner shells of the atoms. Play NO role in determining the electronic properties of the solid! • The Si free atom electronic configuration: 1s22s22p63s23p2 • Core Electrons= 1s22s22p6 (filled shells!) Localized around the nuclei. play no role in the bonding. Lump the core shells together with the Nuclei Ions

Valence Electrons in the unfilled,outer shells of the free atoms.Si free atom electron configuration:1s22s22p63s23p2 Valence Electrons 3s23p2 (unfilled shell!) In the solid,these hybridize with electrons on neighbor atoms. Approximation #2 The Born-Oppenheimer (Adiabatic)Approximation Separates the electron ion motions. A Qualitative (semiquantitative)Justification:the ratio of the electron ion masses is of the order:(m/Mi)~10-3 (<<1)=Classically,the massive ions move much slower than the very small mass electrons! Typical ionic vibrational frequencies:v:~1013 sThe time scale of the ion motion is:t10-13 s.Electronic motion occurs at energies of about a bandgap:Eg=hve =ho~1ev ve~1015 s1=te~10-15s.So,The electrons respond to the ion motion ~instantaneously! In the electron Hamiltonian,He the ions can be treated as stationary!
Valence Electrons in the unfilled, outer shells of the free atoms. Si free atom electron configuration: 1s22s22p63s23p2 Valence Electrons = 3s23p2 (unfilled shell!) • In the solid, these hybridize with electrons on neighbor atoms. Approximation #2 The Born-Oppenheimer (Adiabatic) Approximation Separates the electron & ion motions. A Qualitative (semiquantitative) Justification: the ratio of the electron & ion masses is of the order: (me /Mi ) ~ 10-3 ( << 1) Classically, the massive ions move much slower than the very small mass electrons! Typical ionic vibrational frequencies: υi ~ 1013 s -1 The time scale of the ion motion is: ti ~ 10-13 s .Electronic motion occurs at energies of about a bandgap:Eg= hυe = ħω ~ 1 eV υe ~ 1015 s -1 te ~ 10-15 s . So, The electrons respond to the ion motion ~ instantaneously! In the electron Hamiltonian, He , the ions can be treated as stationary!

In the ion Hamiltonian,Hi,the electrons can be treated in an average way! The Born-Oppenheimer(Adiabatic)Approximation Write the vibrating ion coordinatesas Ri=Rio+Ri Rjo=equilibrium ion positions,5R=deviation from the equilibrium positions The many body electron-ion Hamiltonian is(schematic!) He-i=He-i(ri,Rjo)+He-i(ri,6Ri) The New many body Hamiltonian in this approximation is: H=He(r)+H(ri,Rio)+H(R)+H-i (ri,5R)(1) (neglect the last 2 terms in band calculations)or H=HE [1st 2 terms of (1)]+HI [2nd 2 terms of (1)] HE=Electron Part(gives the energy bands),H=Ion Part(gives the phonons) For electronic properties(bands),this allows us to focus on the electronicpartof the many-body Hamiltonian:He=He(ri)+Hi(ri,Rjo) H(ri)=electron kinetic energy+electron-electron(Coulomb)repulsion H-i(ri,Rio)=electron(Coulomb)attractionto the stationary ions
The Born-Oppenheimer (Adiabatic) Approximation • Write the vibrating ion coordinates as Rj = Rjo + δRj , Rjo = equilibrium ion positions, δRj = deviation from the equilibrium positions • The many body electron-ion Hamiltonian is (schematic!): He-i ~ = He-i (ri ,Rjo) + He-i (ri ,δRj ) • The New many body Hamiltonian in this approximation is: H = He (ri ) + He-i (ri ,Rjo) + Hi (Rj ) + He-i (ri ,δRj ) (1) (neglect the last 2 terms in band calculations) or H= HE [1st 2 terms of (1)] + HI [2nd 2 terms of (1)] HE = Electron Part (gives the energy bands), HI = Ion Part (gives the phonons) In the ion Hamiltonian, Hi , the electrons can be treated in an average way! For electronic properties (bands), this allows us to focus on the electronic part of the many-body Hamiltonian:HE = He (ri ) + He-i (ri ,Rjo) He (ri ) = electron kinetic energy + electron-electron (Coulomb) repulsion He-i (ri ,Rjo) = electron (Coulomb) attraction to the stationary ions