
Chapter 7 The electronic theory of metal Objectives At the end of this Chapter,you should: 1.Understand the physical meaning of Fermi statistical distribution function. 2.Be able to calculate the Fermi level at 0 K. 3.Understand the concept of work function and contact potential. 4.Be able to calculate the heat capacity of electrons for metals
Chapter 7 The electronic theory of metal Objectives At the end of this Chapter, you should: 1. Understand the physical meaning of Fermi statistical distribution function. 2. Be able to calculate the Fermi level at 0 K. 3. Understand the concept of work function and contact potential. 4. Be able to calculate the heat capacity of electrons for metals

7.1 Fermi statistics and the heat capacity of electron The function of Fermi distribution For the equilibrium state of one system,the basic principle of Fermi statistics can be 1 f(E)=eF-+1 Which the probability that the eigenstate at energy E will be occupied by an electron
7.1 Fermi statistics and the heat capacity of electron For the equilibrium state of one system, the basic principle of Fermi statistics can be k 1 ( ) 1 E E T F B f E e − = + Which the probability that the eigenstate at energy E will be occupied by an electron. The function of Fermi distribution
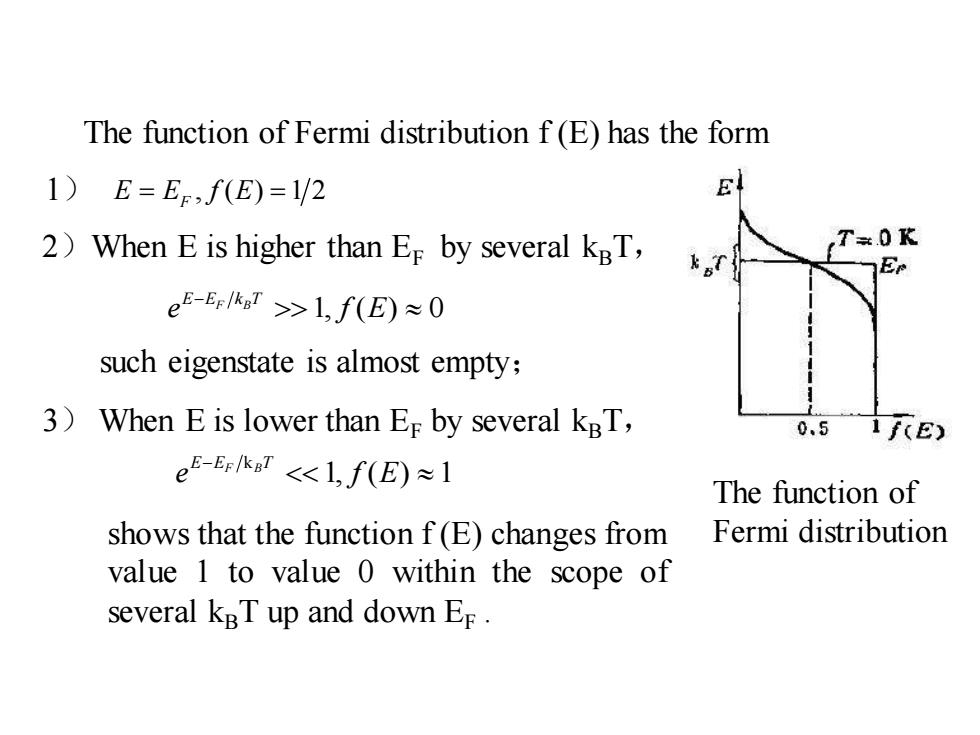
The function of Fermi distribution f(E)has the form 1)E=Ee,f(E)=1/2 E 2)When E is higher than Er by several kBT, T=0K e5-Ee/kr>1,f(E)≈0 such eigenstate is almost empty; 3)When E is lower than Er by several kpT, 0.5 1f(E) eB-Erkr<l,f(E)≈1 The function of shows that the function f(E)changes from Fermi distribution value 1 to value 0 within the scope of several kBT up and down Er
The function of Fermi distribution f (E) has the form , ( ) 1 2 E E f E = = F 1, ( ) 0 E E k T F B e f E − k 1, ( ) 1 E E T F B e f E − The function of shows that the function f (E) changes from Fermi distribution value 1 to value 0 within the scope of several kBT up and down EF . such eigenstate is almost empty; 3) When E is lower than EF by several kBT, 2)When E is higher than EF by several kBT, 1)
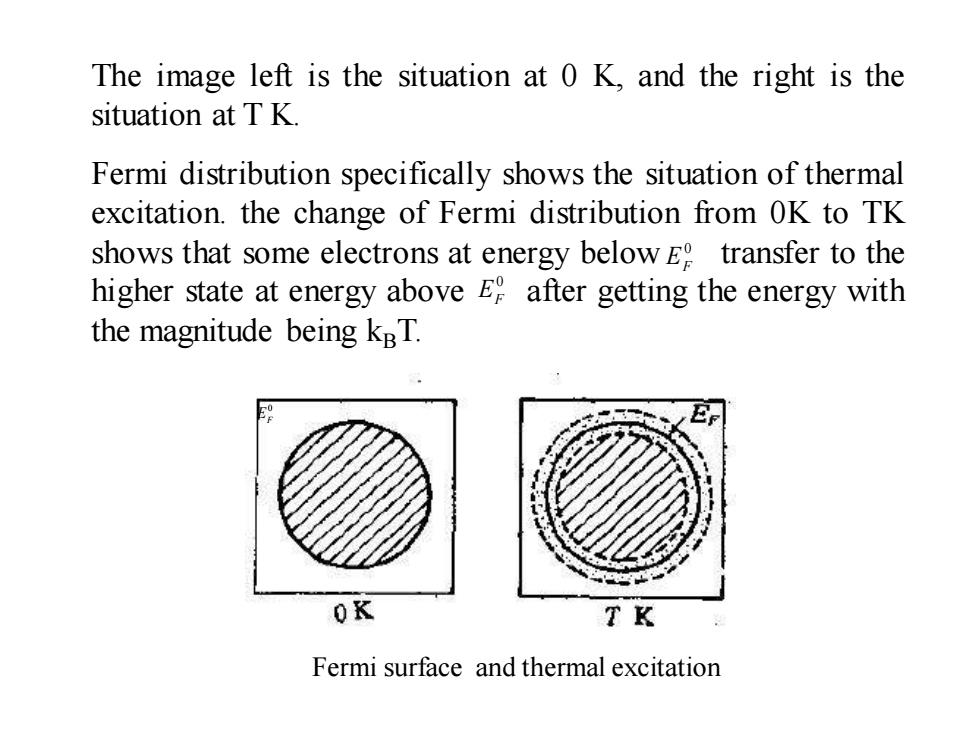
The image left is the situation at 0 K,and the right is the situation at TK. Fermi distribution specifically shows the situation of thermal excitation.the change of Fermi distribution from OK to TK shows that some electrons at energy below E transfer to the higher state at energy above E after getting the energy with the magnitude being kBT. 0K TK Fermi surface and thermal excitation
The image left is the situation at 0 K, and the right is the situation at T K. Fermi distribution specifically shows the situation of thermal excitation. the change of Fermi distribution from 0K to TK shows that some electrons at energy below transfer to the higher state at energy above after getting the energy with the magnitude being kBT. Fermi surface and thermal excitation 0 EF 0 EF 0 EF
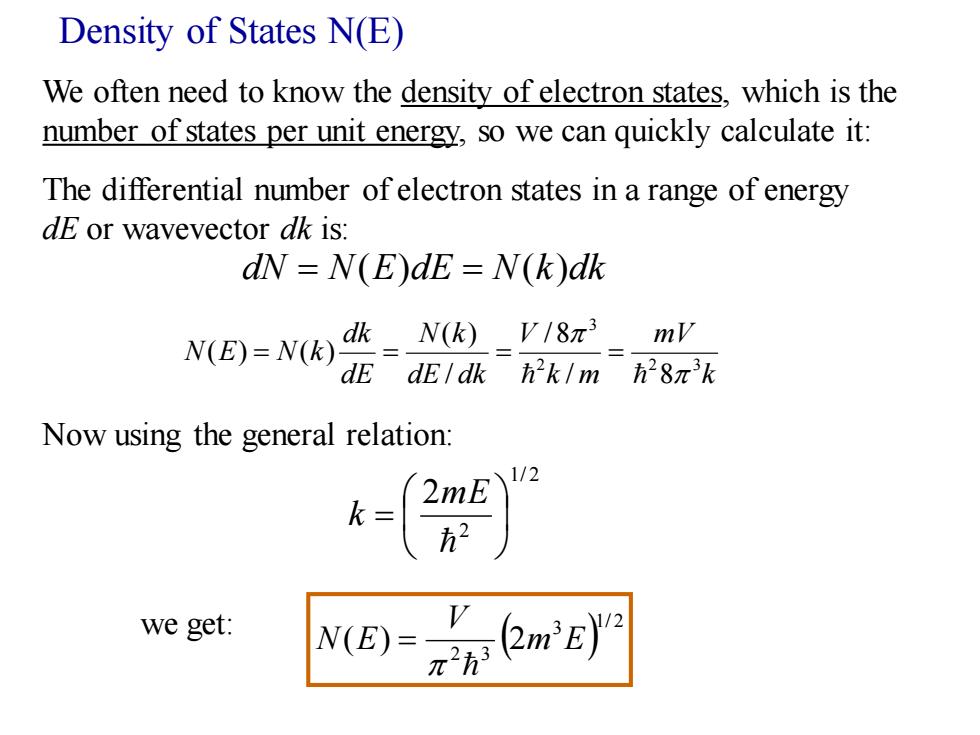
Density of States N(E) We often need to know the density of electron states,which is the number of states per unit energy,so we can quickly calculate it: The differential number of electron states in a range of energy dE or wavevector dk is: dN N(EdE=N(k)dk N(E)=N(k d_N(k)_V/8π3mV dEdE/dk2k/m28π3k Now using the general relation: 1/2 k=司 2mE we get: C2mE)
Density of States N(E) We often need to know the density of electron states, which is the number of states per unit energy, so we can quickly calculate it: dN = N(E)dE = N(k)dk k mV k m V dE dk N k dE dk N E N k 2 2 3 3 / 8 /8 / ( ) ( ) ( ) = = = = Now using the general relation: 1/ 2 2 2 = mE k we get: ( ) 1/ 2 3 2 3 ( ) 2m E V N E = The differential number of electron states in a range of energy dE or wavevector dk is: