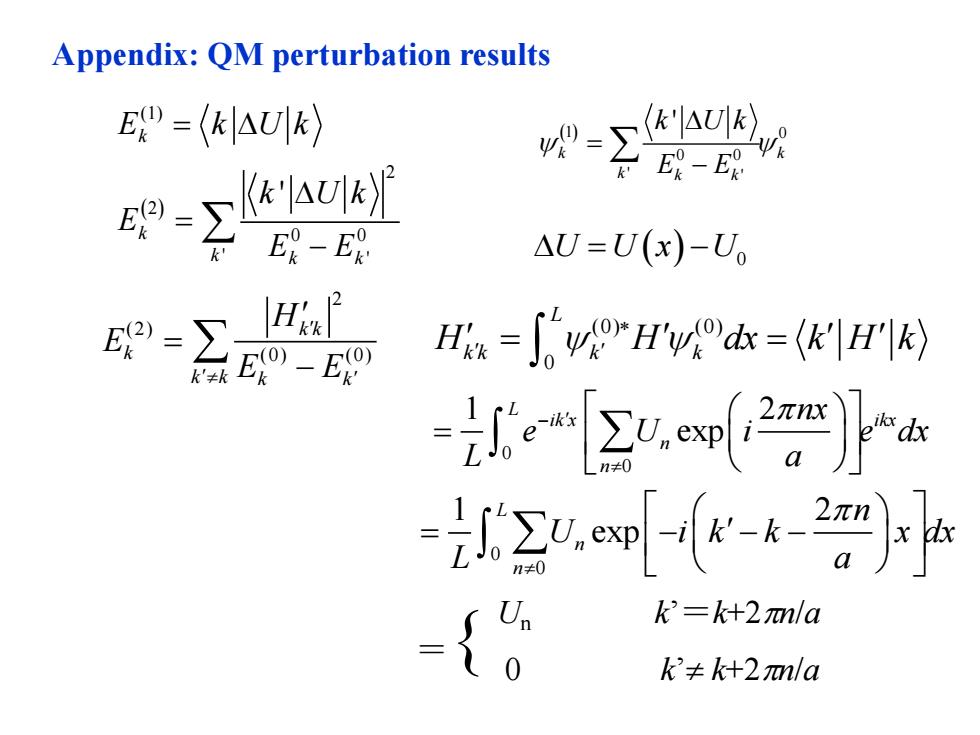
Appendix:QM perturbation results E0=(kAUk〉 - - 1E-E8 △U=U(x)-U。 Hg=∫o"Has=(k|Hk =e[Σ,m2aa v.cop- -{0 k'=k+2mla k'≠k+2mla
Appendix: QM perturbation results ( ) (1) 2 2 0 0 ' ' ' k k k k k E k U k k U k E E E = = − (1) 0 0 0 ' ' ' k k k k k k U k E E = − ( ) = − U U x U0 2 (2) (0) (0) k k k k k k k H E E E = − (0) (0) 0 L H H dx k H k k k k k = = 0 0 1 2 exp L ik x ikx n n nx e U i e dx L a − = 0 0 1 2 exp L n n n U i k k x dx L a = − − − ={ Un k’=k+2n/a 0 k’ k+2n/a
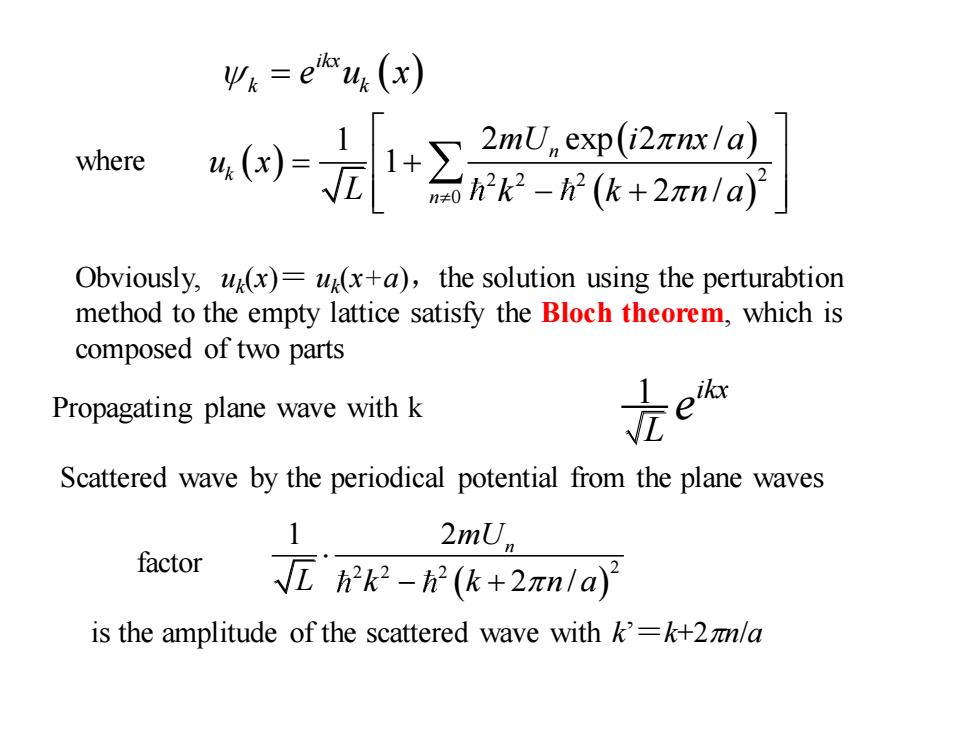
V:=eu (x) where +x器g Obviously,u(x)=u(x+a),the solution using the perturabtion method to the empty lattice satisfy the Bloch theorem,which is composed of two parts Propagating plane wave with k Scattered wave by the periodical potential from the plane waves 1 2mU factor √Dh2k2-(k+2πn/a)2 is the amplitude of the scattered wave with k'=k+2/a
( ) ikx k k = e u x where ( ) ( ) ( ) 2 2 2 2 0 1 2 exp 2 / 1 2 / n k n mU i nx a u x L k k n a = + − + Obviously, uk (x)= uk (x+a),the solution using the perturabtion method to the empty lattice satisfy the Bloch theorem, which is composed of two parts 1 ikx L Propagating plane wave with k e Scattered wave by the periodical potential from the plane waves factor ( ) 2 2 2 2 1 2 2 / mUn L k k n a − + is the amplitude of the scattered wave with k’=k+2n/a