
Perturbation method calculation:a 1D crystal L=Na 「-器e=vd U(x)=U(x+a) a is the lattice constant Fourier expansion: U(x)=U,+∑U,exp ,2πx a where U-U()dk average potential U =constant v.(es U minute term Potential is real term,not complex,so U(x)=U(x),UU
U x U x a ( ) = + ( ) a is the lattice constant Fourier expansion: ( ) 0 0 2 exp n n nx U x U U i a = + where ( ) 0 0 1 L U U x dx L = —— average potential =constant ( ) 0 1 2 exp L n nx U U x i dx L a = − L Na = Un minute term Potential is real term, not complex,so U(x)=U* (x), Un * =U-n 。 Perturbation method calculation: a 1D crystal ( ) ( ) ( ) 2 2 2 2 d U x x E x m dx − + = U

5.3.1.Nondegenerate perturbation H4=E(k)4H=- h2 d2 mdr+U(x) 品+-. 、 =H。+H n≠( H h2 d2 2m dx2 Zeroth order approximation r-22) Perturbationterm expand E(k)and v(k) E(k)=E0+E+E2+. 4=g0)+y0+y2)+
5.3.1. Nondegenerate perturbation ( ) H E k k k = ( ) 2 2 2 d 2 d H U x m x = − + 2 2 2 0 0 0 d 2 exp 2 d n n nx U U i H H m x a = − + + = + 2 2 0 0 2 d 2 d H U m x = − + Zeroth order approximation 0 2 exp n n nx H U i a = Perturbation term expand E(k) and (k) ( ) (0) (1) (2) E k E E E = + + + k k k (0) (1) (2) k k k k = + + +
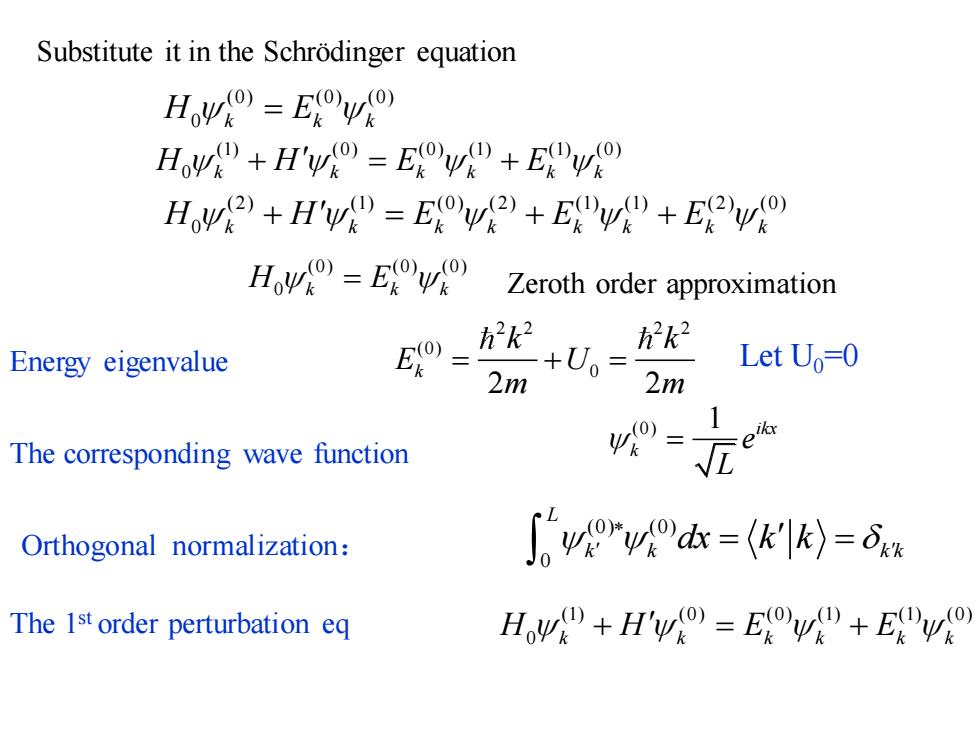
Substitute it in the Schrodinger equation Hw=E How+H'vEw+Eo How +Hv=EE+E HEZeroth order approximation 2k2 Energy eigenvalue Let Uo=0 2m = 1 The corresponding wave function e Orthogonal normalization: ∫yery9=k1k)=d The 1st order perturbation eq H"+Hy°=E0+Ey0
Substitute it in the Schrödinger equation (0) (0) (0) H E 0 k k k = (1) (0) (0) (1) (1) (0) H H E E 0 k k k k k k + = + (2) (1) (0) (2) (1) (1) (2) (0) H H E E E 0 k k k k k k k k + = + + Zeroth order approximation (0) (0) (0) H E 0 k k k = Energy eigenvalue 2 2 2 2 (0) 0 2 2 k k k E U m m = + = The corresponding wave function (0) 1 ikx k e L = Orthogonal normalization: (0) (0) 0 L k k k k dx k k = = The 1st order perturbation eq (1) (0) (0) (1) (1) (0) H H E E 0 k k k k k k + = + Let U0=0
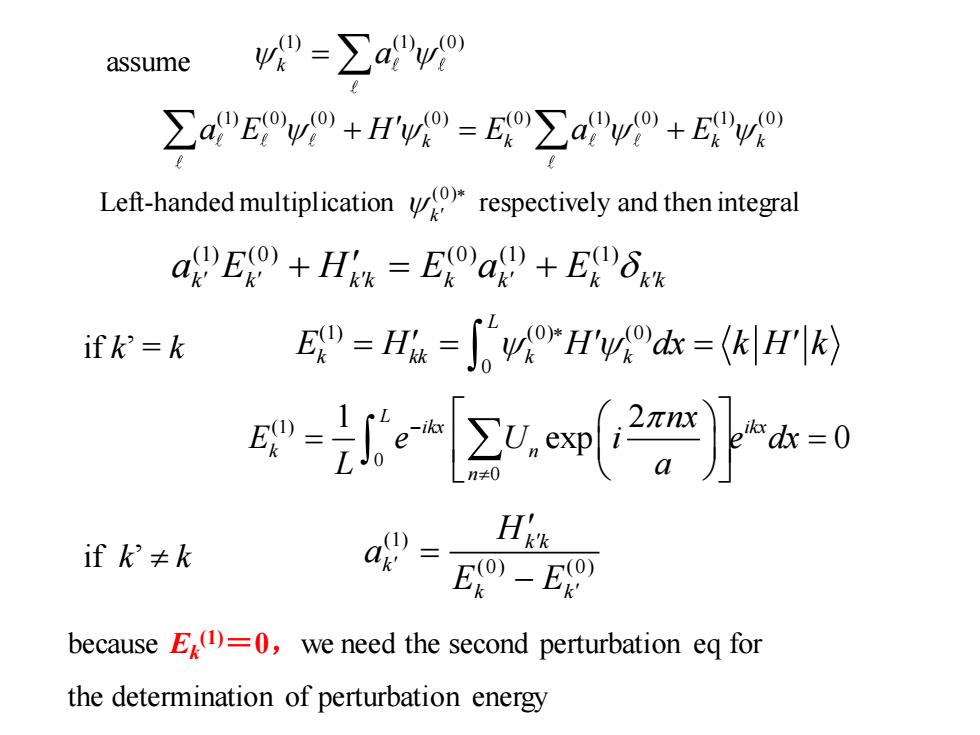
assume "-∑a"y9 ∑a"Ey0+Hy=E∑ap°+Ew° Lef-handed multiplicationrespectively and then integral aE0+Hk=Ega0+Eδ if k'=k E=H=∫*H'yk=kHlk) -e[Σ4,m2a产0 ifk≠k a E-E because E(1)=0,we need the second perturbation eq for the determination of perturbation energy
assume (1) (1) (0) k = a (1) (0) (0) (0) (0) (1) (0) (1) (0) k k k k a E H E a E + = + Left-handed multiplication respectively and then integral (0) k (1) (0) (0) (1) (1) k k k k k k k k k a E H E a E + = + if k’ = k (1) (0) (0) 0 L E H H dx k H k k kk k k = = = (1) 0 0 1 2 exp 0 L ikx ikx k n n nx E e U i e dx L a − = = if k’ k (1) (0) (0) k k k k k H a E E = − because Ek (1)=0,we need the second perturbation eq for the determination of perturbation energy

assume aSubstitute it into the second perturbation eq A=E”+-+a 2m E-E0 + 2mU, 2m 以1时+名” K'=k+2πn a 1得
assume (2) (2) (0) k = a Substitute it into the second perturbation eq 2 2 2 (0) (2) (0) (0) 2 k k k k k k k k k k H E E E m E E = + = + − 2 2 2 2 0 2 2 2 2 2 2 n n k m U m n k k a = + − + (0) (1) (0) (0) (0) (0) k k k k k k k k k k k H E E = + = + − ( ) ( ) 2 2 2 2 0 1 2 exp 2 / 1 2 / ikx n n mU i nx a e L k k n a = + − + 2 n k k a = +