
Examples,calculating (0)? m o(0) 0 0 V2 1 0 6 cos0 2 ±1 sin0 2 2 0 v10 3cos20-1) 4 士1 15 V2 sinecos0 ±2 15 V4 sin20
Examples, calculating ( )? l m 0 0 1 0 ± 1 2 0 ± 1 ± 2 2 2 sin 4 15 sin cos 2 15 ( 3cos 1 ) 4 10 sin 2 3 cos 2 6 2 1 ( )
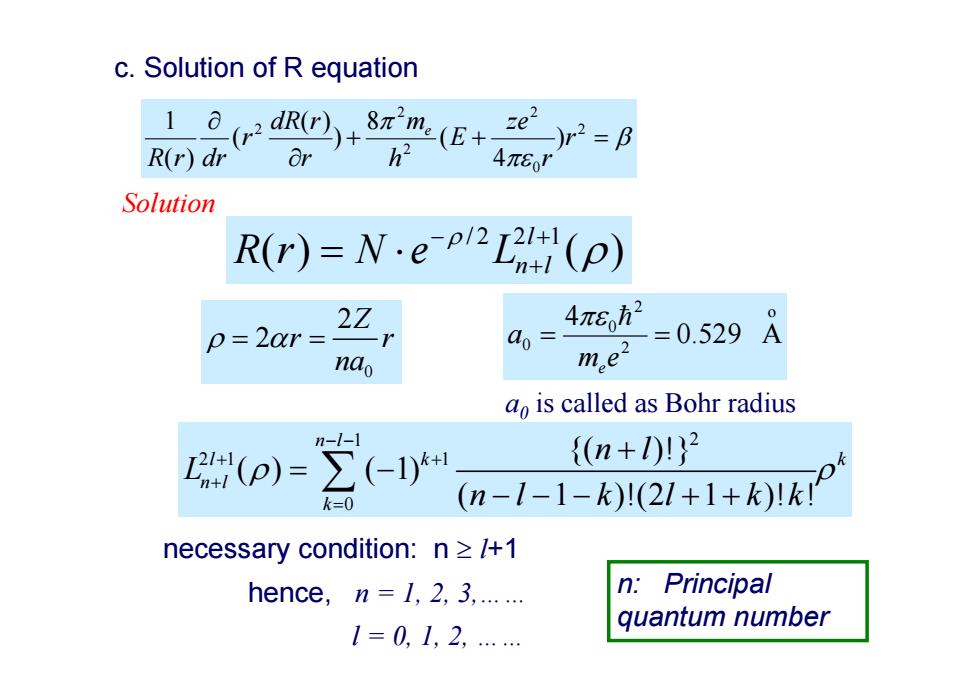
c.Solution of R equation (rdR(r)m(E+e)2=B R(r)dr or 4π8o1 Solution R(r)=N.e(p) 22 4πe p=20r= a0= =0.529A nao me2 a is called as Bohr radius n-1-1 {(n+1))2 k=0 (n-1-1-k)1(21+1+k)1kI necessary condition:n >/+1 hence,n=1,2,3,...... Principal 1=0,1,2,…… quantum number
c. Solution of R equation 2 0 2 2 2 2 ) 4 ( 8 ) ( ) ( ( ) 1 r r ze E h m r dR r r R r dr e necessary condition: n l+1 hence, n = 1, 2, 3,…… l = 0, 1, 2, …… Solution /2 2 1 () ( ) l Rr N e Ln l 0 2 2 Z r r na 1 2 21 1 0 {( )!} ( ) ( 1) ( 1 )!(2 1 )! ! n l lk k n l k n l L nl k l kk a 0 is called as Bohr radius o 2 2 0 0 0.529 A 4 m e a e n: Principal quantum number

Z2h2 h2 E=- =-R 8π2mna 8π2ma n R is called as Rydberg constant with the value of 13.6 eV Example: 3/2 Z n=1,=0 R0=2 e p12 a 3/2 n=2,1=0 Z R20 (2-p)ep/2 2V2 a
22 2 2 2 2 22 2 2 2 2 0 0 ( ) 8 8 Zh h Z Z E R mn a ma n n R is called as Rydberg constant with the value of 13.6 eV n=1, l=0 Example: 3/2 / 2 10 0 2 Z R e a n=2, l=0 / 2 3 / 2 0 20 ( 2 ) 2 2 1 e a Z R
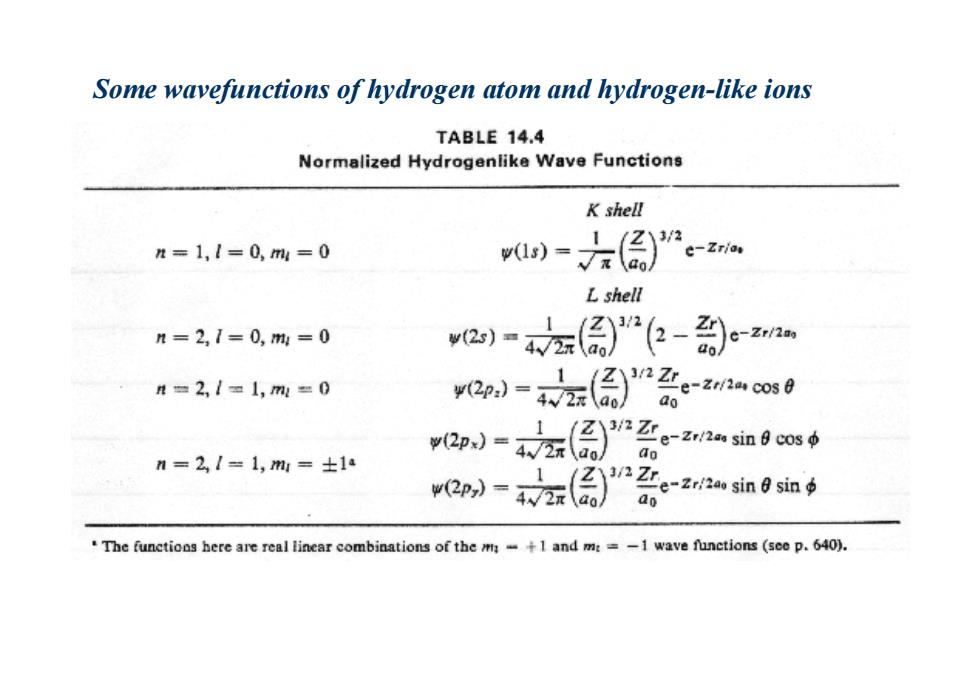
Some wavefunctions of hydrogen atom and hydrogen-like ions TABLE 14.4 Normalized Hydrogenlike Wave Functions K shell n=1,1=0,m=0 )- "e-Zrias Lshell n=2,1=0,m=0 2)"左(偏-- n=2,1=1,m=0 o-寸z原)品e-ano0 ao n=2,1=1,m=士14 2p小-左()系cn如9oms6 ap=左(原名e-血0n The functions here are real linear combinations of the m+1 and m:=-1 wave functions (see p.640)
Some wavefunctions of hydrogen atom and hydrogen-like ions

2.2 The physical significance of quantum numbers
2.2 The physical significance of quantum numbers