
b.⊙o)equation sinθa ©(0)a0 (sine (+Bsin=m a0 When B=1(/+1),=0,1,2,3,......(0)is a well behaved function, 1:angular momentum quantum number necessary condition:>m hence,l=0,1,2,3…s,p,dfg,h…) m=0.1,0n1,2,-1,0,1,2).… Examples of⊙(o): 1 m Θ(8) 0 0 店 1 0 6 2 cos0 ±1 -sin0 2 2 0 v1 4 (3c0s20-1) ±1 15 sinθcos0 ±2 15 V4 sin20
b. Θ(θ) equation When β=l(l+1), l =0,1,2,3,…… Θ(θ) is a well behaved function, l : angular momentum quantum number necessary condition: l ≥ |m| hence, l = 0, 1, 2, 3,…(s,p,d,f,g,h…) m=0,(-1,0,1),(-2,-1,0,1,2)…… 2 2 ) sin ( ) (sin ( ) sin + = m ∂ ∂Θ ∂ ∂ Θ β θ θ θ θ θ θ θ Examples of Θ(θ): l m 0 0 1 0 ±1 2 0 ±1 ±2 Θ(θ ) θ2 sin 4 15 2 1 cosθ 2 6 sinθ 2 3 (3cos 1) 4 10 2 θ − sinθ cosθ 2 15
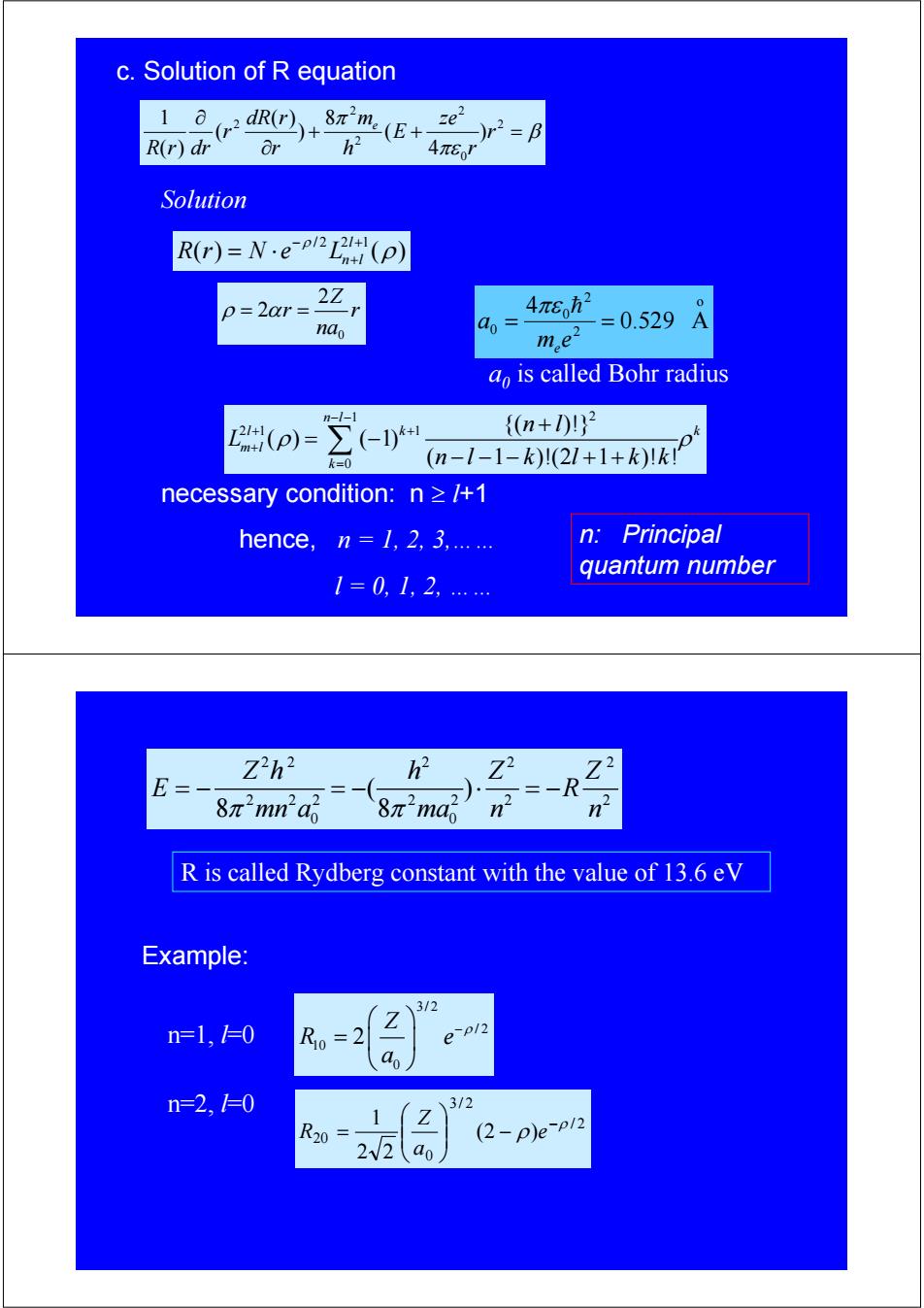
c.Solution of R equation 1 dR(r) 8πme(E+ e R(r)dr Or 3 )r2=B 4π6J Solution R(r)=N·ep2L2H n+1(p) 2Z p=2ar= 4πEh2 nao ao =0.529 me2 ao is called Bohr radius n--1 2(p)=-I) {n+10}2 k=0 (n-1-1-k)(21+1+k)Ik necessary condition:n /+1 hence,n 1,2,3,...... n: Principal quantum number 1=0,1,2 Zh2 E= 8元2na 8π2ma R is called Rydberg constant with the value of 13.6 eV Example: 3/2 n=1,=0 R0=2 e-pl2 do n=2,1=0 3/2 1 Z R20 (2-p)ep/2 2W2 ao
c. Solution of R equation β πε π + + = ∂ ∂ 2 0 2 2 2 2 ) 4 ( 8 ) ( ) ( ( ) 1 r r ze E h m r dR r r R r dr e Solution /2 2 1 () ( ) l Rr N e Ln l ρ ρ − + = ⋅ + 0 2 2 Z r r na ρ α = = 1 2 21 1 0 {( )!} ( ) ( 1) ( 1 )!(2 1 )! ! n l lk k m l k n l L nl k l kk ρ ρ − − + + + = + = − −−− ++ ∑ a0 is called Bohr radius o 2 2 0 0 0.529 A 4 = = m e a e πε h necessary condition: n ≥ l+1 hence, n = 1, 2, 3,…… l = 0, 1, 2, …… n: Principal quantum number 22 2 2 2 2 22 2 2 2 2 0 0 ( ) 8 8 Z h hZ Z E R π π mn a ma n n =− =− ⋅ =− R is called Rydberg constant with the value of 13.6 eV n=1, l=0 Example: 3/2 / 2 10 0 2 Z R e a −ρ ⎛ ⎞ = ⎜ ⎟ ⎝ ⎠ n=2, l=0 / 2 3/ 2 0 20 (2 ) 2 2 1 ρ ρ − − ⎟ ⎟ ⎠ ⎞ ⎜ ⎜ ⎝ ⎛ = e a Z R

Some wavefunctions of hydrogen atom and hydrogen-like ions TABLE 14.4 Normalized Hydrogenlike Wave Functions K shell n=1,1=0,m=0 )-图)。 )e-zian Lshell n=2,1=0,m=0 )-(”6- )c-mm n=2,1✉1,m=0 0-z原”系enos0 ao n=2,1=1,m=±1 yp=}ean如9m6 do o-左佰 e-Zr2a0 sinsin do The functions here are real linear combinations of the m+I and m:=-1 wave functions (see p.640). Ψ(,0,φ)=R)⊙(⊙)Φ() 2.2 The physical significance of quantum number
Some wavefunctions of hydrogen atom and hydrogen-like ions Ψ(r, θ, φ) =R(r)Θ(θ)Φ(φ) 2.2 The physical significance of quantum number

2.2.1 The allowed values of quantum numbers Quantum Numbers m 010+1010+1Λ -2-10+1+2 -2-10+1+2 -3-2-10+1+2+3 Problem:What values of the azimuthal (1)and magnetic (m)quantum numbers are allowed for a principal quantum number(n)of 4?How many orbitals are allowed for n=4? Solution:The 1 values go from 0 to (n-1),and for n=3 they are: 1 =0,1,2,3.The values for m go from-1 to zero to +l For1=0,m1=0 1=1,m=-1,0,+1 1=2,m1=-2,-1,0,+1,+2 1=3,m1=-3,-2,-1,0,+1,+2,+3 There are 16 mr values,so there are 16 orbitals for n=4! The total number of orbitals for a given value of n is n2
Quantum Numbers n l ml 1 2 3 4 0 0 1 0 1 2 0 1 2 3 0 0 -1 0 +1 0 -1 0 +1 0 -1 0 +1 -2 -1 0 +1 +2 -2 -1 0 +1 +2 -3 -2 -1 0 +1 +2 +3 2.2.1 The allowed values of quantum numbers Problem: What values of the azimuthal (l) and magnetic (m) quantum numbers are allowed for a principal quantum number (n) of 4? How many orbitals are allowed for n=4? Solution: The l values go from 0 to (n-1), and for n=3 they are: l = 0,1,2,3. The values for m go from -l to zero to +l For l = 0, ml = 0 l = 1, ml = -1, 0, +1 l = 2, ml = -2, -1, 0, +1, +2 l = 3, ml = -3, -2, -1, 0, +1, +2, +3 There are 16 mL values, so there are 16 orbitals for n=4! • The total number of orbitals for a given value of n is n2

2.2.2 The principal quantum number,n .Also called the"energy"quantum number,indicates the approximate distance from the nucleus. Denotes the electron energy shells around the atom,and is derived directly from the Schrodinger equation. 。The higher the value of“n”,the greater the energy of the orbital. Positive integer values of n=1,2,3,etc. Example:Energy states of a H atom. 1 n principal En=-13.6 En -R n=1,2,3..∞ n n=1 ground state R=13.6eV E1=-13.6eV n 2 first excited state n 3 second excited state E2=-3.40eV Principle Quantum Energy. E3=-1.51eV Numher Continuum ev 0 n=5 -0.54 n=4 VVYV业 -0.85 E4=-0.85eV n=3- tvvve Brackett Paschen series -1.51 series n=2 YY业业 -3.39 E5=-0.54eV Balmer senes A=950A A=973A A=1026A A-1216A 业业业业" Convergence limit n"1 -13.6 Lyman series E。=0eV
2.2.2 The principal quantum number, n •Also called the “energy “ quantum number, indicates the approximate distance from the nucleus . • Denotes the electron energy shells around the atom, and is derived directly from the Schrodinger equation. • The higher the value of “n” , the greater the energy of the orbital. • Positive integer values of n = 1 , 2 , 3 , etc. n = principal n = 1, 2, 3 …∞ n = 1 : ground state n = 2 : first excited state n = 3 : second excited state En n = − ⎛ ⎝ ⎜ ⎜ ⎞ ⎠ ⎟ ⎟ 13 6 1 2 . E1 = -13.6 eV E2 = -3.40 eV E3 = -1.51 eV E4 = -0.85 eV E5 = -0.54 eV • • • E∞ = 0 eV Example: Energy states of a H atom. 2 n 2 Z E R n = − R = 13.6 eV