
seqular equation made equation resolved(c,c2≠O) Hu-ES Hv-ES2 =0 H21-ES21 H22-ES22 get E→get C1,c2→8et 中 The algebraic equation has 2 roots,E and E2. p=C+C2Ψ2++CnΨn Hu-ES H1-ES12 .. Hyn-ESin H21-ES21 H2-ES22 H2n-ES2n =0 Hn-ESn Hn2-ES2 Hm-ESom The algebraic equation has n roots,which can be shown to be real Arranging these roots in order of increasing value:E,≤E2≤..≤En From the variation theorem,we know that the lowest value of root (W)is the upper bound for the system's ground-state energy. E<W .Moreover,it can be proved that the linear variation method provides upper bounds to the energies of the lowest n states of the system. E2sW2E≤W3.…,En≤Wm We use the roots as approximations to the energies of the lowest states. If approximation to the energies of more states are wanted,we add more functions to the trial functionΦ,. The addition of more functions can be shown to increase the accuracy of the previously calculated energies
get E get c c get φ H ES H ES H ES H ES made equation resolved c c seqular equation ⇒ ⇒ = − − − − ≠ 1 2 21 21 22 22 11 11 12 12 1 2 , 0 ( , 0) 0 ... . . ... . . . ... . ... ... ... 1 1 2 2 21 21 22 22 2 2 11 11 12 12 1 1 1 1 2 2 = − − − − − − − − − = + + + n n n n nn nn n n n n n n H ES H ES H ES H ES H ES H ES H ES H ES H ES φ cψ c ψ c ψ The algebraic equation has n roots, which can be shown to be real. Arranging these roots in order of increasing value: E1≤ E2≤… ≤En. The algebraic equation has 2 roots, E1 and E2. • From the variation theorem, we know that the lowest value of root (W1) is the upper bound for the system’s ground-state energy. E1≤W1 • We use the roots as approximations to the energies of the lowest states. • If approximation to the energies of more states are wanted, we add more functions fk to the trial function φ. • The addition of more functions fk can be shown to increase the accuracy of the previously calculated energies. E2≤ W2, E3≤ W3, … , En ≤ Wn, •Moreover, it can be proved that the linear variation method provides upper bounds to the energies of the lowest n states of the system

3.The solution of ForH达that is a e-Ta B 1S4= =Ψa R Wπ e-n =Ψb Vπ Note:we have as many linear combinations as we have atomic orbitals φ=CaΨa+CbΨ6 Trial functions =CaΨa+CbΨb seqular equation Hoa-ESaa Hab-ESab =0 Hpa-ESpa Hob-ESbb has the same form as ∴Ha=Ho, Hab=Ha (Ha-ESa)2=(Hb-ESb)月 Haa-ESaa=(Hab-ESab) 计 Ha-ESa=-(Hab-ESab) E= Haa+Hab_a+B 1+Sab 1+S Haa-ESoa=Hab-ESab E2 Hoe-Hab= -B 1-Sab 1-S
3. The solution of H2 + A B e- r r b a R θ For H2 + that is : Note : we have as many linear combinations as we have atomic orbitals a r A a e s ψ π = = − 1 b 1 ψ π = = − br B e s a a b b φ = c ψ + c ψ Trial functions ( ) ( ) ( ) has the same form as 2 2 , aa aa ab ab aa aa ab ab a b aa bb ab ba H ES H ES H ES H ES H H H H − = ± − − = − Qψ ψ ∴ = = = 0 − − − − = + ba ba bb bb aa aa ab ab a a b b H ES H ES H ES H ES seqular equation φ c ψ c ψ S S H H E if H ES H ES S S H H E if H ES H ES ab aa ab aa aa ab ab ab aa ab aa aa ab ab − − = − − = − = − + + = + + = − = − − 1 1 1 1 ( ) 2 1 α β α β

substituting E to seqular equation (Haa-ESaa)Ca+(Hab-ESab)Cb=O () (Hba-ESba)Ca+(Hob-ESBb)Co=O (2) Soa =Sbb=1 aa h。- HaatHab )c,+(HHga+Hab Sab )c 1+Sab 1+Sab (Haa(1+Sab)-(Haa+Hab))Ca+(Hab(1+Sab)-(Haa+Hab)Sab)Cp=O (Hoa(I+Sab)-(Moa+Hab))ca+(Hab(1+Sab)-(Hoa+Mab)Sab)Co=O (HaaSab-Hab)Ca+(Hab-Haa Sab)Cp=O Ca-Ch=0 Ca=Cb 4=ca。+C6。=Ca(。+6) nomalization condition ∫4g=1 (c(y。+ys)'ca(y。+wb)dx=1 Ic Wa+2c WaVs+cWs]dt=1 2c。2(1+Sb)=1 1 Ca= V21+S6) 1 (y。+6) V2(1+Sb) substituting E2 to seqular equation C。+C6=0 Ca=-Cb 2=CΨ。+CbΨ。=C(y。-Ψ6) 1 02= (ya-Ψ) V2(1-Sb)
( ) ( ) 0 (2) ( ) ( ) 0 (1) 1 − + − = − + − = ba ba a bb bb b aa aa a ab ab b H ES c H ES c H ES c H ES c substituting E to seqular equation ( ) 0 ( ) ( ) 0 ( (1 ) ( )) ( (1 ) ( ) ) 0 ( (1 ) ( )) ( (1 ) ( ) ) 0 ) 0 1 ) ( 1 ( 1 1 a a b b a a b a b a b aa ab ab a ab aa ab b aa ab aa ab a ab ab aa ab ab b aa ab aa ab a ab ab aa ab ab b ab b ab aa ab a ab ab aa ab aa aa bb c c c c c c c H S H c H H S c H S H H c H S H H S c H S H H c H S H H S c S c S H H c H S H H H S S φ = ψ + ψ = ψ +ψ − = = − + − = / + − / + + + / − + / = + − + + + − + = = + + + − + + − = = ( ) 2(1 ) 1 2(1 ) 1 2 (1 ) 1 [ 2 ] 1 ( ( )) ( ) 1 1 1 2 2 2 2 2 2 * 1 * 1 a b ab ab a a ab a a a a b a b a a b a a b S S c c S c c c d c c d nomalization condition φ ψ ψ ψ ψ ψ ψ τ ψ ψ ψ ψ τ φ φ + + = + ∴ = + = + + = + + = = ∫ ∫ ∫ ( ) 2(1 ) 1 ( ) 0 2 2 2 a b ab a a b b a a b a b a b S c c c c c c c substituting E to seqular equation φ ψ ψ φ ψ ψ ψ ψ − − = = + = − + = = −
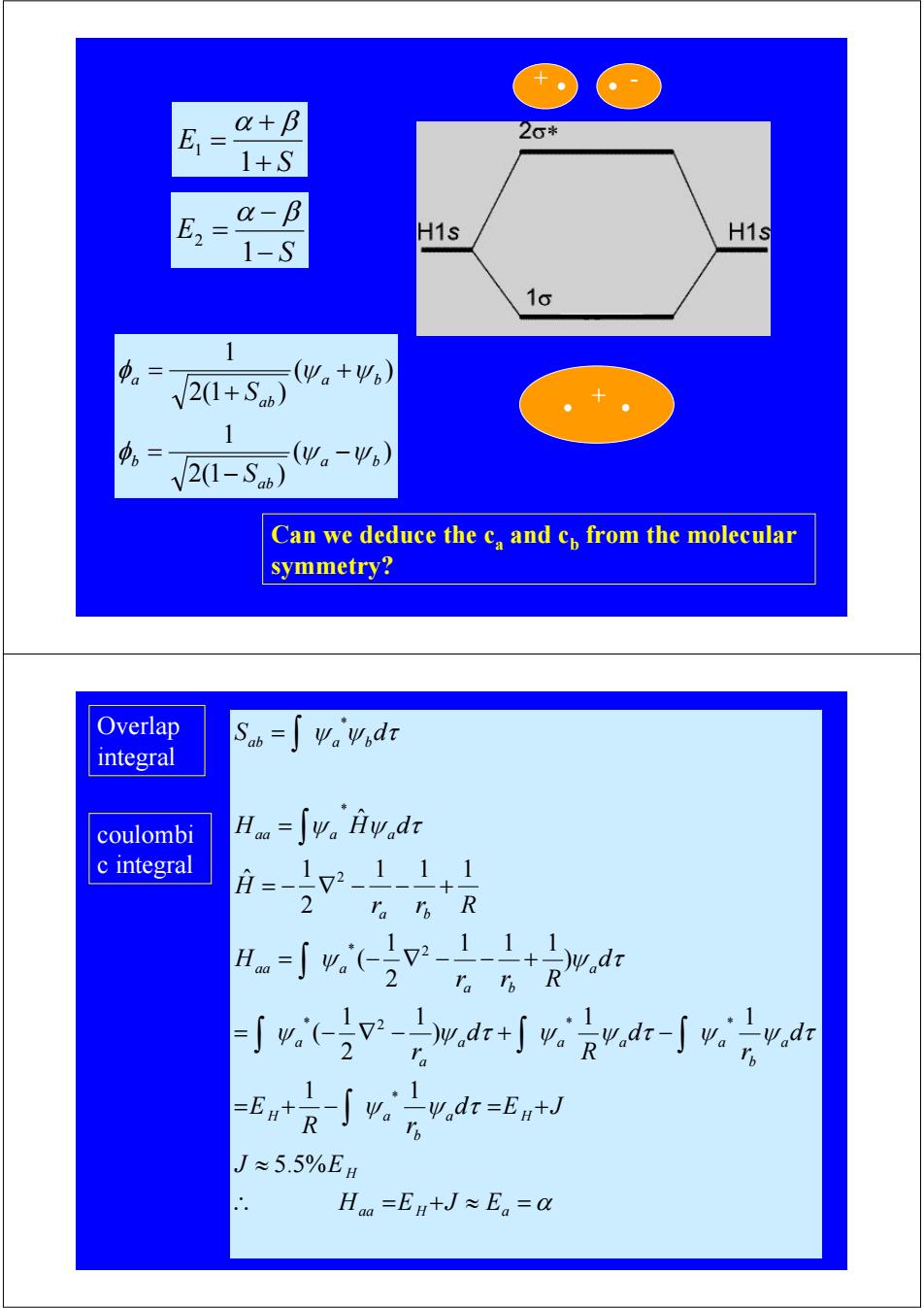
E a+B 26* 1+S E2= Q-B H1s H1s 1-S 16 1 V2(1+Sb) (Ψ。+Ψ6) 1 V2(1-Sb) (Ψ。-Ψ6) Can we deduce the c and cp from the molecular symmetry? Overlap Sab= Wa widr integral coulombi H=∫g,iwd c integral =-1v2-11+1 2 ra R _1-1+2w.dr w,ar+0d-可v J≈5.5%EH Haa=EH+J≈En=a
S E + + = 1 1 α β S E − − = 1 2 α β ( ) 2(1 ) 1 ( ) 2(1 ) 1 a b ab b a b ab a S S φ ψ ψ φ ψ ψ − − = + + = + + - Can we deduce the ca and cb from the molecular symmetry? Overlap integral α ψ ψ τ ψ ψ τ ψ ψ τ ψ ψ τ ψ ψ τ ψ ψ τ ψ ψ τ ∴ = + ≈ = ≈ = + − = + = − ∇ − + − = − ∇ − − + = − ∇ − − + = = ∫ ∫ ∫ ∫ ∫ ∫ ∫ aa H a H a H b H a a b a a a a a a a a b aa a a b aa a a ab a b H E J E J E d E J R r E d r d R d r d r r R H r r R H H H d S d 5.5% 1 1 1 1 ) 1 2 1 ( ) 1 1 1 2 1 ( 1 1 1 2 1 ˆ ˆ * * 2 * * * 2 2 * * coulombi c integral