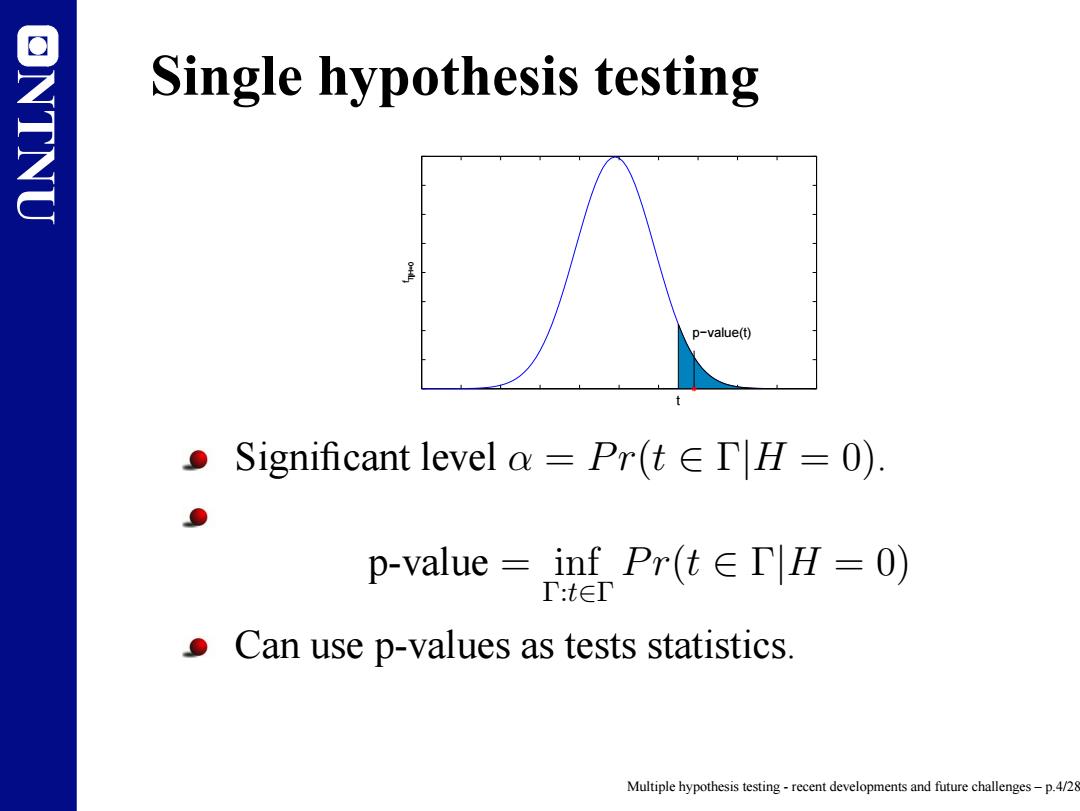
ONTNU Single hypothesis testing p-value(t) Significant level a=Pr(t∈TlH=O) p-value inf Pr(t EIH=0) T:t∈I Can use p-values as tests statistics. Multiple hypothesis testing-recent developments and future challenges-p.4/28
NTNU Single hypothesis testing f T|H=0 p−value(t) t Significant level α = Pr(t ∈ Γ|H = 0). p-value = inf Γ:t∈Γ Pr(t ∈ Γ|H = 0) Can use p-values as tests statistics. Multiple hypothesis testing - recent developments and future challenges – p.4/28
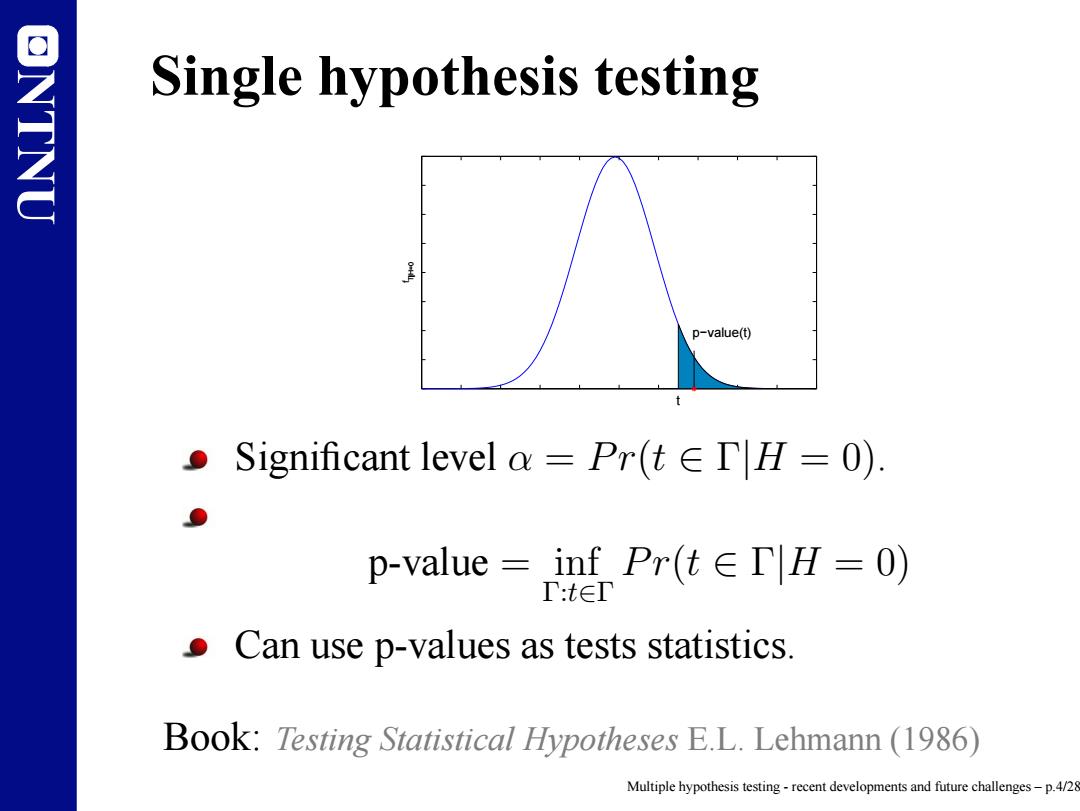
ONTNU Single hypothesis testing p-value(t) Significant level a=Pr(t∈TlH=O) p-value inf Pr(t EIH=0) T:t∈T Can use p-values as tests statistics. Book:Testing Statistical Hypotheses E.L.Lehmann(1986) Multiple hypothesis testing-recent developments and future challenges-p.4/28
NTNU Single hypothesis testing f T|H=0 p−value(t) t Significant level α = Pr(t ∈ Γ|H = 0). p-value = inf Γ:t∈Γ Pr(t ∈ Γ|H = 0) Can use p-values as tests statistics. Book: Testing Statistical Hypotheses E.L. Lehmann (1986) Multiple hypothesis testing - recent developments and future challenges – p.4/28

ONTNU Multiple hypothesis testing m hypothesis tests H1=0 versus H1=1 H2=0 versus H2 =1 0 0 。Hm=0 versus Hm=1 Want to make simultaneous inference. Rejection regions? Multiple hypothesis testing-recent developments and future challenges-p.5/28
NTNU Multiple hypothesis testing m hypothesis tests H1 = 0 versus H1 = 1 H2 = 0 versus H2 = 1 Hm = 0 versus Hm = 1 Want to make simultaneous inference. Rejection regions? Multiple hypothesis testing - recent developments and future challenges – p.5/28
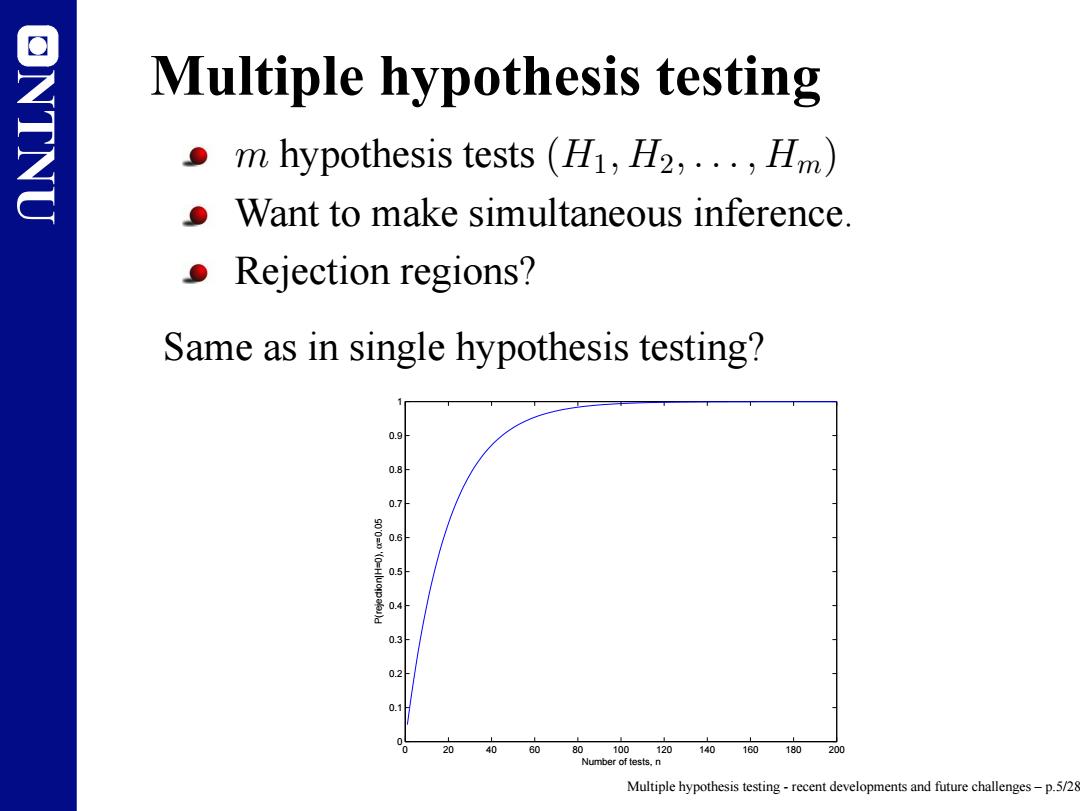
ONTNU Multiple hypothesis testing m hypothesis tests (H,H2,...,Hm) Want to make simultaneous inference. Rejection regions? Same as in single hypothesis testing? 0.9 0.2 0.1 60 80100 120140160180200 Number of tests.n Multiple hypothesis testing-recent developments and future challenges-p.5/28
NTNU Multiple hypothesis testing m hypothesis tests (H1, H2, . . . , Hm) Want to make simultaneous inference. Rejection regions? Same as in single hypothesis testing? 0 20 40 60 80 100 120 140 160 180 200 0 0.1 0.2 0.3 0.4 0.5 0.6 0.7 0.8 0.9 1 Number of tests, n P(rejection|H=0), α=0.05 Multiple hypothesis testing - recent developments and future challenges – p.5/28
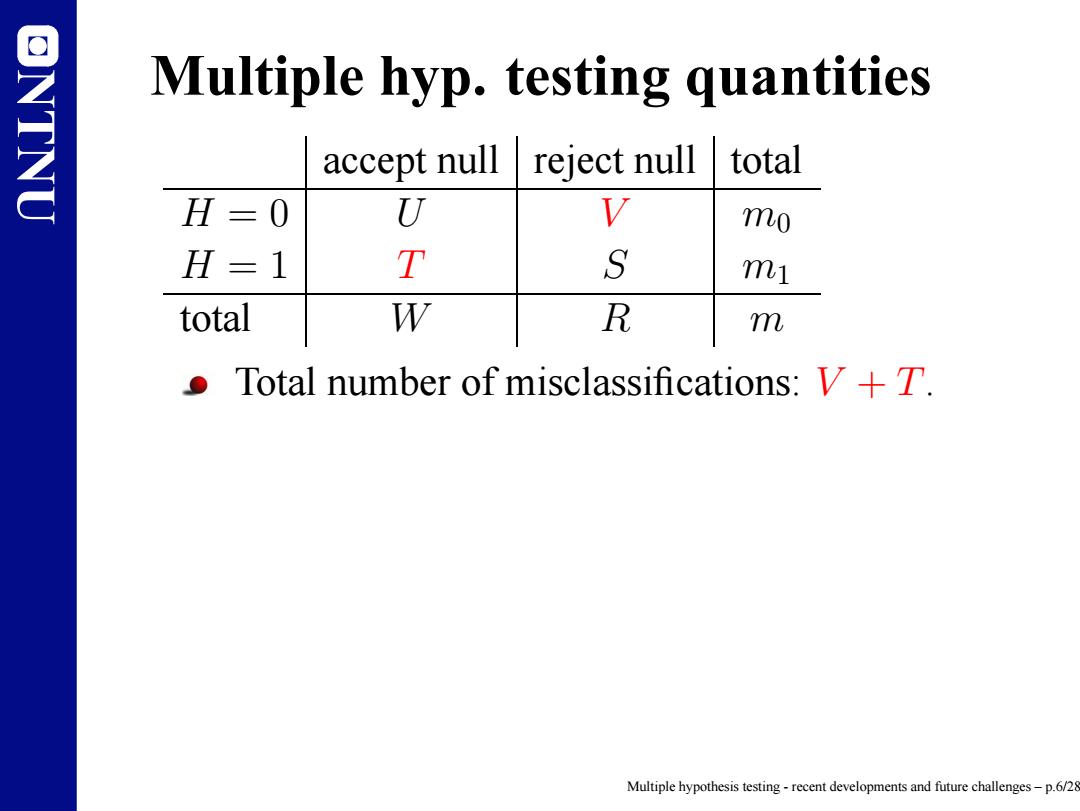
ONTNU Multiple hyp.testing quantities accept null reject null total H=0 U V mo H=1 T S mi total W R m Total number of misclassifications:V+T Multiple hypothesis testing-recent developments and future challenges-p.6/28
NTNU Multiple hyp. testing quantities accept null reject null total H = 0 U V m0 H = 1 T S m1 total W R m Total number of misclassifications: V + T. Multiple hypothesis testing - recent developments and future challenges – p.6/28