Introduction oTwo sample problem definitions Parametric solution Non parametric solution: permutation test randomization test bootstrap test R.Dahyot (TCD) 453 Modern statistical methods 20056/22
Introduction Two sample problem : definitions Parametric solution Non parametric solution: I permutation test I randomization test I bootstrap test R. Dahyot (TCD) 453 Modern statistical methods 2005 6 / 22
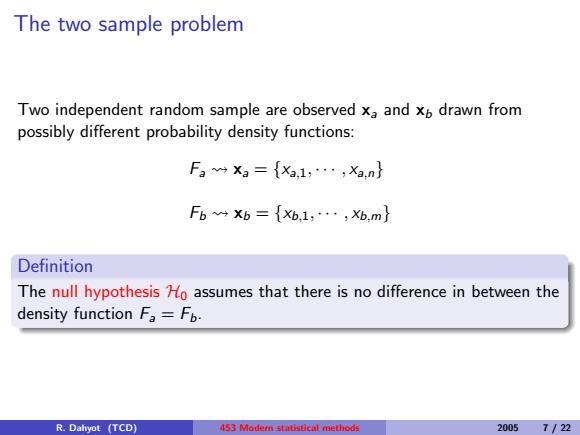
The two sample problem Two independent random sample are observed xa and xb drawn from possibly different probability density functions: Fgxa={x,1,…,X,n} Fbxb={xb,1,…,b,m} Definition The null hypothesis Hto assumes that there is no difference in between the density function Fa=Fb. R.Dahyot (TCD) 453 Moder statistical methods 20057/22
The two sample problem Two independent random sample are observed xa and xb drawn from possibly different probability density functions: Fa xa = {xa,1, · · · , xa,n} Fb xb = {xb,1, · · · , xb,m} Definition The null hypothesis H0 assumes that there is no difference in between the density function Fa = Fb. R. Dahyot (TCD) 453 Modern statistical methods 2005 7 / 22

Hypothesis test and Achieved significance level (ASL) Definition A hypothesis test is a way of deciding whether or not the data decisively reject the hypothesis Ho. Definition The achieved significance level of the test (ASL)is defined as: AsL=P(0*≥Ho) =6P(1to)d0 The smaller ASL,the stronger is the evidence of Ho false.The notation star differentiates between an hypothetical value generated according to Ho,and the actual observation 0. R.Dahyot (TCD)】 453 Modem statistical methods 2005 8/22
Hypothesis test and Achieved significance level (ASL) Definition A hypothesis test is a way of deciding whether or not the data decisively reject the hypothesis H0. Definition The achieved significance level of the test (ASL) is defined as: ASL = P(θ ˆ∗ ≥ θ ˆ|H0) = R +∞ θˆ P(θˆ∗ |H0) dθˆ∗ The smaller ASL, the stronger is the evidence of H0 false. The notation star differentiates between an hypothetical value θˆ∗ generated according to H0, and the actual observation θˆ. R. Dahyot (TCD) 453 Modern statistical methods 2005 8 / 22