上海交通大学：《Design and Manufacturing》课程教学资源（讲义）Lecture 6 Vector fundamentals for kinematic analysis

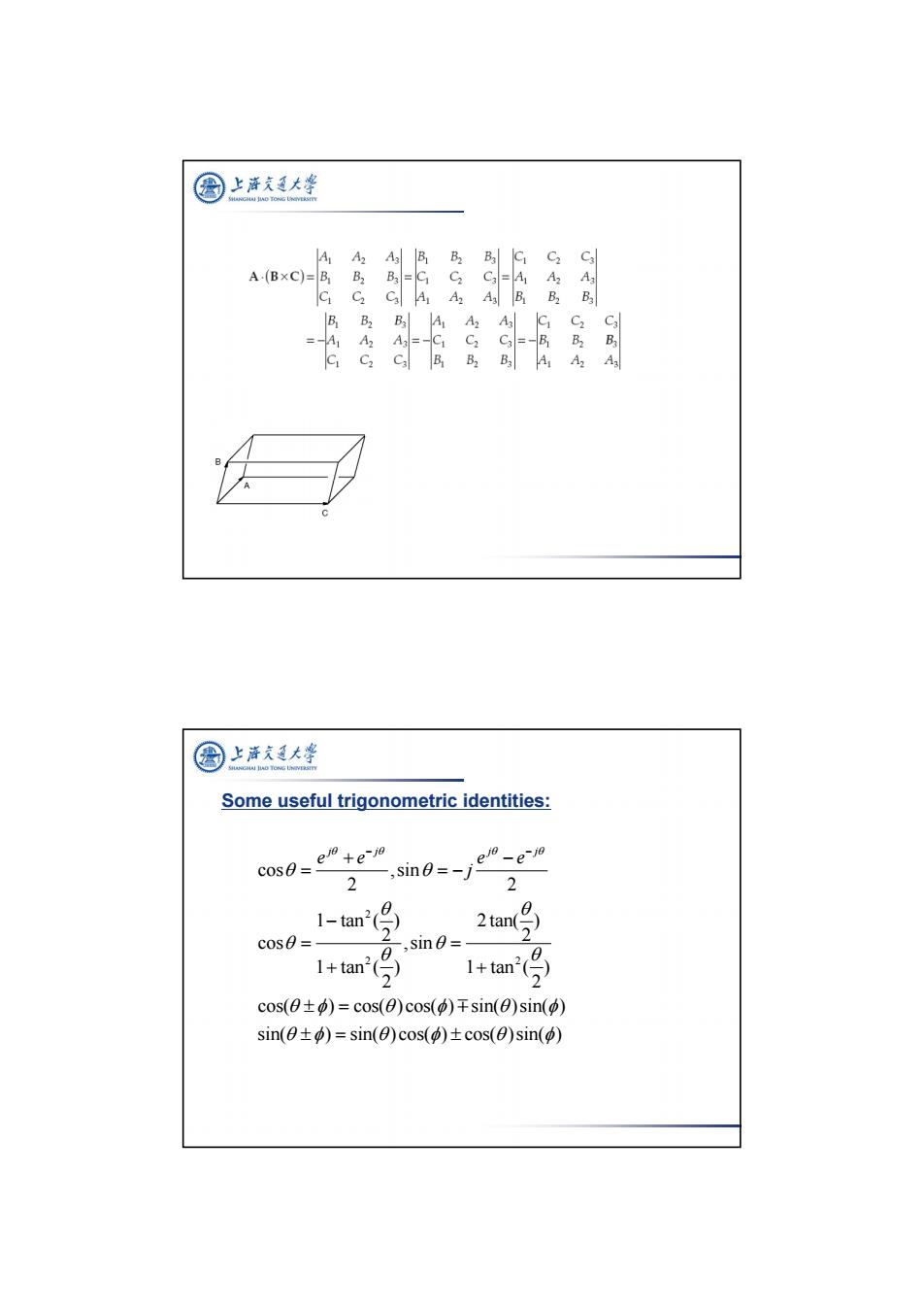
国上活我大峰 4 A(B×C)= 马G U 马G 5 ÷ G知肠 B B2 B o C 小0民 刘口朗 国 上海文通大学 Some useful trigonometric identities: cos0= -,sin=-j lo-e-n 2 2 cos0 1 sin 2a 1+a9 1*a9 cos(0±p)=cos(0)cos(p)干sin(0)sin(p) sin(e±)=sin(e)cos()±cos(g)sin()

Some useful trigonometric identities: 2 2 2 cos ,sin 2 2 1 tan ( ) 2 tan( ) 2 2 cos ,sin 1 tan ( ) 1 tan ( ) 2 2 cos( ) cos( )cos( ) sin( )sin( ) sin( ) sin( )cos( ) cos( )sin( ) jj jj ee ee j                                    

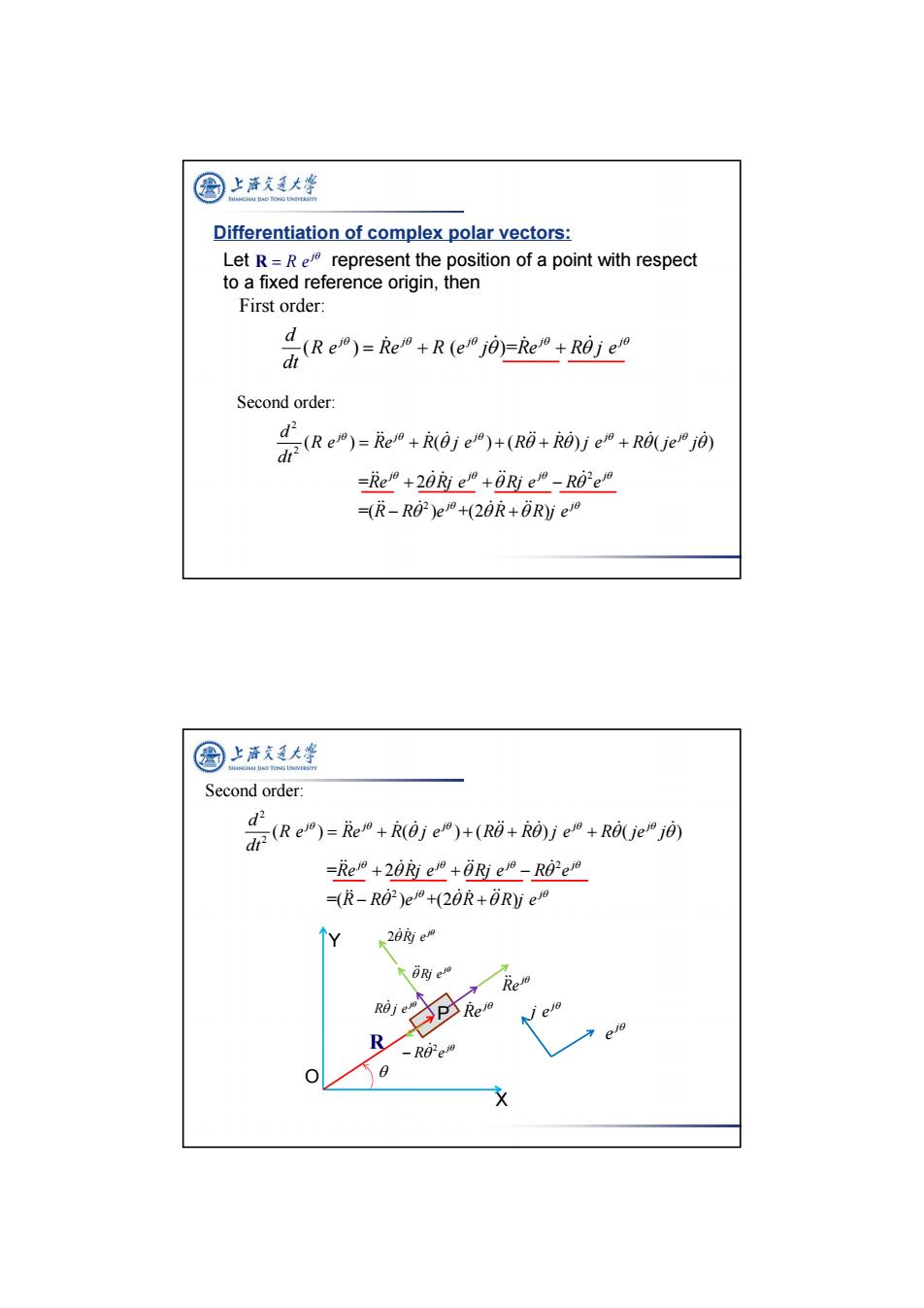
圆上清大峰 Differentiation of complex polar vectors: Let R=R e5 represent the position of a point with respect to a fixed reference origin,then First order kee Second order: (Re）=e+a0je+(R6+djeP+RUe7间 d =Re°+20Re+8Re-R0e =(R-R0)e+(20R+iR/e 圆上泽文大学 Second order: R)e)(Roe) d -Re+20Rj el+Rj e-ROeo -(R-RO-)e+(20R+0R)i ei Y 20Rje Rje -ROeo

Differentiation of complex polar vectors: j R e  Let repres R  ent the position of a point with respect to a fixed reference origin, then First order: ( ) ( )= d j jj j j R e Re R e j Re R j e dt             2 2 2 2 Second order: ( ) ( ) ( ) ( ) = 2 =( ) +(2 ) jj j j j j j jj j d R e Re R j e R R j e R je j dt Re Rj e Rj e R e RR e R R                                           j j e  X Y O R  j j e j  Re   j R j e    j e  j Re   2 j R e     2 j Rj e     j Rj e    P 2 2 2 2 Second order: ( ) ( ) ( ) ( ) = 2 =( ) +(2 ) jj j j j j j jj j d R e Re R j e RR j e R je j dt Re Rj e Rj e R e RR e R R                                           j j e 