
INFORMATIONAL CASCADES 997 TABLE 1 SIGNAL PROBABILITIES Pr(X;=HV) Pr(Xi=L|V) V=1 1- V=0 1- Pi rejected (in which case even an H signal induces him to reject and thus creates a DowN cascade),or(3)one has adopted and the other rejected.In the last case,the third individual is in the same situation as the first individual:his expected value of adoption,based only on his predecessors'actions,is 2,and therefore his signal determines his choice.Should this come about,then a similar analysis shows that the fourth individual would be in the same situation as the second individual,the fifth as the third,and so forth. With this decision rule,we can derive the unconditional ex ante probabilities of an Up cascade,no cascade,or a DowN cascade after two individuals, 2p-p31-p+p 1-力+2 2 and 1-(中-p2)m2 1-(中-p2)n2 9 ,( -p)3 2 (1) after an even number of individuals n.1 Equation(1)shows that the closer p is to l,the later a cascade is likely to start.A reduction in p toward is equivalent to adding noise to the signal;at p=,the signal is uninformative.In other words,cascades tend to start sooner when individuals have more precise signals of the value of adoption.2 Moreover,according to(1),the probability of not being in a cascade falls exponentially with the number of individuals.Even for a very 11 After two individuals,no cascade occurs if there is one H and one L.This value can be calculated assuming V=1 (or 0).The occurrence of either HL or LH involves a coin flip,so the total probability is 'p(1-p)+p(1-p)=p(1 -p).For the other two values,it suffices to note that since these probabilities are not conditional on V,Pr(up)=Pr(DOwN)=[l -Pr(no cascade)].For the expressions in eq.(1),note that the probability of an Up cascade after four individuals is the probability of an up cascade after two individuals plus the probability of not being in a cascade after two individuals multiplied by the probability of an UP cascade after another two individuals. In contrast,the probability of not being in a cascade after four individuals is simply the probability of not being in a cascade after two individuals multiplied by the proba- bility of not being in a cascade after another two individuals. 1Specifically,higher-precision p raises the probability of histories that lead to the correct cascade
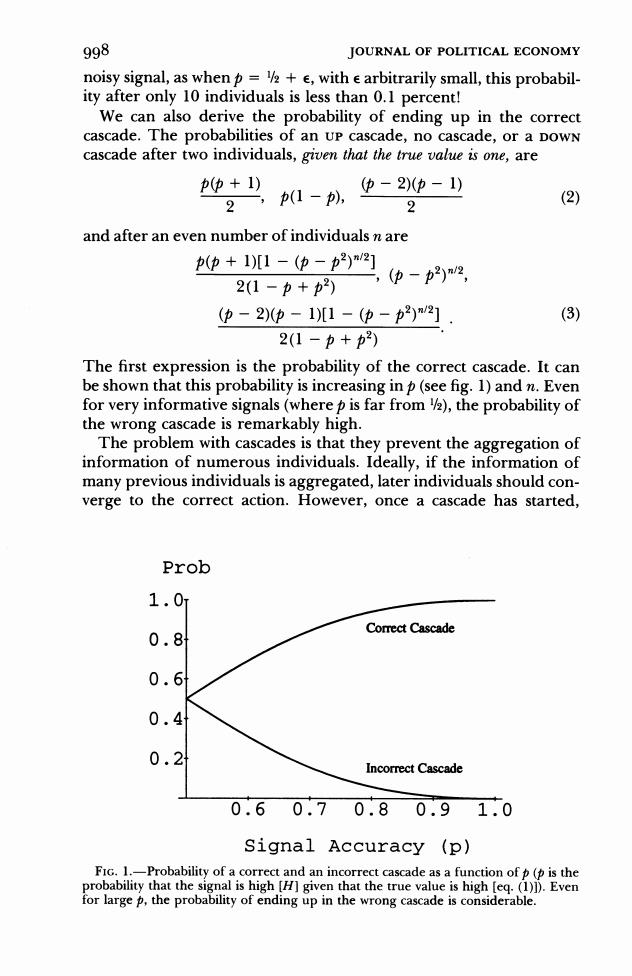
998 JOURNAL OF POLITICAL ECONOMY noisy signal,as whenp=+e,with e arbitrarily small,this probabil- ity after only 10 individuals is less than 0.1 percent! We can also derive the probability of ending up in the correct cascade.The probabilities of an Up cascade,no cascade,or a DowN cascade after two individuals,given that the true value is one,are 中+1) 2p1-, 中-2)中-1) 2 (2) and after an even number of individuals n are 中+1[1-(中-2)2] ,(中-2)2, 2(1-p+2) (中-2)(中-1)[1-(中-2)2] (3) 2(1-p+2) The first expression is the probability of the correct cascade.It can be shown that this probability is increasing in p(see fig.1)and n.Even for very informative signals(where p is far from )the probability of the wrong cascade is remarkably high. The problem with cascades is that they prevent the aggregation of information of numerous individuals.Ideally,if the information of many previous individuals is aggregated,later individuals should con- verge to the correct action.However,once a cascade has started, Prob 1.0 Correct Cascade 0.8 0.6 .41 2 Incorrect Cascade 0.60.70.80.91.0 Signal Accuracy (p) FIc.1.-Probability of a correct and an incorrect cascade as a function ofp(p is the probability that the signal is high [H]given that the true value is high [eq.(1)1).Even for large p,the probability of ending up in the wrong cascade is considerable

INFORMATIONAL CASCADES 999 actions convey no information about private signals;thus an individ- ual's action does not improve later decisions. Rogers and Shoemaker (1971)summarize research on the ability of outsiders(or"change agents")to bring about the adoption of desir- able innovations within communities.They offer the general proposi- tion that"change agent success is positively related to his efforts in increasing his clients'ability to evaluate innovations"(p.247).This is consistent with the prediction of this binary example that as the preci- sion of the signal,p,increases,a correct cascade starts with higher probability and,on average,earlier. It is instructive to compare the outcome in the previous-actions- observable(PAO)regime to that of the more informative previous- signals-observable(PSO)regime.In the binary signal case,PAO leads to a more uniform outcome.Following any given sequence of signal realizations,the two regimes lead to precisely identical outcomes,un- til a cascade begins in the PAO regime.However,in the PAO regime, after a cascade starts it is never reversed.In the PSO regime,even if an individual does not follow his private signal,it joins the common pool of knowledge.Hence,a long enough series of opposing signals will eventually cause people's behavior to switch.Thus the PAO leads to greater uniformity.We shall argue in Section V that this uniformity is brittle:small shocks can easily shift the behavior of many indi- viduals. B.A General Model We now show that under mild assumptions on the signals and values, cascades will always arise.Let there be a sequence of individuals i 1,2,...,n,...,each deciding whether to adopt some behavior or to reject it.Each individual observes the decisions of all those ahead of him.The order of individuals is exogenous and is known to all.All individuals have the same cost of adopting,C,and gain to adopting,V.The gain V has a finite set of possible values,v<v2 <..<vs,and the decision is not trivial (v<C<vs).The prior probability that V =v is denoted We use the concept of perfect Bayesian equilibrium.Since an indi- vidual's payoffs do not depend on what later individuals do,there is no incentive to make an out-of-equilibrium move to try to influence a later player.Thus,without loss of generality,we assume that if any player is observed to deviate from the equilibrium,either by rejecting when he should have adopted regardless of his signal realization or by adopting when he should have rejected regardless,then subsequent individuals have the same beliefs as though he had chosen his correct (equilibrium)action

1000 JOURNAL OF POLITICAL ECONOMY Individuals differ not only by their positions in the queue but by the signals they privately observe.Each individual i observes one of a conditionally independent and identically distributed sequence of signals X;with possible values x<x2<x3<...<xg.Let pat be the probability that an individual observes signal value x given a true value of adoption of v.We assume thata>0 for all g and l.Let P be the cumulative distribution of X;given V=v,that is, P=Pr(,=xlV=-之r Let J;be the set of signal realizations that lead individual i to adopt. His decision communicates to others that he observed either a signal in the set J;or its complement.IfJi=x1,x2,...,xg}or if Ji is empty,then individual i's action conveys no information about his realization. DEFINITION.An informational cascade occurs if an individual's ac- tion does not depend on his private information signal. If an individual i is in a cascade,then his action conveys no informa- tion and individual i+1 draws the same inference from all previous actions.Since the signal Xi+is drawn from the same distribution as Xi,individual i+1 is also in a cascade.Thus,by induction,all individ- uals after i are in a cascade.Consequently,a cascade once started will last forever,even if it is wrong.We shall see later that this fallibility causes cascades to be fragile.For instance,if individuals'signals have different distributions,as in Section IIC,if public information is re- vealed at a later date,as in Section IIIA,or if underlying values can change,as in Section V,then cascades can easily be broken. Let a;be individual i's action (adopt or reject)and let A;=(a1,a2,..., a,)represent the history of actions taken by individuals 1,2,...,i. Given history A:-1,leti(A:-1,a)be the set of signal realizations that lead individual i to choose action a;.Then individual n l's condi- tional expectation of V given his own signal realization x and the history An is Vn+1(cgAn)=E[VlXn+1=xg,X,∈J(A:-,a),for alli≤l. Individual n 1 adopts if1s V+1(A)C.Therefore,the infer- ence drawn from n I's action a+1 is that X+1En+(An,an+), where n+1(An,adopt)=[x such that V+1(A)=Ch, n+1(An,reject)={x such that V+1(xA)<C). is Since we are no longer restricting ourselves to a symmetric example,we use a tie-breaking assumption (indifferent individuals adopt)slightly different from the one in the previous section (indifferent individuals randomize).This reduces notation but not generality

INFORMATIONAL CASCADES 1001 We impose two regularity conditions.The first is that the condi- tional distributions Pr(XilV =v)are ordered by the monotone likeli- hood ratio property.4 This ensures that if an individual observes a higher signal realization,he infers that the value of adoption is higher (in a first-order stochastic dominance sense). AssUMPTIoN 1.Monotone likelihood ratio ordering.-For all l<S, L≥for all<R, Pg+1.1Pg+1,1+1 with strict inequality for at least one g. Assumption 1 ensures that the conditional expectation of each indi- vidual increases in his signal realization.Thus if individual i is not in a cascade and he adopts,later individuals conclude that X;=x for some q.If i does not adopt,then the conclusion is that X:< The second assumption ensures that if individuals learn enough about value by observing predecessors,then they are not indifferent between adopting and rejecting.15 ASSUMPTION2.No long-run ties..一M≠C for all, A major result of this section is that a cascade eventually begins. Suppose that an individual late in the sequence is still making a deci- sion based on his own information.Then the decisions of earlier individuals convey some information about their signals.If this indi- vidual is far enough down the line,then,by the strong law of large numbers,with probability close to one he can infer the true value of adoption with almost perfect certainty.But then his own signal contributes arbitrarily little to his information set,and he acts ac- cording to the information conveyed by the actions of previous indi- viduals.Therefore,he ignores his private information and starts a cascade. PRoposrTIoN 1.If assumptions 1 and 2 hold,then as the number of individuals increases,the probability that a cascade eventually starts approaches one. While we have shown that cascades must eventually occur,perhaps the more interesting point is that they will often be wrong.16 In an 1 The monotone likelihood ratio property is a standard assumption in models in which inferences must be drawn from a noisy signal.Milgrom(1981)provides a presen- tation and applications. i5 It is a mild assumption.If v,v,...,vs and C are drawn randomly from any nonatomic probability measure,assumption 2 is satisfied with probability one.This assumption prevents asymptotic indifference but is not a tie-breaking convention. 16 The proof of proposition 1 is based on the idea that many observations of informa- tive actions would lead with high probability to nearly perfect knowledge of value. Thus with high probability a cascade must start at or before such a point,but this cascade will often be an incorrect one that started much earlier.As fig.I in Sec.IIA shows,the probability of an incorrect cascade in the specific model can be close to.5 if the signal is noisy enough