
二、粒子的运动形式(The basic modes of motion) 1.平动(translation):分子在空间的整体运动。( )(motion through space in three dimensions) 2.转动(rotation):分子绕质心的转动。(二或三 维) 3.振动(vibration):原子在平衡位置附近的振动 4.电子运动(electron):分子内电子运动。 5.核运动(nucleus):分子内原子核运动。 若各种能量的运动形式彼此独立,则粒子的运动能 量为:e=e十e,+e,十ee十em PDF文件使用"pdfFactory Pro”试用版本创建酸能,fineprint.com,cn
二、粒子的运动形式(The basic modes of motion) 1.平动(translation):分子在空间的整体运动。( 三维)(motion through space in three dimensions) 2.转动(rotation):分子绕质心的转动。(二或三 维) 3.振动(vibration):原子在平衡位置附近的振动 4.电子运动(electron):分子内电子运动。 5.核运动(nucleus):分子内原子核运动。 若各种能量的运动形式彼此独立,则粒子的运动能 量为: e=et+er +ev +ee +en PDF 文件使用 "pdfFactory Pro" 试用版本创建 駿ÿ www.fineprint.com.cn

三、粒子运动的自由度数(Degrees of freedom of motion) 一个由N个原子组成的分子,运动的总自由度为3N。 自由度(3N) 平动 转动 振动 N原子 3 3 3N-6 单原子 3 0 0 双原子 3 2 1 PDF文件使用"pdfFactory Pro”试用版本创建晦.fineprint.com,cn
一个由N个原子组成的分子,运动的总自由度为3N。 三、粒子运动的自由度数(Degrees of freedom of motion) 自由度(3N) 平动 转动 振动 N原子 3 3 3N-6 单原子 3 0 0 双原子 3 2 1 PDF 文件使用 "pdfFactory Pro" 试用版本创建 ÿ昀www.fineprint.com.cn
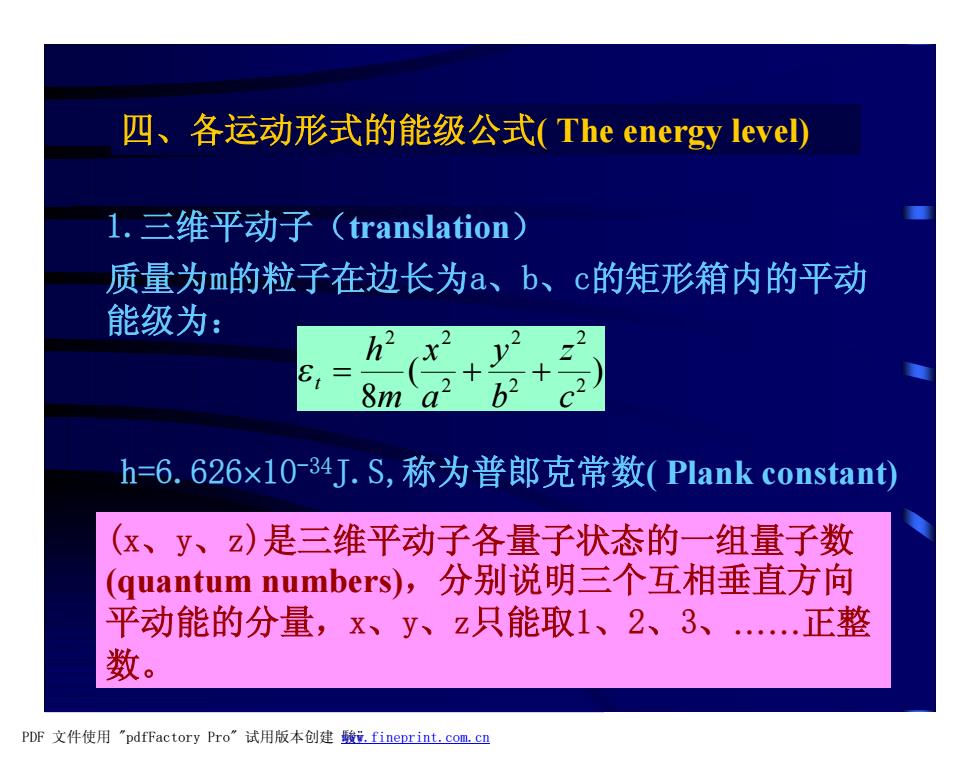
四、各运动形式的能级公式(The energy level) 1.三维平动子(translation) 质量为m的粒子在边长为a、b、c的矩形箱内的平动 能级为: 8m ae h=6.626x10-34J.S,称为普郎克常数(Plank constant) (x、y、z)是三维平动子各量子状态的一组量子数 (quantum numbers),分别说明三个互相垂直方向 平动能的分量,x、y、z只能取1、2、3、.正整 数。 PDF文件使用"pdfFactory Pro”试用版本创建駿能,fineprint.com,cn
四、各运动形式的能级公式( The energy level) 1.三维平动子(translation) 质量为m的粒子在边长为a、b、c的矩形箱内的平动 能级为: ( ) 8 2 2 2 2 2 2 2 c z b y a x m h et = + + h=6.626´10-34J.S,称为普郎克常数( Plank constant) .(x、y、z)是三维平动子各量子状态的一组量子数 (quantum numbers),分别说明三个互相垂直方向 平动能的分量,x、y、z只能取1、2、3、¼¼正整 数。 PDF 文件使用 "pdfFactory Pro" 试用版本创建 駿ÿ www.fineprint.com.cn

若a=b=c粒子在立方箱中则: h2 8: ++ 8m a- a 8mr2(r2+y2+z2 Discussion: 1)平动能基态(the lowest state):x,y,z=l,1,1,很小。 2)8=f(m,V) 3)有简并度(degenerate,.meaning that they correspond to the same energy)),例:第一微发态(the first excited state)可取(1,1,2),(1,2,1),(2,1, 1),g=3 4)△e/kT~10-19,量子效应(quantization)不明显,可近似 认为平动能量是连续的。 PDF文件使用"pdfFactory Pro”试用版本创建m,fineprint.com,c里
若a=b=c粒子在立方箱中则: ( ) 8 ( ) 8 2 2 2 2 / 3 2 2 2 2 2 2 2 2 x y z mV h a z a y a x m h et = + + = + + Discussion: 1)平动能基态( the lowest state):x,y,z=1,1,1,e0很小。 2)et =f(m,V) 3)有简并度(degenerate, meaning that they correspond to the same energy),例:第一激发态(the first excited state)可取(1,1,2),(1,2,1),(2,1, 1),gi=3 4) De/kT~10-19 ,量子效应(quantization)不明显,可近似 认为平动能量是连续的。 PDF 文件使用 "pdfFactory Pro" 试用版本创建 ÿwww.fineprint.com.cn ÿ

2.刚性直线转子(双原子分子)Rotational motion) 刚性直线转子是指原子间距离R不变的转子。 能级公式:8,=J(J+1)h2/8π2I J为转动量子数J=0,1,2,3...正整数 I=μR,2一转动惯量 μ=血m2/(m1+m2)--折合质量。 PDF文件使用"pdfFactory Pro”试用版本创建m,fineprint..com,cn
2.刚性直线转子(双原子分子)(Rotational motion) 刚性直线转子是指原子间距离R0不变的转子。 J为转动量子数 J=0,1,2,3¼¼正整数 I=mR0 2 ---转动惯量 m=m1m2/(m1+m2)---折合质量。 能级公式: er=J(J+1)h2/8p 2I PDF 文件使用 "pdfFactory Pro" 试用版本创建 f www.fineprint.com.cn Ì