
ANIDEAL GAS MEANS WHAT? It is one for which we assume that: 1)The molecules or atoms do not occupy any space or volume 2)The molecules do not interact with each other This is a bold assumption,but many gases obey the relationship quite well. PDF文件使用"pdfFactory Pro”试用版本创建脑m,fineprint.com,c里
7 It is one for which we assume that: 1) The molecules or atoms do not occupy any space or volume 2) The molecules do not interact with each other This is a bold assumption, but many gases obey the relationship quite well. AN “IDEAL GAS” MEANS WHAT? PDF 文件使用 "pdfFactory Pro" 试用版本创建 嘀www.fineprint.com.cn

PROPORTIONALITY CONSTANT R 'Molar Gas Constant'or 'Universal Gas Constant' The value of R depends on the units we use for P and V.The units of Tare always Kelvin(K)and 'n'is always the number of moles of material. R=8.314JK1mo1 when P in Pascals(Pa)and V in cubic metres(m3). (SI units) R=0.0821 Latm K-1 mol-1 when P in atmospheres(atm)and Vin litres (L). Can also use R=8.314 when Pin kPa and Vin litres which are mixed units but it works because Pa m3 (Px10)x(Vx10-3)the10-3and103 (kPa) () cancel each other out PDF文件使用"pdfFactory Pro”试用版本创建鳍m,fineprint.com,cn
8 The value of R depends on the units we use for P and V. The units of T are always Kelvin (K) and ‘n’ is always the number of moles of material. R = 8.314 JK-1 mol-1 when P in Pascals (Pa) and V in cubic metres (m3 ). (SI units) R = 0.0821 Latm K-1 mol-1 when P in atmospheres (atm) and V in litres (L). Can also use R = 8.314 when P in kPa and V in litres which are mixed units but it works because Pa m3 (P x 103 ) x (V x 10-3) the 10-3 and 103 (kPa) (L) cancel each other out PROPORTIONALITY CONSTANT R ‘Molar Gas Constant’ or ‘Universal Gas Constant’ PDF 文件使用 "pdfFactory Pro" 试用版本创建 嘀www.fineprint.com.cn
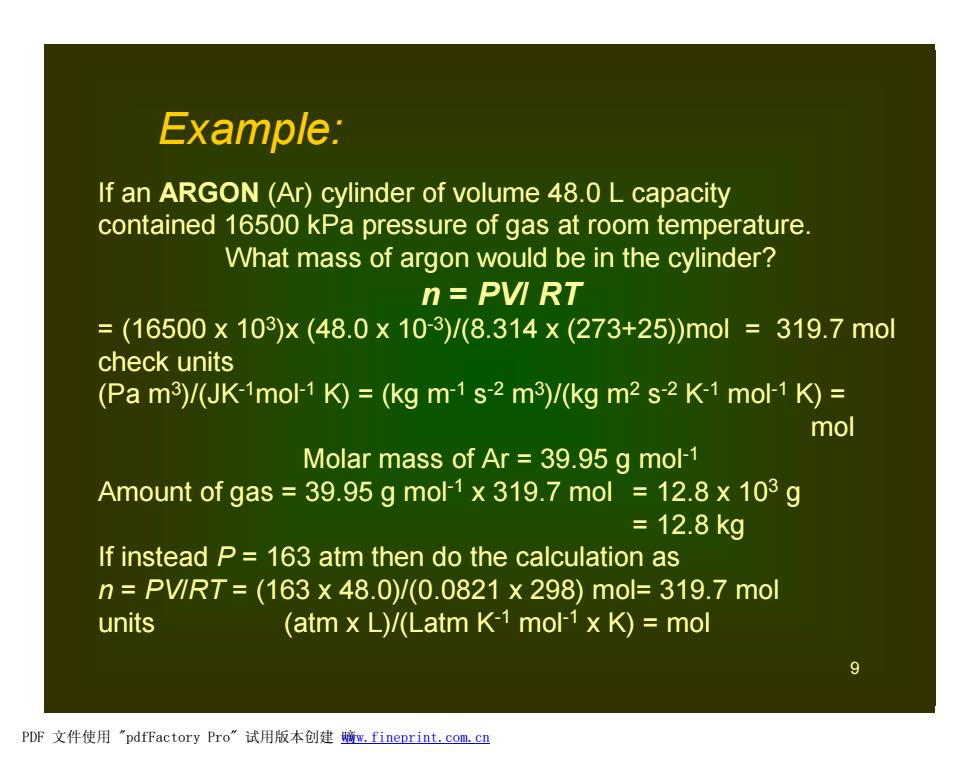
Example: If an ARGON (Ar)cylinder of volume 48.0 L capacity contained 16500 kPa pressure of gas at room temperature. What mass of argon would be in the cylinder? n=PVI RT =(16500×103)x(48.0×10-3)/(8.314×(273+25)mol=319.7mol check units (Pa m3)/(JK-1mol-1 K)=(kg m-1s-2 m3)/(kg m2 s-2 K-1 mol-1 K)= mol Molar mass of Ar 39.95 g mol-1 Amount of gas 39.95 g mol-1 x 319.7 mol 12.8 x 103 g =12.8kg If instead P=163 atm then do the calculation as n=PV1RT=(163x48.0)/(0.0821x298)mol=319.7mol units (atm x L)/(Latm K-1 mol-1 x K)=mol PDF文件使用"pdfFactory Pro”试用版本创建暗m,fineprint.com,c里
9 If an ARGON (Ar) cylinder of volume 48.0 L capacity contained 16500 kPa pressure of gas at room temperature. What mass of argon would be in the cylinder? n = PV/ RT = (16500 x 103 )x (48.0 x 10-3)/(8.314 x (273+25))mol = 319.7 mol check units (Pa m3 )/(JK-1mol-1 K) = (kg m-1 s -2 m3 )/(kg m2 s -2 K-1 mol-1 K) = mol Molar mass of Ar = 39.95 g mol-1 Amount of gas = 39.95 g mol-1 x 319.7 mol = 12.8 x 103 g = 12.8 kg If instead P = 163 atm then do the calculation as n = PV/RT = (163 x 48.0)/(0.0821 x 298) mol= 319.7 mol units (atm x L)/(Latm K-1 mol-1 x K) = mol Example: PDF 文件使用 "pdfFactory Pro" 试用版本创建 嘀www.fineprint.com.cn
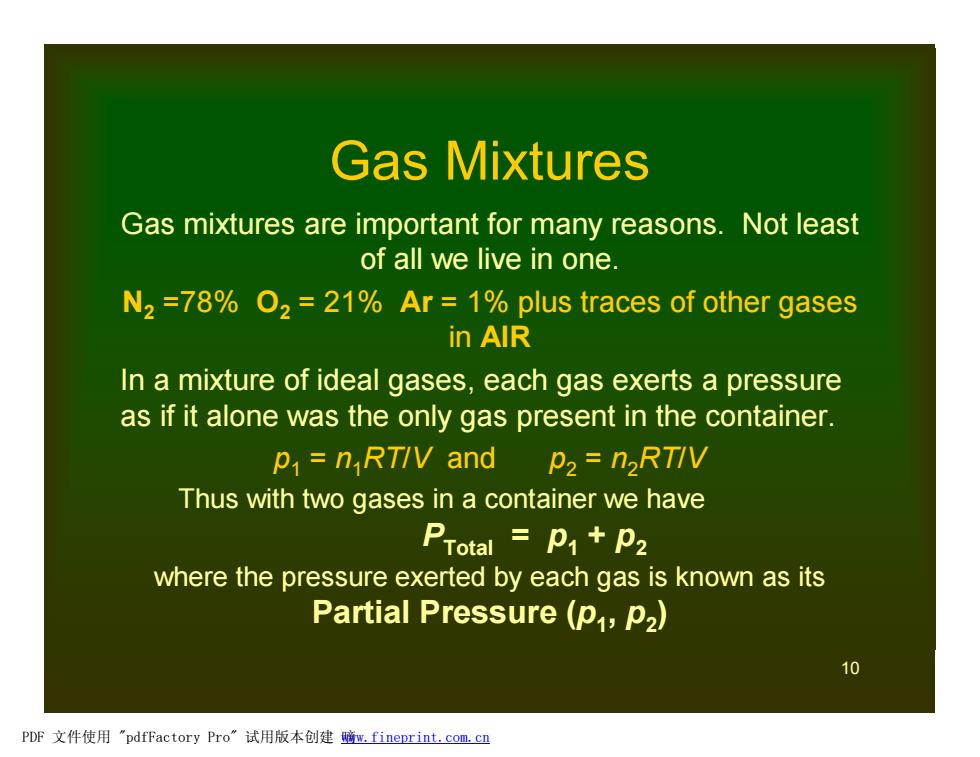
Gas Mixtures Gas mixtures are important for many reasons.Not least of all we live in one. N2=78%O2=21%Ar =1%plus traces of other gases in AlR In a mixture of ideal gases,each gas exerts a pressure as if it alone was the only gas present in the container. p=nRTIV and p2=n2RTIV Thus with two gases in a container we have PTotal p1+p2 where the pressure exerted by each gas is known as its Partial Pressure (p,P2) 10 PDF文件使用"pdfFactory Pro”试用版本创建鳍m,fineprint.com,cn
10 Gas Mixtures Gas mixtures are important for many reasons. Not least of all we live in one. N2 =78% O2 = 21% Ar = 1% plus traces of other gases in AIR In a mixture of ideal gases, each gas exerts a pressure as if it alone was the only gas present in the container. p1 = n1RT/V and p2 = n2RT/V Thus with two gases in a container we have PTotal = p1 + p2 where the pressure exerted by each gas is known as its Partial Pressure (p1 , p2 ) PDF 文件使用 "pdfFactory Pro" 试用版本创建 嘀www.fineprint.com.cn
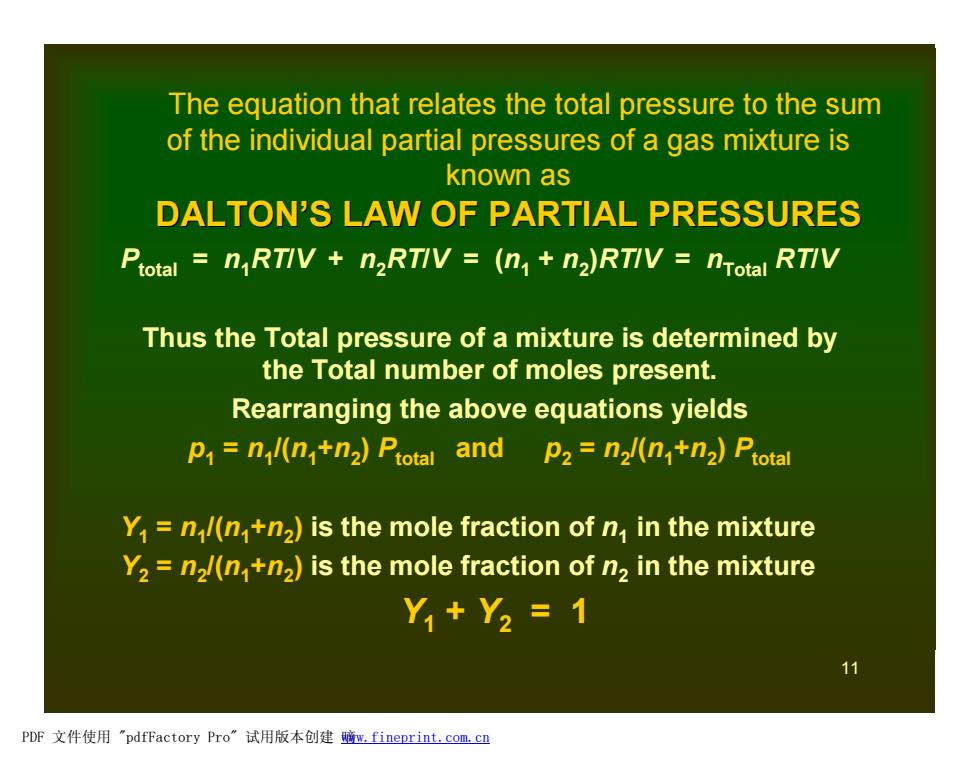
The equation that relates the total pressure to the sum of the individual partial pressures of a gas mixture is known as DALTON'S LAW OF PARTIAL PRESSURES Ptotal nRTIV n2RTIV =(n+n2)RTIV nTotal RTIV Thus the Total pressure of a mixture is determined by the Total number of moles present. Rearranging the above equations yields p=nl(n+n2)Ptotal and p2=n2l(n+n2)Ptotal Y1 n/(n+n2)is the mole fraction of n in the mixture Y2 n2/(n+n2)is the mole fraction of n2 in the mixture Y,+Y2=1 11 PDF文件使用"pdfFactory Pro”试用版本创建脑m,fineprint.com,cn
11 The equation that relates the total pressure to the sum of the individual partial pressures of a gas mixture is known as DALTON’S LAW OF PARTIAL PRESSURES Ptotal = n1RT/V + n2RT/V = (n1 + n2 )RT/V = nTotal RT/V Thus the Total pressure of a mixture is determined by the Total number of moles present. Rearranging the above equations yields p1 = n1 /(n1+n2 ) Ptotal and p2 = n2 /(n1+n2 ) Ptotal Y1 = n1 /(n1+n2 ) is the mole fraction of n1 in the mixture Y2 = n2 /(n1+n2 ) is the mole fraction of n2 in the mixture Y1 + Y2 = 1 PDF 文件使用 "pdfFactory Pro" 试用版本创建 嘀www.fineprint.com.cn