
Parameter Estimation and Evaluation Population and Distribution Model Population and Distribution Model Suppose T is the duration from a population with PDF f(t) and CDF F(t).Then the survival function is S(t)=P(T>t)=1-F(t), and the hazard rate P(t<T:≤t+oT:>t) 入(t) lim 6→0+ f(t) S(t) Intuitively,the hazard rate A(t)is the instantaneous proba- bility that an event of interest will end at time t given that it has lasted for period t.Note that the specification of A(t) is equivalent to a specification of PDF f(t),but A(t)is more interpretable from an economic point of view. Parameter Estimation and Evaluation Introduction to Statistics and Econometrics April 21,2020 11/207
Parameter Estimation and Evaluation Parameter Estimation and Evaluation Introduction to Statistics and Econometrics April 21, 2020 11/207 Population and Distribution Model Population and Distribution Model
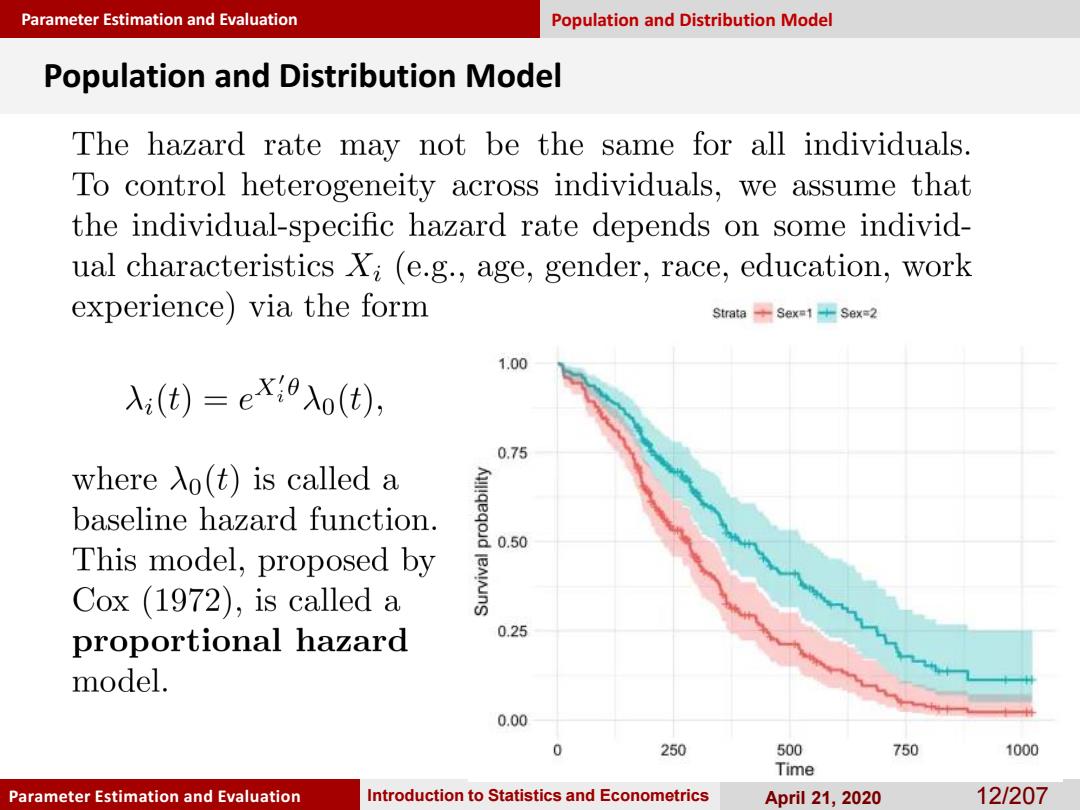
Parameter Estimation and Evaluation Population and Distribution Model Population and Distribution Model The hazard rate may not be the same for all individuals. To control heterogeneity across individuals,we assume that the individual-specific hazard rate depends on some individ- ual characteristics Xi (e.g.,age,gender,race,education,work experience)via the form Strata→Sex=1+Sex=2 1.00 Xi(t)ex:0Xo(t), 0.75 whereλo(t)is called a baseline hazard function. 0e9o, This model,proposed by Cox (1972),is called a proportional hazard 0.25 model. 0.00 0 250 500 750 1000 Time Parameter Estimation and Evaluation Introduction to Statistics and Econometrics April 21,2020 12/207
Parameter Estimation and Evaluation Parameter Estimation and Evaluation Introduction to Statistics and Econometrics April 21, 2020 12/207 Population and Distribution Model Population and Distribution Model
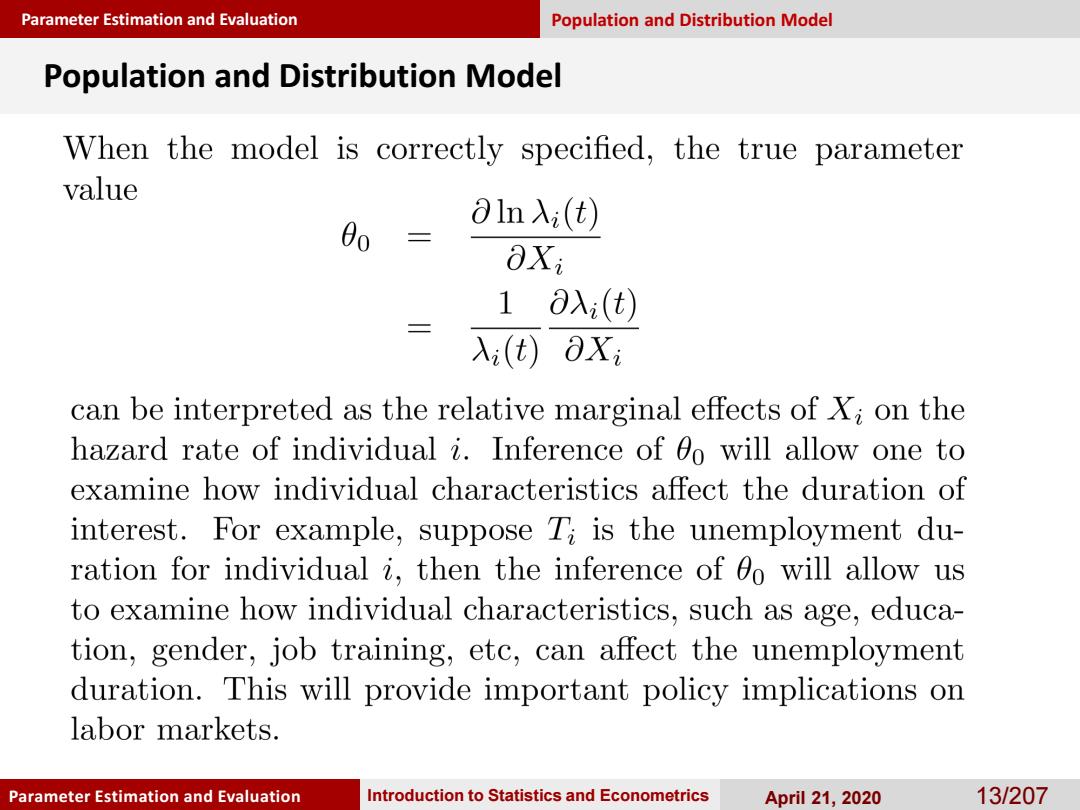
Parameter Estimation and Evaluation Population and Distribution Model Population and Distribution Model When the model is correctly specified,the true parameter value aln入(t) ∂Xi 10入(t) λ(t)∂Xi can be interpreted as the relative marginal effects of X;on the hazard rate of individual i.Inference of 0o will allow one to examine how individual characteristics affect the duration of interest.For example,suppose T is the unemployment du- ration for individual i,then the inference of 0o will allow us to examine how individual characteristics,such as age,educa- tion,gender,job training,etc,can affect the unemployment duration.This will provide important policy implications on labor markets. Parameter Estimation and Evaluation Introduction to Statistics and Econometrics April 21,2020 13/207
Parameter Estimation and Evaluation Parameter Estimation and Evaluation Introduction to Statistics and Econometrics April 21, 2020 13/207 Population and Distribution Model Population and Distribution Model

Parameter Estimation and Evaluation Population and Distribution Model Population and Distribution Model Because one can obtain the conditional PDF of Yi given Xi as follows: f(t)=λ(t)S(t), where the survival function S(t)=eJλx(s)ds we can estimate 0o by the so-called maximum likelihood es- timation (MLE)method. Parameter Estimation and Evaluation Introduction to Statistics and Econometrics April 21,2020 14/207
Parameter Estimation and Evaluation Parameter Estimation and Evaluation Introduction to Statistics and Econometrics April 21, 2020 14/207 Population and Distribution Model Population and Distribution Model

Parameter Estimation and Evaluation Population and Distribution Model Population and Distribution Model Remarks: Usually,the true parameter value 0o is unknown,and one is interested in making inference of 0o using an observed data x". Traditionally,problems of statistical inference are di- vided into problems of estimation and hypothesis test- ing.In this chapter,we are interested in estimating 00. We shall introduce two most commonly used estima- tion methods,namely the maximum likelihood estima- tion (MLE)and the generalized method of moments estimation (GMM). Parameter Estimation and Evaluation Introduction to Statistics and Econometrics April 21,2020 15/207
Parameter Estimation and Evaluation Parameter Estimation and Evaluation Introduction to Statistics and Econometrics April 21, 2020 15/207 Population and Distribution Model Population and Distribution Model Remarks: