
中国科学院数学与系统科学研究院 Academy of Mathematics and Systems Science Chinese Academy of Sciences Classical Linear Regression Model Professor Yongmiao Hong March 24.2021
Classical Linear Regression Model Professor Yongmiao Hong March 24, 2021

CONTENTS 3.1 Framework and Assumptions 3.2 Ordinary Least Squares (OLS)Estimation 3.3 Goodness of Fit and Model Selection Criteria 3.4 Consistency and Efficiency of OLS 3.5 Sampling Distribution of OLS 3.6 Variance Estimation for OLS 3.7 Hypothesis Testing ADVANCED ECONOMETRICS Classical Linear Regression Model May11,2021 2
ADVANCED ECONOMETRICS Classical Linear Regression Model May 11, 2021 2 3.1 Framework and Assumptions 3.2 Ordinary Least Squares (OLS) Estimation 3.3 Goodness of Fit and Model Selection Criteria 3.4 Consistency and Efficiency of OLS 3.5 Sampling Distribution of OLS 3.6 Variance Estimation for OLS 3.7 Hypothesis Testing CONTENTS

CONTENTS 3.8 Applications 3.9 Generalized Least Squares (GLS) Estimation 3.10 Conclusion ADVANCED ECONOMETRICS Classical Linear Regression Model May11,2021 3
ADVANCED ECONOMETRICS Classical Linear Regression Model May 11, 2021 3 3.8 Applications 3.9 Generalized Least Squares (GLS) Estimation 3.10 Conclusion CONTENTS
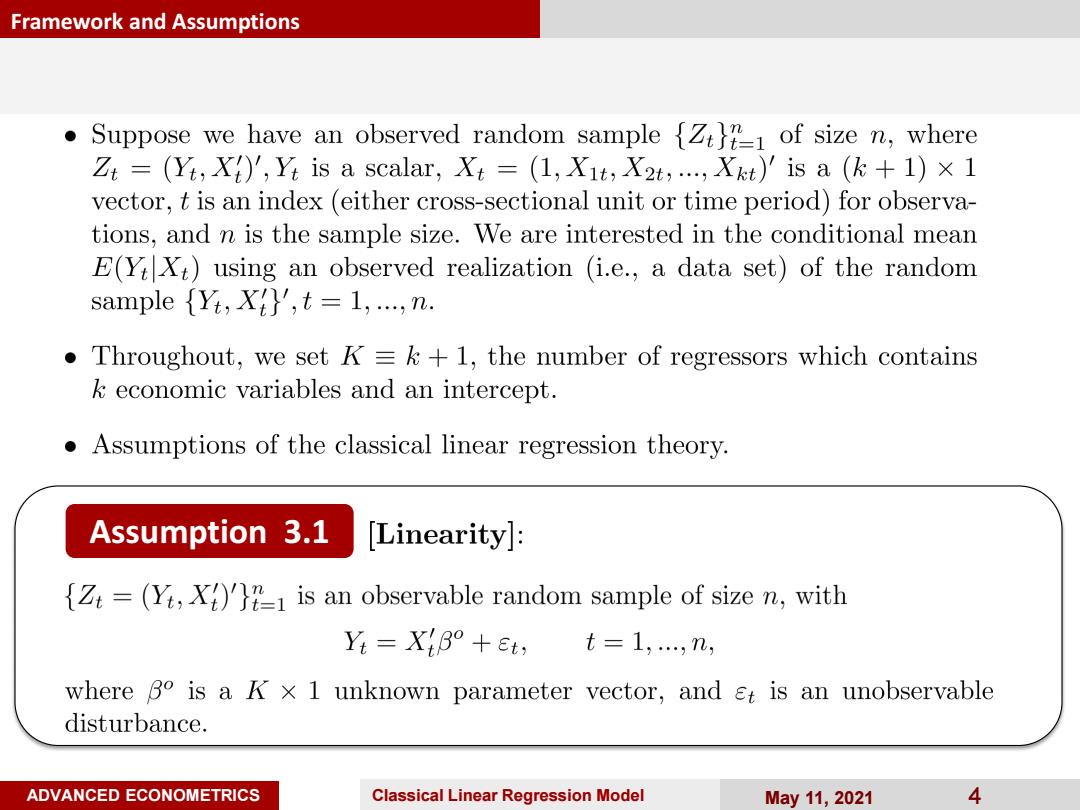
Framework and Assumptions Suppose we have an observed random sample {Z1 of size n,where Zt =(Yt;X)',Yt is a scalar,Xt =(1,Xit;X2t,..Xit)'is a (k+1)x 1 vector,t is an index(either cross-sectional unit or time period)for observa- tions,and n is the sample size.We are interested in the conditional mean E(YiXt)using an observed realization (i.e.,a data set)of the random sample (rt,xi',t =1,....n. Throughout,we set K=k+1,the number of regressors which contains k economic variables and an intercept. Assumptions of the classical linear regression theory. Assumption 3.1 [Linearity]: Z=(Yi,X)')1 is an observable random sample of size n,with Y=XtB°+et, t=1,,n, where Bo is a k x 1 unknown parameter vector,and et is an unobservable disturbance. ADVANCED ECONOMETRICS Classical Linear Regression Model May11,2021 4
ADVANCED ECONOMETRICS Classical Linear Regression Model May 11, 2021 4 Framework and Assumptions Assumption 3.1
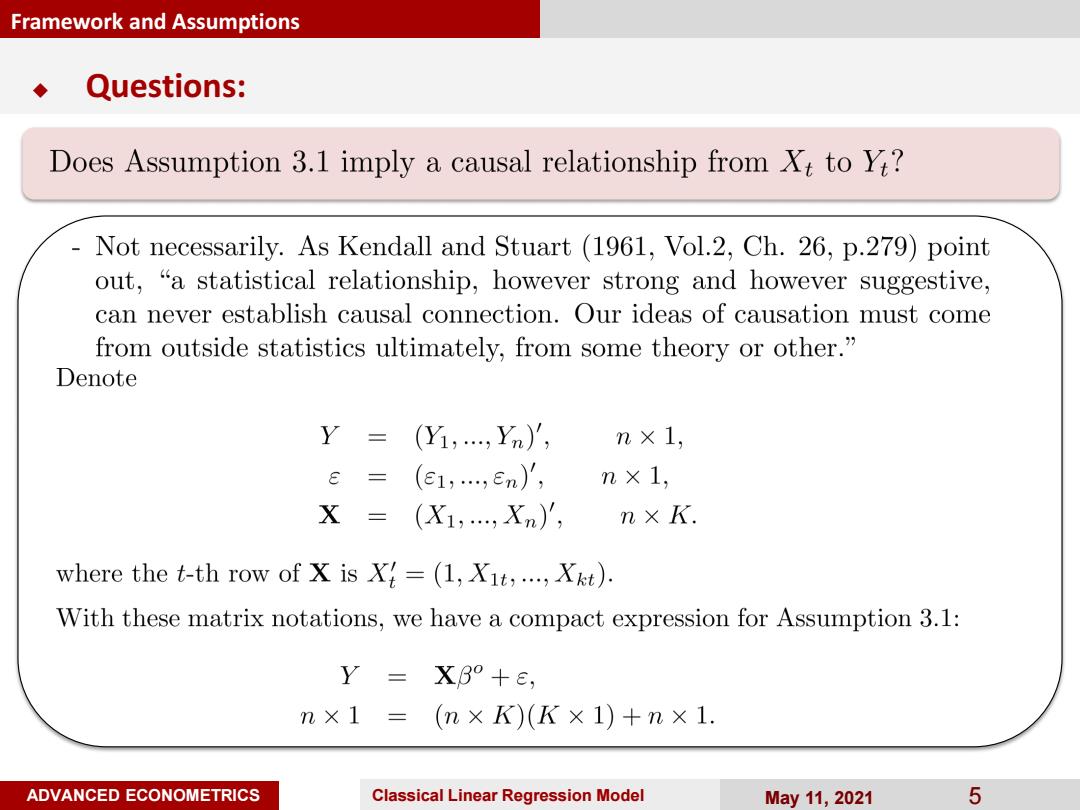
Framework and Assumptions Questions: Does Assumption 3.1 imply a causal relationship from Xt to Yi? Not necessarily.As Kendall and Stuart (1961,Vol.2,Ch.26,p.279)point out,"a statistical relationship,however strong and however suggestive, can never establish causal connection.Our ideas of causation must come from outside statistics ultimately,from some theory or other." Denote Y= (Yi,,Yn)', n×1, E= (e1,,en)/, n×1, X=(X1,,Xm), n×K. where the t-th row of X isX=(1,Xit,...,Xit). With these matrix notations,we have a compact expression for Assumption 3.1: Y= XB°+e, n×1=(n×K)(K×1)+n×1. ADVANCED ECONOMETRICS Classical Linear Regression Model May11,2021 5
ADVANCED ECONOMETRICS Classical Linear Regression Model May 11, 2021 5 Framework and Assumptions Questions: