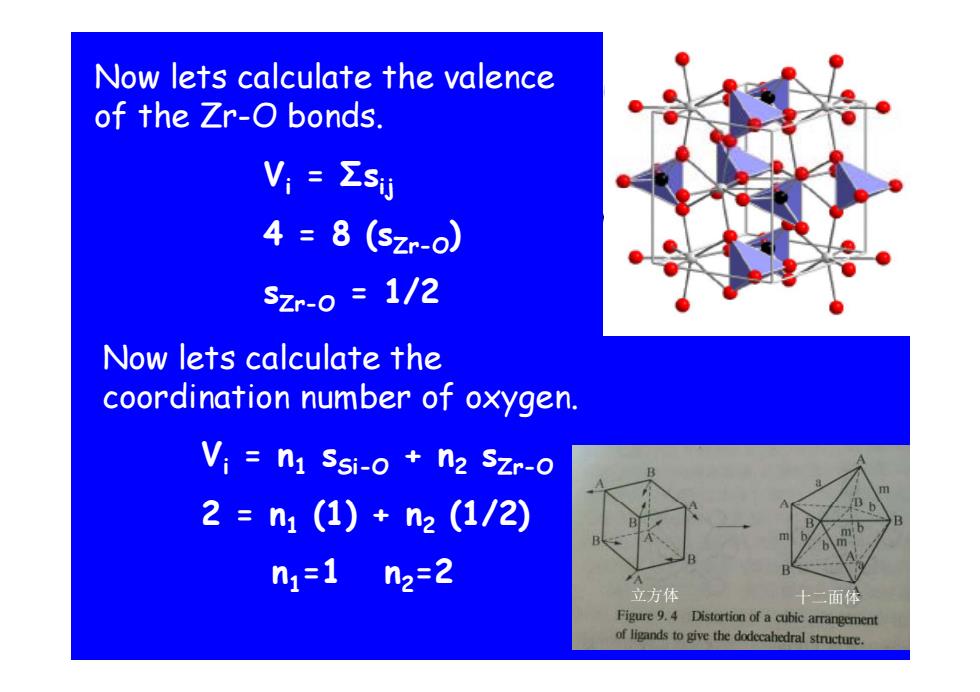
Now lets calculate the valence of the Zr-O bonds. V,=ΣsU 4=8(szr-o) Szr-0=1/2 Now lets calculate the coordination number of oxygen. Vi n1 Ssi-o n2 Szr-0 2=n1(1)+n2(1/2) n1=1n2=2 立方体 十二面体 Figure 9.4 Distortion of a cubic arrangement of ligands togive the dodecahedral structure
Now lets calculate the valence of th Ze Zr-O b nds O b onds. Vi = Σ sij 4 = 8 (sZr-O ) sZr-O = 1/2 N l ll h Now lets calculate t he coordination number of oxygen. Vi = n 1 sSi-O + n 2 sZr-O 2 = n (1) (1/2) 1 + n 2 (1/2) n 1 =1 n 2 = 2 立方体 十二面体
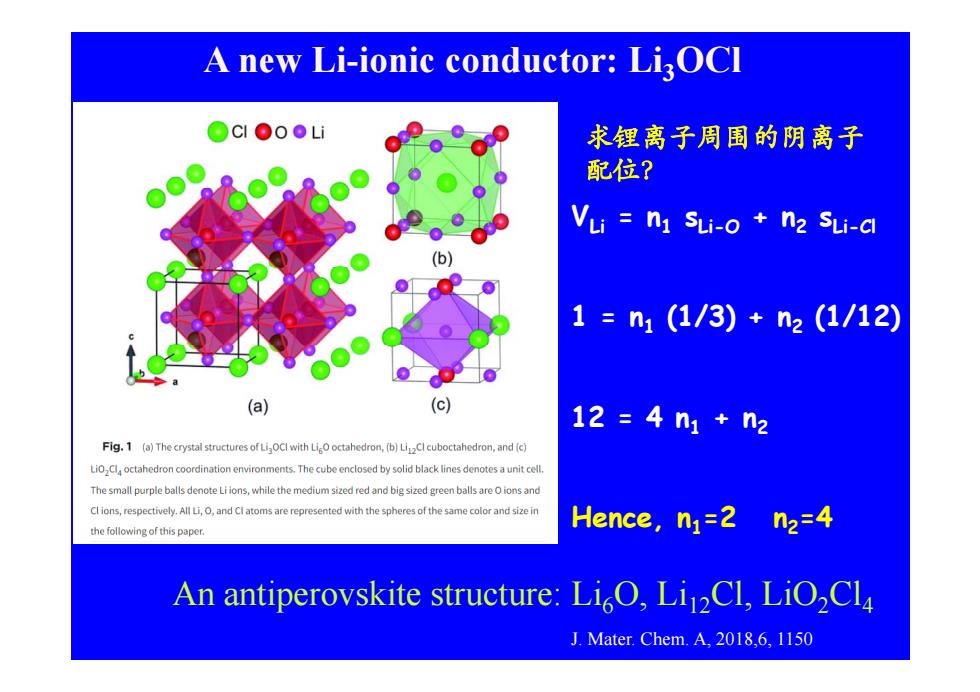
A new Li-ionic conductor:LiOCl OCIoLi 求锂离子周围的阴离子 配位? VLi n1 SLi-o n2 SLi-a (b) 1=n1(1/3)+n2(1/12) (a) (c) 12=4n1+n2 Fig.1 (a)The crystal structures of LigOCl with LiO octahedron,(b)LiCl cuboctahedron,and (c) coordination environments.The cube enclosed by solid black lines denotes a unitcell The small purple balls denote Li ions,while the medium sized red and big sized green balls are Oions and Clions,respectively.All Li,and Clatoms are represented with the spheres of the same color and size in the following of this paper. Hence,nj=2 n2=4 An antiperovskite structure:LiO,LiCl,LiO2Cla J.Mater.Chem.A,2018,6,1150
A new Li-ionic conductor: Li 3OCl 求锂离子周围的阴离子 配位? VLi = n 1 sLi-O + n 2 sLi-Cl 1 = n 1 (1/3) + n 2 (1/12) 12 = 4 n 1 + n 2 A i ki i O i Cl iO Cl Hence, n 1=2 n 2=4 An ant iperovskite structure: L i 6 O, L i12Cl, LiO 2Cl 4 J. Mater. Chem. A, 2018,6, 1150

§Pauling第二规则的发展和应用 -定量键价方法(Quantitative Bond Valence Method) West,p.231 Bond valence 20 75 39 Na,O (反萤石结构) Bond length (A) 氧与第二周期原子Na,Mg,AL,Si,P,S之间的键价-键长关系(I.D Brown,.1978)[O-M键长:1.43(S0),1.60,1.62(SiO22.65Na2O] Sy=(Ro/R)N P,Os ·式中R是键长,R,、N为常数(R,是单位键价的键长值) ◆对上图代表的元素,R=1.622,N=4.290
§ Pauling Pauling第二规则的发展和应用 第二规则的发展和应用 -定量键价方法 (Quantitative Bond Valence Method) West, p.231 价 Q N O 氧与第二周期原子Na, Mg, Al, Si, P, S之间的键价-键长关系(I.D. Na2O (反萤石结构) N (R / R) Brown, 1978)[O-M键长:1.43(SO2); 1.60,1.62(SiO2); 2.65(Na2O)] N sij (R / R) 0 式中R是键长 R N为常数(R 是单位键价的键长值) P2O5 式中R是键长,R0、N为常数(R0是单位键价的键长值); 对上图代表的元素,R0=1.622, N=4.290
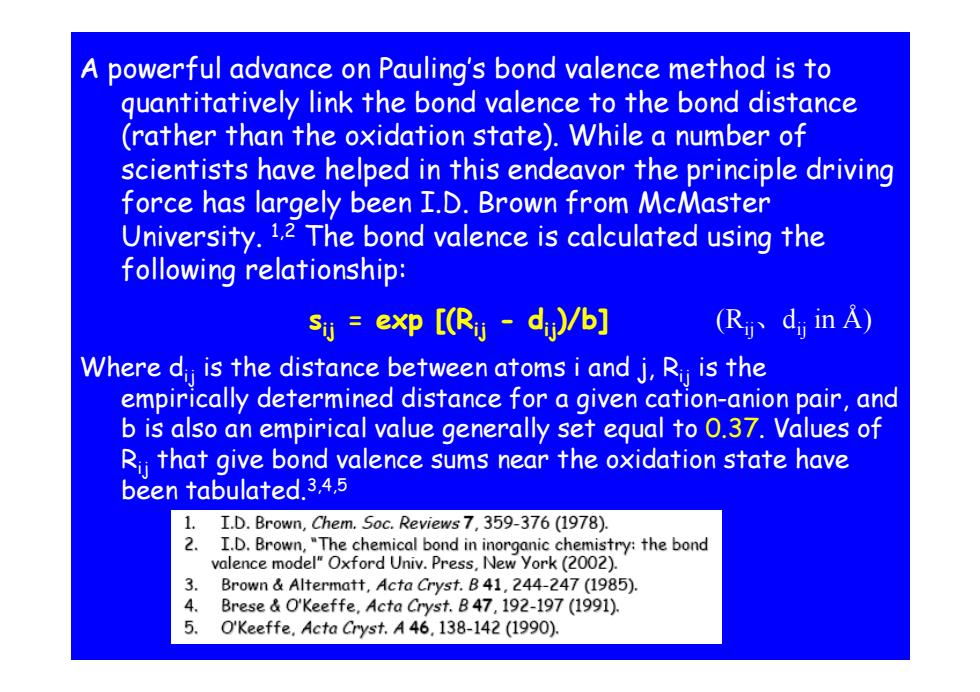
A powerful advance on Pauling's bond valence method is to guantitatively link the bond valence to the bond distance (rather than the oxidation state).While a number of scientists have helped in this endeavor the principle driving force has largely been I.D.Brown from McMaster University.1.2 The bond valence is calculated using the following relationship: si exp [(Rij dj)/b] R、dinA) Where d is the distance between atoms i and j,Ri is the empirically determined distance for a given cation-anion pair,and b is also an empirical value generally set equal to 0.37.Values of Rj that give bond valence sums near the oxidation state have been tabulated.3,4.5 1. I.D.Brown,Chem.Soc.Reviews 7,359-376(1978). 2.I.D.Brown,"The chemical bond in inorganic chemistry:the bond valence model"Oxford Univ.Press,New york(2002). 3 Brown Altermatt,Acta Cryst.B41,244-247 (1985). 4. Brese O'Keeffe,Acta Cryst.B47,192-197(1991). 5. O'Keeffe.Acta Cryst.A 46.138-142(1990)
A powerful advance on Pauling’s bond valence method is to quantitatively link the bond valence to the bond distance quantitatively link the bond valence to the bond distance (rather than the oxidation state). While a number of scientists have helped in this endeavor the principle driving force has largely been I.D. Brown from McMaster University. 1,2 The bond valence is calculated using the following relationship: following relationship: sij = exp [(Rij - dij)/b] (Rij 、 dij in Å) Where dij is the distance between atoms i and j, Rij is the empirically determined distance for a given cation-anion pair, and b is als an empirical value enerally set equal t b is also an empirical value generally set equal to 0 37 . . Values f Values o f Rij that give bond valence sums near the oxidation state have been tabulated.3,4,5
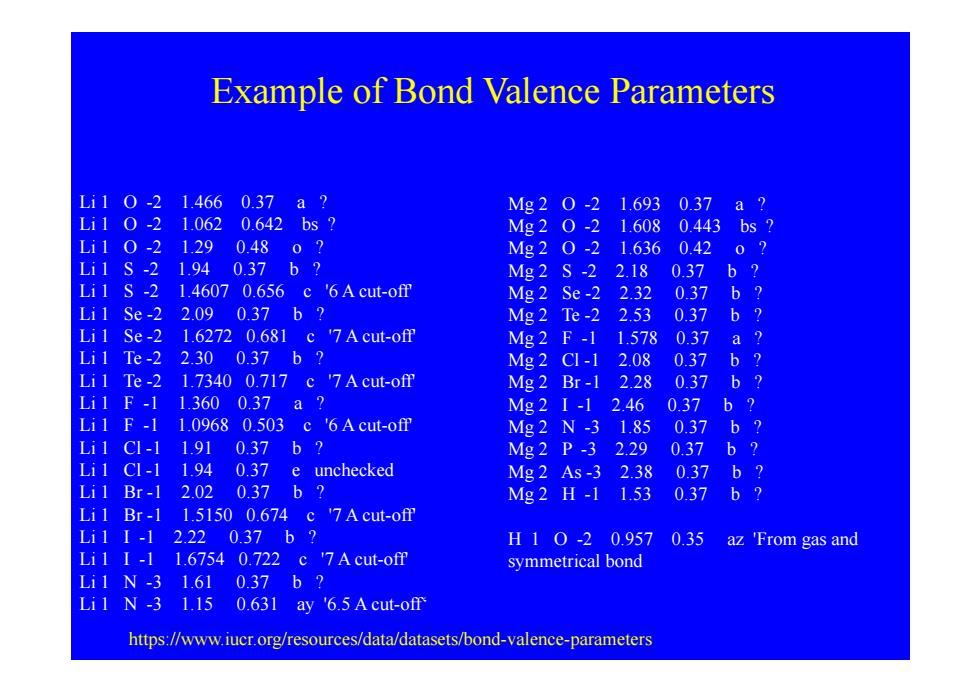
Example of Bond Valence Parameters Li10-21.4660.37a? Mg20-21.6930.37a? Li10-21.0620.642bs? Mg20-21.6080.443bs2 Li10-21.290.480? Mg20-21.6360.420? Li1S-21.940.37b? Mg2S-22.180.37b? Li1 S-2 1.4607 0.656 c '6A cut-off Mg2Se-22.320.37b? Li1Se-22.090.37b? Mg2Te-22.530.37b? Li1 Se-2 1.6272 0.681 c '7A cut-off Mg2F-11.5780.37a? Li1Te-22.300.37b? Mg2CI-12.08 0.37b? Li1 Te-2 1.7340 0.717 c '7A cut-off Mg2Br-12.280.37b? Li1F-11.3600.37a? Mg21-12.460.37b? Li1 F-1 1.0968 0.503 c '6A cut-off Mg2N-31.850.37b? Li1C1-11.91037b? Mg2P-32.29 0.37b? Li1 CI-1 1.94 0.37 e unchecked Mg2As-32.38 0.37b? Li1Br-12.020.37b? Mg2H-11.53 0.37 b? Li1 Br-1 1.5150 0.674 c '7A cut-off Lil I-1 2.220.37b? H10-20.957 0.35 az 'From gas and Li11-11.67540.722c7Acut-ofm symmetrical bond Li1N-31.610.37b? Li1 N-3 1.15 0.631 ay '6.5 Acut-off https://www.iucr.org/resources/data/datasets/bond-valence-parameters
Example of Bond Valence Parameters Li 1 O -2 1.466 0.37 a ? Li 1 O -2 1.062 0.642 bs ? Li 1 O -2 1.29 0.48 o ? Li 1 S 2 1 94 0 37 b ? Mg 2 O -2 1.693 0.37 a ? Mg 2 O -2 1.608 0.443 bs ? Mg 2 O -2 1.636 0.42 o ? Li 1 S -2 1.94 0.37 b ? M2S 2 2 18 0 37 b ? Li 1 S -2 1.4607 0.656 c '6 A cut-off' Li 1 Se -2 2.09 0.37 b ? Li 1 Se -2 1.6272 0.681 c '7 A cut-off' Mg 2 S -2 2.18 0.37 b ? Mg 2 Se -2 2.32 0.37 b ? Mg 2 Te -2 2.53 0.37 b ? Mg 2 F -1 1.578 0.37 a ? Li 1 Te -2 2.30 0.37 b ? Li 1 Te -2 1.7340 0.717 c '7 A cut-off' Li 1 F -1 1.360 0.37 a ? Li 1 F 1 1 0968 0 503 c '6 A cut off' g Mg 2 Cl -1 2.08 0.37 b ? Mg 2 Br -1 2.28 0.37 b ? Mg 2 I -1 2.46 0.37 b ? Li 1 F -1 1.0968 0.503 c 6 A cut-off M2N 3 1 85 0 37 b ? Li 1 Cl -1 1.91 0.37 b ? Li 1 Cl -1 1.94 0.37 e unchecked Li 1 Br -1 2.02 0.37 b ? Mg 2 N -3 1.85 0.37 b ? Mg 2 P -3 2.29 0.37 b ? Mg 2 As -3 2.38 0.37 b ? Mg 2 H -1 1.53 0.37 b ? Li 1 Br -1 1.5150 0.674 c '7 A cut-off' Li 1 I -1 2.22 0.37 b ? Li 1 I -1 1.6754 0.722 c '7 A cut-off' Li 1 N 3 1 61 0 37 b ? g H 1 O -2 0.957 0.35 az 'From gas and symmetrical bond Li 1 N -3 1.61 0.37 b ? Li 1 N -3 1.15 0.631 ay '6.5 A cut-off‘ https://www.iucr.org/resources/data/datasets/bond-valence-parameters