电子科技大学：《信号检测与估计 Signal Detection and Estimation》课程教学资源（课件讲稿）Chapter 11 Estimation of Specific Parameters

/9 The minimum variance of the unbiased estimate is given by For the continuous case this result can be expressed as N dt UESTC 6

The minimum variance of the unbiased estimate is given by For the continuous case this result can be expressed as 6 UESTC ( ) ( ) 2 2 2 1 min k i i s     = =          α ( ) ( ) 2 0 2 0 α , 2 min T N s t dt    =         

11.4 Amplitude estimation in the coherent case with WGN Assume the phase is known and the amplitude must be estimated. y;=aS+n,i=1,.,k The amplitude a can either be a random variable of an unknown constant. ML: -a四-0+2（0ag0 i=l Symbol energy UESTC ∑y e=∑s 7 i=l

11.4 Amplitude estimation in the coherent case with WGN Assume the phase is known and the amplitude must be estimated. The amplitude a can either be a random variable of an unknown constant. ML: 7 UESTC , 1, , i i i y n = + = as i k ( ( )) ( ) 1 0 k i i i i s y s   =   − =   ( ) 1 0 k i i i i as s =  y − = 1 1 k ML i i i a s = = y ε 2 1 k i i s  = ε= Symbol energy

The mean of this estimate is Ea=a,it is unbiased.The variance of it is .According to we have o≥o2/e It can be seen that the estimate is efficient. A MAP estimate can be obtained by selecting an a priori distribution of p(a). UESTC 8

The mean of this estimate is , it is unbiased. The variance of it is . According to we have It can be seen that the estimate is efficient. A MAP estimate can be obtained by selecting an a priori distribution of . 8 UESTC E a aML = 2  ε ( ) ( ) 2 2 2 1 min k i i s     = =          α 2 2   aˆ  ε p a( )

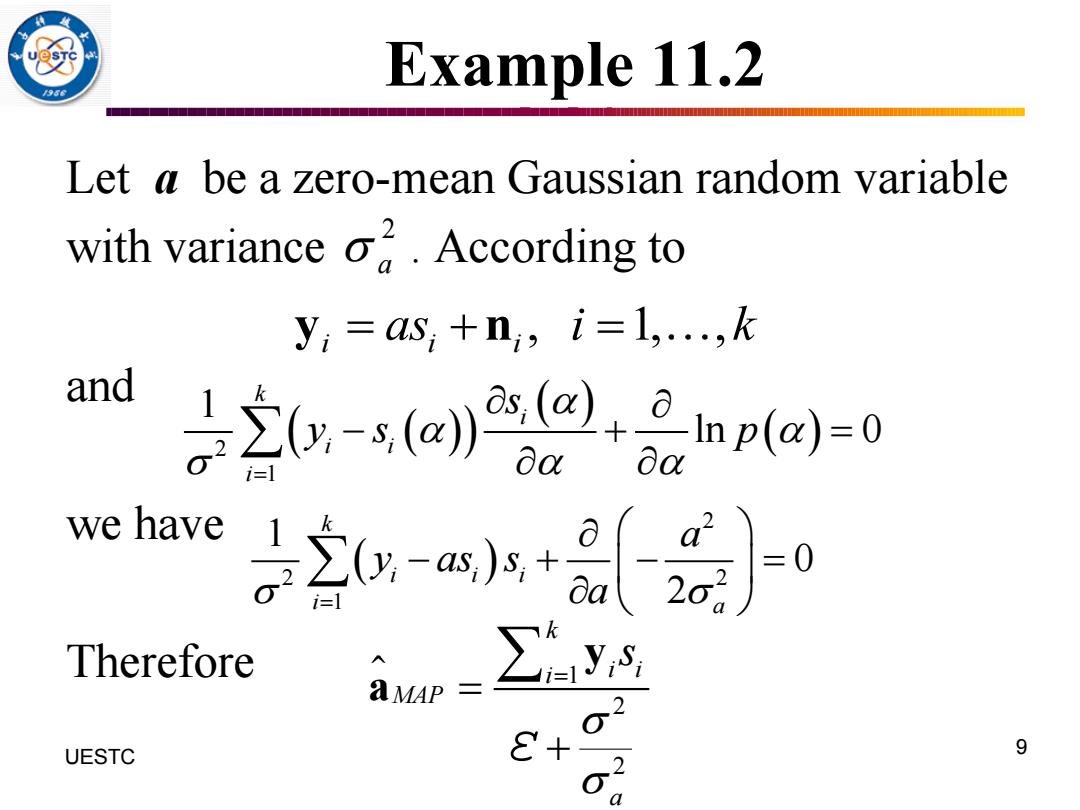
Example 11.2 Let a be a zero-mean Gaussian random variable with varianceo.According to y:=aS,+n,i=1,…,k and 空to但ana-0 aa we have 合0w+}-0 Therefore ∑y aMAP 2 UESTC E+ 9 2

Example 11.2 Let a be a zero-mean Gaussian random variable with variance . According to and we have Therefore 9 UESTC 2  a , 1, , i i i y n = + = as i k ( ( )) ( ) ( ) 2 1 1 ln 0 k i i i i s y s p       =   − + =    ( ) 2 2 2 1 1 0 2 k i i i i a a y as s   = a    − + − =       1 2 2 k i i i MAP a s   = = +  y a ε

Since p(a)is Gaussian in this example,the posteriori pdf p(is 点m最-ar+刘 Letting 2 K We have UESTC 10

Since is Gaussian in this example, the posteriori pdf is Letting We have 10 UESTC p a( ) p a y ( ) ( ) ( ) 2 2 2 2 2 2 2 1 1 exp exp 2 2 2 2 k i i i i a i a a p a y y as y a s     =       = − − − +              2 2 0 2 2 2 2 1 1 1 exp 2 2 2 k MAP i i a a a y       =     = − + +              ε ( ) ( ) 2 0 2 2 1 1 exp 2 MAP a p a y a a        = − + −             ε