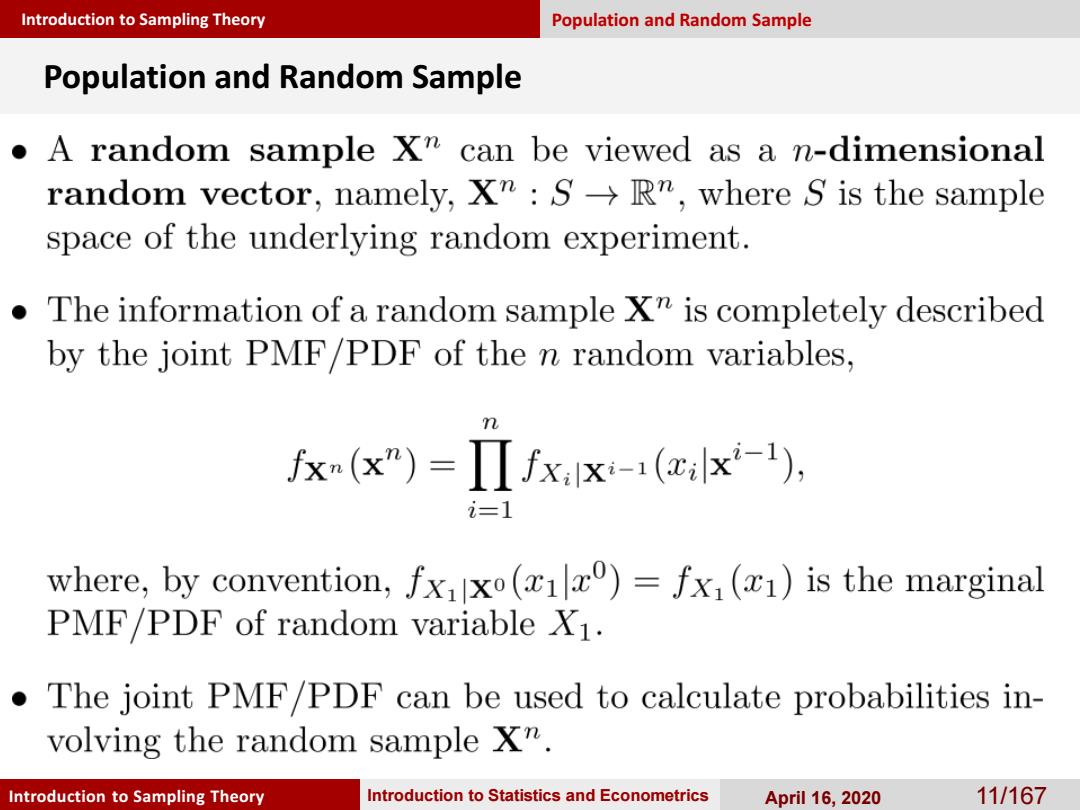
Introduction to Sampling Theory Population and Random Sample Population and Random Sample .A random sample X"can be viewed as a n-dimensional random vector,namely,Xm:S-R",where S is the sample space of the underlying random experiment. The information of a random sample X is completely described by the joint PMF/PDF of the n random variables, fxn(x”)=Πfx:x-1(clx-1), i=1 where,by convention,fxxo(i)=fx(1)is the marginal PMF/PDF of random variable X1. The joint PMF/PDF can be used to calculate probabilities in- volving the random sample X". Introduction to Sampling Theory Introduction to Statistics and Econometrics April 16,2020 11/167
Introduction to Sampling Theory Introduction to Sampling Theory Introduction to Statistics and Econometrics April 16, 2020 11/167 Population and Random Sample Population and Random Sample
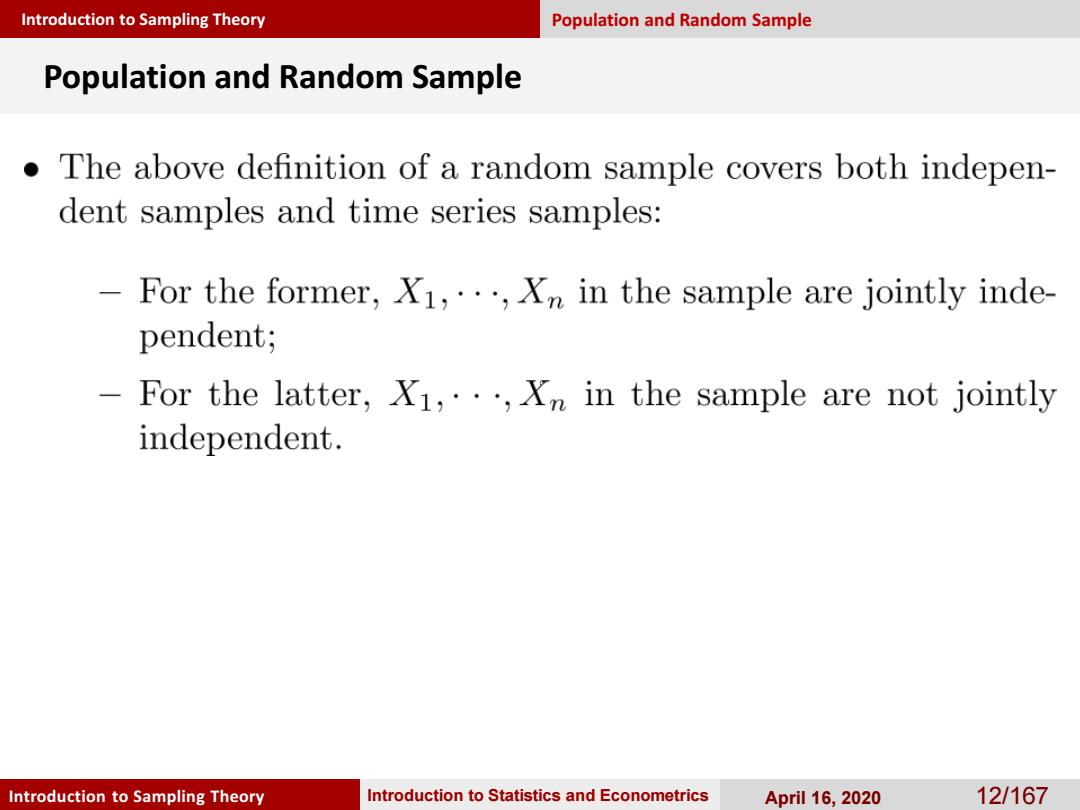
Introduction to Sampling Theory Population and Random Sample Population and Random Sample The above definition of a random sample covers both indepen- dent samples and time series samples: For the former,X1,...,Xn in the sample are jointly inde- pendent; For the latter,X1,...,Xn in the sample are not jointly independent. Introduction to Sampling Theory Introduction to Statistics and Econometrics April 16,2020 12/167
Introduction to Sampling Theory Introduction to Sampling Theory Introduction to Statistics and Econometrics April 16, 2020 12/167 Population and Random Sample Population and Random Sample
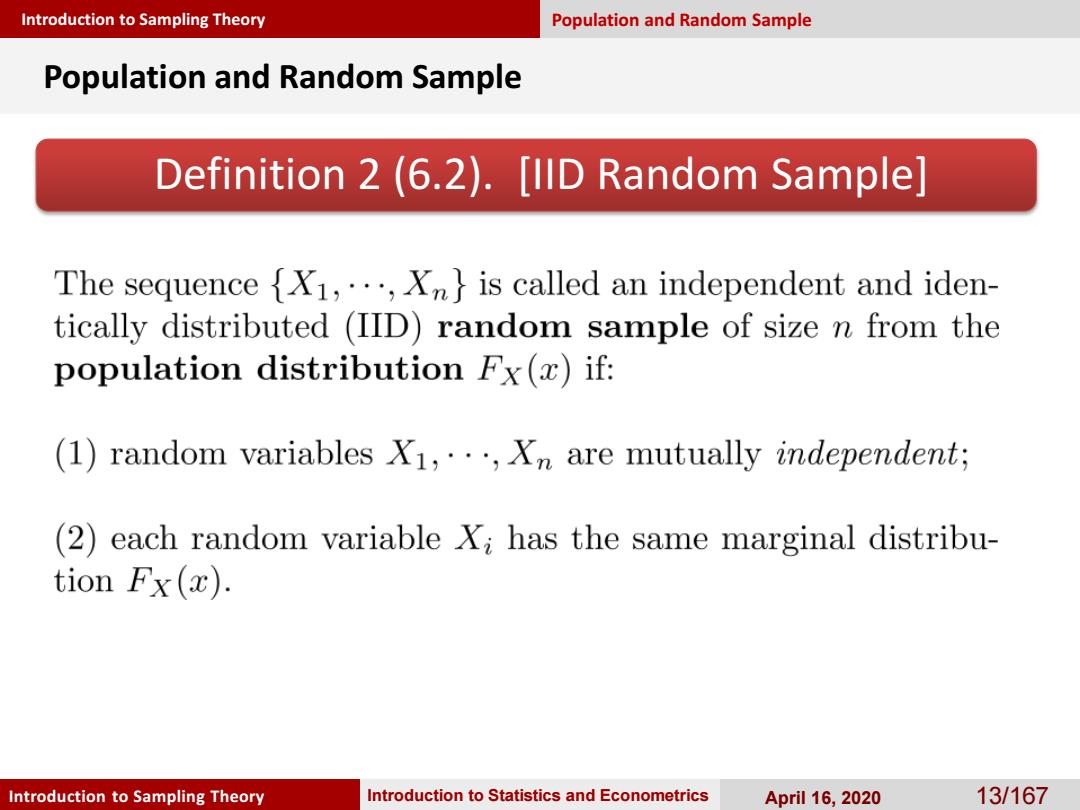
Introduction to Sampling Theory Population and Random Sample Population and Random Sample Definition 2(6.2).[lID Random Sample] The sequence {X1,..,Xn}is called an independent and iden- tically distributed (IID)random sample of size n from the population distribution Fx(x)if: (1)random variables X1,..,Xn are mutually independent; (2)each random variable Xi has the same marginal distribu- tion Fx(x). Introduction to Sampling Theory Introduction to Statistics and Econometrics April 16,2020 13/167
Introduction to Sampling Theory Introduction to Sampling Theory Introduction to Statistics and Econometrics April 16, 2020 13/167 Definition 2 (6.2). [IID Random Sample] Population and Random Sample Population and Random Sample

Introduction to Sampling Theory Population and Random Sample Population and Random Sample Question:What is the interpretation and implication of an IID random sample? Suppose we have a random experiment in which the vari- able of interest X has a probability distribution Fx(x). Suppose the random experiment is repeated n times. Then we observe n outcomes for the variable of inter- est,denoted as xm =(1,...,n). Introduction to Sampling Theory Introduction to Statistics and Econometrics April 16,2020 14/167
Introduction to Sampling Theory Introduction to Sampling Theory Introduction to Statistics and Econometrics April 16, 2020 14/167 Population and Random Sample Population and Random Sample

Introduction to Sampling Theory Population and Random Sample Population and Random Sample Question:What is the interpretation and implication of an IID random sample? If we denote Xi as the variable of interest associated with the i-th experiment,then Xi has the probability distribution Fx(x)and xi can be viewed as a realization of Xi. Identical distribution for the Xi means repeated ex- periments of same kind,and independence means that experiments are implemented independently so that new information can be obtained from each experiment. Introduction to Sampling Theory Introduction to Statistics and Econometrics April 16,2020 15/167
Introduction to Sampling Theory Introduction to Sampling Theory Introduction to Statistics and Econometrics April 16, 2020 15/167 Population and Random Sample Population and Random Sample