
Coupled-Mode Theory HERMANN A.HAUS AND WEIPING HUANG,MEMBER,IEEE Invited Paper The principal features of coupled mode theory are reviewed and fiber optics in recent years are described.We then con- in particular as applied to passive structures,such as coupled sider lossless coupling of two modes in time.Two coupled resonators and coupled waveguides.The former are examples of coupling of modes in time,the latter are examples of coupling of resonance circuits,or two coupled microwave or optical modes in space.Active structures are considered briefly insofar resonators,are the physical examples.Energy conservation as they obey conservation laws in terms of positive and negative requires that the eigenfrequencies be real.The start-up of a energies.The complications caused by nonorthogonality of the parametric oscillator is another example.Then we look at mode energies,or powers,are brought up.These were the topic of recent publications. the formal derivation of coupled mode theory and consider The coupling of modes formalism has intuitive appeal in that the the more general case when the modes are not energy- coupling equations in their simplest form can be written down by orthogonal and the energies are not necessarily positive. inspection.When "fine points"as,for instance,cross talk are at A more detailed account of the nonorthogonal coupled issue,then more formal derivations are required.The one presented mode theory developed in the last five years for optical here is based on a variational principle. waveguides is given.When one resonator stores negative energy,the other positive energy,the frequencies appear in I.INTRODUCTION complex-conjugate pairs.A resonator containing an active In this paper we review the principal features of coupled medium,the latter treated in a linearized approximation,is mode theory.The literature on the subject is vast and it an example. would be difficult to do justice to all aspects of coupled This study is followed up by showing how the coupled mode theory.When the first author,H.A.Haus,was asked mode formalism can be derived from a variational principle to contribute this invited paper,he accepted on the grounds for the frequencies of the system.If a trial solution is that aspects of coupled mode theory had intrigued him introduced for the electric field in a lossless electromagnetic throughout his career,his first papers on the subject having system that is the linear superposition of modes,the coupled been written in the 1950's.Recent work done with W.P. mode formalism is the result.We do not consider the formal Huang,one of his doctorate students,prompted him to ask derivation for more complicated,active systems,they can him to collaborate on this invited paper.Hence the present be found in the literature.Indeed,the very virtue of the cou- paper is an overview of coupled mode theory through the pling of modes formalism lies in its intuitive appeal.Much “lenses”of two“afficionados'of coupled mode theory.The physical insight can be obtained by inspection,without the review is not intended to be exhaustive due to the imposed need for a formal derivation limit on the length of the paper.It represents the view of Next,we consider coupling of modes in space.Here the the authors based on their own experience and knowledge initial conditions in time,relevant to coupling of modes in We first give a brief historic perspective of the coupled time,are replaced by boundary conditions in space.The mode theory.The development and applications of the sign of the group velocity of a wave indicates at which theory in microwaves in early years and in optoelectronics "end"of a structure a wave is excited.The sign of its energy indicates whether it is passive (positive energy)or active Manuscript received August 2,1990.revised December 28.1990.This (negative energy).On the basis of such simple arguments work was supported in part by the National Science Foundation under one may distinguish the operation of a Bragg reflector, Grant EET8700474 and by the Joint Services Electronics Program under Contract DAAL03-89-C-0001. traveling wave tube,or backward wave oscillator H.A.Haus is with the Department of Electrical Engineering and The formal derivation of the coupled mode formalism in Computer Science and Research Laboratory of Electronics,Cambridge, space is again restricted to the case of passive electromag- MA02139. W.P.Huang is with the Department of Electrical Engineering,Univer. netic structures.We show how the formalism results from sity of Waterloo,Waterloo,OntCanada. a variational principle.The variational principle provides a IEEE Log Number 9103551. refined method for the evaluation of coupling coefficients, 0018-9219/91/01.00©1991IEEE PROCEEDINGS OF THE IEEE,VOL 79,NO.10,OCTOBER 1991 1505

some aspects of which are easily missed by the intuitive then the best value obtainable for the propagation constant approach.The refinements are necessary for an understand- results from the coupled mode equations ing of cross talk in optical waveguide couplers.It was in The coupled mode theory for optical waveguides was this context that the need for a more formal derivation of developed by Marcuse [12],[13],Snyder [14],[15],Yariv coupled mode theory reasserted itself. and Taylor [161,[17],and Kogelnik [18],[19]in the early 1970's.It has been successfully applied to the modeling and analysis of various guided-wave optoelectronic and fiber II.HISTORICAL PERSPECTIVE optical devices,such as optical directional couplers made The concept of coupled modes in electromagnetics may of thin film and channel waveguides [20]-[23]and optical be traced back to the early 1950's.The application was fibers [24]-[28],multiple waveguide lenses [29],[30], initially to microwaves and developed gradually through phase-locked laser arrays [31],distributed feedback lasers the contributions of many people.In 1954,Pierce applied [31]-[37]and distributed Bragg reflectors (38]-[40],grating the coupled mode theory to the analysis of microwave waveguides and couplers [41]-[45],nonparallel and tapered traveling-wave tubes [1].Later followed the work of Gould waveguiding structures [46]-[55],Y-branch waveguides [2]on the backward-wave oscillators.The coupled mode 56]-[58],TE/TM polarization converters [59]-(63],polar- theory was then employed to treat parametric amplifiers, ization rotation in optical fibers [64]-(66],mode conversion oscillators,and frequency converters [3]. and radiation loss in slab waveguides and fibers [67]-(71], A parallel development happened in microwave waveg- residual coupling among scalar modes [72].[73].It has uides and devices.Miller [4]first introduced the coupled also been used to study the wave coupling phenomena mode theory to the analysis and design of microwave in nonlinear media such as harmonic generation in bulk waveguides and passive devices.The theory was soon 74]and guided-wave devices [75],[76],nonlinear pulse or generalized by Louisell [5]to treat tapered waveguide soliton propagation[77],[78]and the modulation instability structures,where the coupling coefficients depend on the [79]in optical fibers,and nonlinear coherent couplers length a.In the 1960's,the coupled mode theory was further [80]-[86.Many of these applications are well documented developed to describe mode conversions due to various and summarized in [87]-(93]. irregularities in microwave waveguides [6],[7]and periodic An assumption made in the conventional coupled mode waveguide structures [8],[9]. theory is that the modes of the uncoupled systems are The early approach to the coupled mode theory was orthogonal to each other.This may be true if the modes rather heuristic.The modes of the "uncoupled systems" belong to the same reference structures.In studying the were identified and the coupled mode equations obeyed mode coupling in coupled systems,however,one often by the mode amplitudes were determined from power chooses the modes of the isolated systems as the basis considerations. for the mode expansion and these modes may not be A rigorous derivation of coupled mode theory was car- orthogonal.The orthogonal coupled mode theory (OCMT) ried out by Schelkunoff (10].By expanding the unknown is not correct for the description of the mode-coupling electromagnetic fields of a coupled system in terms of the process in this case.The effect of nonorthogonality between known modes of an uncoupled system,he obtained a set waveguide modes on cross talk in optical couplers was first of generalized telegraphist's equations (a different version recognized by Chen and Wang [94]and then studied by of the coupled mode equations)directly from Maxwell's Haus and Whitaker [95]in a proposal to eliminate the equations.The coupling coefficients are determined unam- cross talk due to this effect.Later on,several formula- biguously once the modes of the uncoupled system are tions of the nonorthogonal coupled mode theory (NCMT) defined.The coupled mode equations are equivalent to were developed by Hardy and Streifer [96],Haus,Huang. Maxwell's equations as long as a complete set is assumed Kawakami,and Whitaker [97],and Chuang [98].The new for the mode expansion.For most applications,however, nonorthogonal coupled mode theory (NCMT)is shown only a limited number of modes (usually two)is used to yield more accurate dispersion characteristics and field in the expansion.Therefore,the coupled mode theory patterns for the modes of the coupled waveguides.It also remains an approximate,yet insightful and often accurate calls for a modification of the description of the power mathematical description of electromagnetic oscillation and exchange between the waveguides. wave propagation in a coupled system.In order to put the There were some discrepancies among the different for- approach on a more formal mathematical footing,one of mulations at the early stage of the development.Some were the authors (HAH)showed in 1958 that the coupled mode superficial and soon resolved by reformulation [99];some theory was derivable from a variational principle set up are more subtle [97],[99]-101].Snyder,Ankiewicz,and for the propagation constant of the coupled system [111. Altintasl [102]showed that the nonorthogonal formulations Because of the stationary nature of the variational principle, could lead to erroneous results for the coupling length of the the errors made in an incomplete expansion do not lead to TM modes of parallel slabs when the index discontinuity a dramatic deterioration of the accuracy for the propagation is large.The origin of the error is apparent in this case constants calculated from the coupled mode theory.If one since the waveguide modes used as the trial solution in decides to approximate the fields of the coupled system as a the coupled mode theory are subject to serious error when linear superposition of the fields of the uncoupled systems, the index steps are large.But they also demonstrated in PROCEEDINGS OF THE IEEE,VOL 79,NO.10,OCTOBER 1991

the same example that the conventional orthogonal coupled is important only when ww2.Then,the derivative of mode theory based on the same trial solution gives excellent a2 is approximately equal to jw x a2,its integral equal prediction about the coupling length.This unexpected result to 1/jw2 x a2.In the coupling term of (2.3),the coupling triggered a series of debates in the field [103]-[110].It was coefficient is small compared with both w and w2,and later resolved by Haus,Huang,and Snyder [111]. thus the replacement of the derivative or integral by its Despite the controversies,there have been intense re- approximate values causes an error of higher order in the search activities in the past few years in developing and coupling and can be ignored.This is why the coupling of applying the nonorthogonal coupled mode theory in the modes formalism can be set up so simply in the limit of area of optoelectronics and fiber optics.Simplified scalar weak coupling versions that may be applied to weakly guiding struc tures [112][115]and a modified vector version for the A.Coupling of Energy Orthogonal Modes of Positive Energy strongly guided structures [111],[115]were developed. Generalizations of the theory to multiwaveguide and/or We think of energy,generally,as a positive quantity.This multimode structures [116]-[121],anisotropic media [122] is the case we shall address first.There are many perfectly [123],periodic and tapered structures [124]-[128],and non realistic situations in which the energy must be considered linear couplers [129]were attempted.The nonorthogonal negative.We shall come back to such cases later on. coupled mode theory has been employed in the analysis and We concentrate first on energy orthogonal modes for design of optical guided-wave devices [130]-[138]and fiber which the energy can be written optical couplers [139]-(146].The experimental verification of the theory was carried out by Marcatili [147]and Syms W=la12+a22 (2.5) [148}-[150] even in the presence of the coupling.Here we have nor- malized the electric field amplitudes a1 and a2 so that III.COUPLING OF MODES IN TIME their squares are equal to the energies in the modes.No We shall first address coupling of modes in time starting cross terms are assumed to exist,the modes are energy with a few intuitively obvious postulates.From these we orthogonal.If the coupling is lossless,the only case we can develop a formalism that describes coupling of two shall treat here,energy must be conserved: resonators,parametric amplification and oscillation.Then we shall show how the coupling of modes of passive d electromagnetic structures can be derived from a varia- (l+laa)=jmajaz+jaa tional principle.This variational principle provides rules for -jki2a1a2-jK21a2a1=0(2.6) the evaluation of the coupling coefficients when intuitive arguments are not sufficiently precise to provide these where we have used (2.3)and (2.4).Because the initial coefficients. conditions can be picked arbitrarily,(2.6)can only be Consider two weakly coupled lossless resonators.Denote obeyed when the amplitude with the time dependence exp(jwit)in one resonator by a1,the amplitude in the other resonator K12=K21=K (2.7 with the time dependence exp(jwat)by a2.These are the positive frequency components of the electric field This is the constraint on the coupling coefficients of two amplitudes.They obey the differential equations: modes coupled in a lossfree way.When (2.7)is intro- duced into (2.3)and(2.4),a time dependence of the form dai dt jw1a1 (2.1) exp(jwt)is assumed and the determinantal equation is solved one finds the two roots for w: da2 =1w202. (2.2) w1+w2 w= W1-W2 When the two resonators are coupled,their time dependence +2 (2.8) 2 changes.When the coupling is weak,it has to be of the form: The two frequencies are real,as they must be when two day modes of positive energy couple and energy is to be dt =jw1a1+jK1202 (2.3) conserved.Figure 1 shows the roots ws (lower curve) daz and wa(upper curve)of the symmetric and antisymmetric dt jw2a2 jK21a1 (2.4) normal modes for the case when one of the resonance frequencies (w)is changed,the other is kept fixed.This is At first it may not be obvious why the coupling should the kind of diagram familiar also from quantum mechanical be proportional to the amplitude of the other resonator, perturbation theory.When an energy level crossing occurs rather than to the time derivative,or the time integral of in a coupled system,the "eigenvalues"split,crossing is the amplitude,or any complicated operator operating on prevented.At the crossover point,for real the solutions the amplitude.However,if the coupling is weak,the time are the symmetric and antisymmetric combinations of a dependence is perturbed only weakly and the coupling term and a2.Farther from the crossover point the solutions HAUS AND HUANG:COUPLED-MODE THEORY 1507

1 、+。 (3 2 28 。+61 Fig.1.The resonance frequencies of the symmetric (lower curve) and antisymmetric(upper curve)mode of a coupled resonator as a function of detuning 1. acquire the character of the mode to whose frequency the eigenvalue is the closest. It is of interest to ask for the implications of this simple coupled mode formalism.Consider first the case when the two resonators have equal frequencies.Then,if resonator 1 is excited at t=0,a=1,and resonator 2 is unexcited,a2 =0,the initial conditions are matched by a superposition of equal amounts of the symmetric and antisymmetric solutions.The phases of the two solutions evolve at different rates and after the time t=/2 all of the excitation will have been transferred to the other resonator.The excitation oscillates back and forth between the two resonators.When the frequencies of the Fig.2.Coupled metallic rectangular waveguide resonators that permit exact analysis. two uncoupled resonators are not equal,and initially only one resonator is excited,the transfer is not complete. by setting B.A Simple Example 1 Figure 2 shows a simple example that permits both a rigorous analysis and yields easily to the coupled mode for- malism.In this way one may compare the exact answer with We use energy arguments to derive the coupling.Consider the approximate solution.The two resonators are metallic the time rate of change of the energy in mode (1): rectangular waveguides partially filled with a dielectric so d that the empty waveguides are below cutoff at the resonance a1P=jN12aia2-jxza1o时. (2.13) frequency.The uncoupled waveguides are each terminated in infinite air-filled waveguides.The question is as to how This energy change must be equal to the power fed into one may find the coupling coefficient for this structure,and mode (1)by mode(2).Mode(2)finds the perturbation of thus the value of the beat frequency of the two eigenmodes. dielectric constant e within resonator (1)and drives a Denote the spatial distribution of the dielectric constant that polarization current density through that perturbation that forms cavity (1)by is equal to Eo +6E1 2.9) jwP12 jwde1a2e2 (2.14) and the one that forms resonator (2)by The power fed into mode (1)by this current density is Eo +662. (2.10) equal to The actual distribution is 1 4 juwP12·ajeidv+c.c. e=e。+6e1+6e2, (2.11) =jk12aja2-jki2a1a2 Denote the electric field patterns of modes(1)and(2)by 1 er and e2,respectively.The energy in mode (1)is 4 jwbeie2ejdvaja2 +c.c.(2.15) cleil2dv a12 (2.12) and must be responsible for the rate of growth in time of the energy in resonator (1)due to the presence of mode (2) 1508 PROCEEDINGS OF THE IEEE,VOL 79,NO.10.OCTOBER 1991
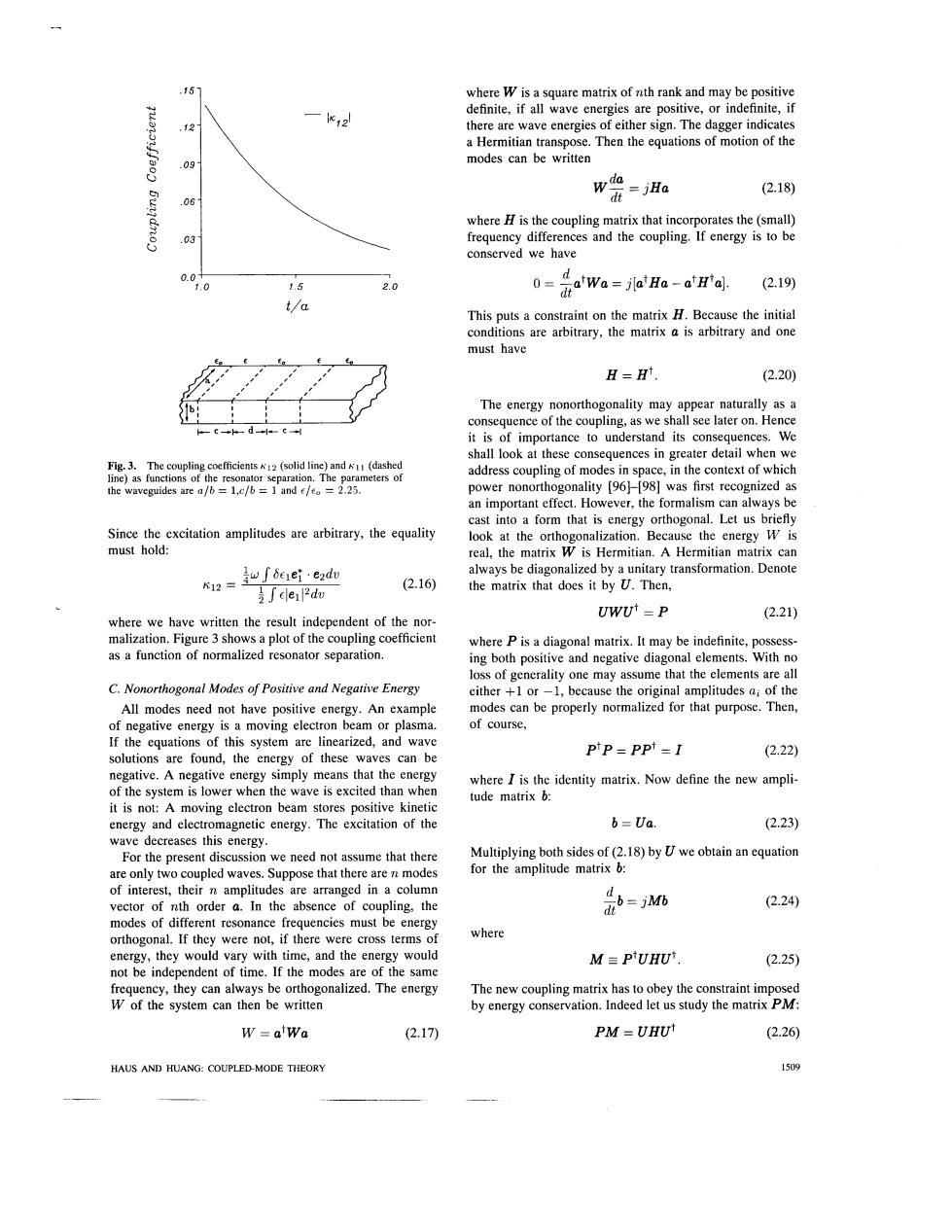
15 where W is a square matrix of nth rank and may be positive 一k12 definite,if all wave energies are positive,or indefinite,if there are wave energies of either sign.The dagger indicates a Hermitian transpose.Then the equations of motion of the .09 modes can be written 形6 dt jHa (2.18) .06 where H is the coupling matrix that incorporates the (small) 03 frequency differences and the coupling.If energy is to be conserved we have 0.0 .0 1.5 2.0 0- da'Wa=jla'Ha-a'H'al. (2.19) d t/a This puts a constraint on the matrix H.Because the initial conditions are arbitrary,the matrix a is arbitrary and one must have H=H (2.20) The energy nonorthogonality may appear naturally as a consequence of the coupling,as we shall see later on.Hence c-+h d+-c+ it is of importance to understand its consequences.We shall look at these consequences in greater detail when we Fig.3.The coupling coefficients12 (solid line)and (dashed line)as functions of the resonator separation.The parameters of address coupling of modes in space,in the context of which the waveguides are a/b 1,c/b=1 and e/o 2.25. power nonorthogonality [96]-98]was first recognized as an important effect.However,the formalism can always be cast into a form that is energy orthogonal.Let us briefly Since the excitation amplitudes are arbitrary,the equality look at the orthogonalization.Because the energy W is must hold: real,the matrix W is Hermitian.A Hermitian matrix can w∫6e1ei·e2du always be diagonalized by a unitary transformation.Denote K12= (2.16) 支∫cel2do the matrix that does it by U.Then, UWUt=P (2.21) where we have written the result independent of the nor- malization.Figure 3 shows a plot of the coupling coefficient where P is a diagonal matrix.It may be indefinite,possess- as a function of normalized resonator separation ing both positive and negative diagonal elements.With no loss of generality one may assume that the elements are all C.Nonorthogonal Modes of Positive and Negative Energy either +1 or-1,because the original amplitudes a:of the All modes need not have positive energy.An example modes can be properly normalized for that purpose.Then, of negative energy is a moving electron beam or plasma of course, If the equations of this system are linearized,and wave P'P=PP=I solutions are found,the energy of these waves can be (2.22) negative.A negative energy simply means that the energy where I is the identity matrix.Now define the new ampli- of the system is lower when the wave is excited than when tude matrix b: it is not:A moving electron beam stores positive kinetic energy and electromagnetic energy.The excitation of the b=Ua. (2.23) wave decreases this energy. For the present discussion we need not assume that there Multiplying both sides of(2.18)by U we obtain an equation are only two coupled waves.Suppose that there are n modes for the amplitude matrix b: of interest,their n amplitudes are arranged in a column vector of nth order a.In the absence of coupling,the aib=jMb (2.24) modes of different resonance frequencies must be energy orthogonal.If they were not,if there were cross terms of where energy,they would vary with time,and the energy would M=P'UHUT (2.25) not be independent of time.If the modes are of the same frequency,they can always be orthogonalized.The energy The new coupling matrix has to obey the constraint imposed W of the system can then be written by energy conservation.Indeed let us study the matrix PM: W=aWa (2.17) PM =UHU (2.26) HAUS AND HUANG:COUPLED-MODE THEORY 1509