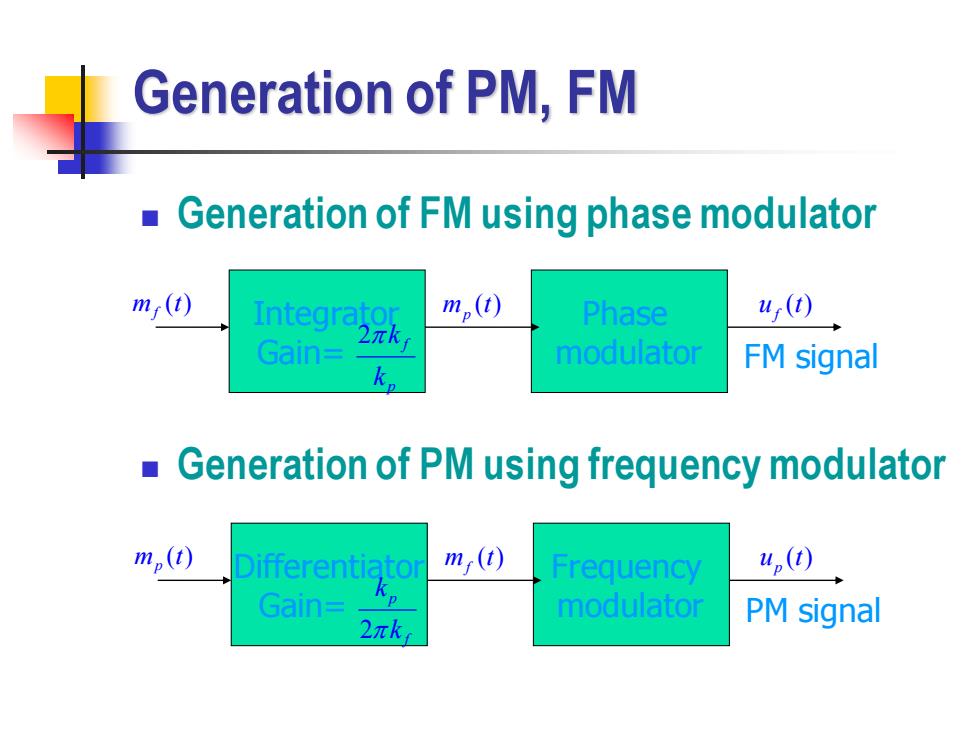
Generation of PM,FM Generation of FM using phase modulator mr(t) Integrator m (t) Phase u,(t) Gain与 modulator FM signal ■ Generation of PM using frequency modulator m,(t) Differentiator m,(t) Frequency u,(t) Gain= modulator 2nk PM signal
Generation of PM, FM ◼ Generation of FM using phase modulator ◼ Generation of PM using frequency modulator Integrator Gain= 2 f p k k Phase modulator ( ) m t f ( ) m t p ( ) f u t FM signal Differentiator Gain= 2 p f k k Frequency modulator ( ) m t p ( ) m t f ( ) p u t PM signal

PM and FM in Frequency domain ■Very complex Due to the nonlinearity For analysis,assume m(t)is sinusoidal ·m,())=acos(2πfmf),m,(t)=-asin(2πfm) Then,the modulated signal u,(t)=A.cos{2πft+k,acos(2πfmt)}=Acos{2πft+B,cos(2πfmt)} 马0=cs2zu+艺co2x}=A2/+B,ea20州》
PM and FM in Frequency domain ◼ Very complex ◼ Due to the nonlinearity ◼ For analysis, assume m(t) is sinusoidal ◼ ◼ Then, the modulated signal ( ) cos(2 ), ( ) sin(2 ) m t a f t m t a f t p m f m = = − ( ) cos{2 cos(2 )} cos{2 cos(2 )} ( ) cos{2 cos(2 )} cos{2 cos(2 )} p c c p m c c p m f f c c m c c f m m u t A f t k a f t A f t f t k a u t A f t f t A f t f t f = + = + = + = +

Modulation index of PM and FM ■For sinusoidal m(t) ■Phase modulation index:p。=kna Peak phase deviation More phase shift for a large magnitude a k a Frequency modulation index:A,= Peak frequency deviation More phase shift for al large magnitude a and small frequency fm In general,for non-sinusoidal m(t) ■B。=k max m(t)l),p,= k,max m(t) W W is Bandwidth of m(t)
Modulation index of PM and FM ◼ For sinusoidal m(t) ◼ Phase modulation index : ◼ Peak phase deviation ◼ More phase shift for a large magnitude a ◼ Frequency modulation index: ◼ Peak frequency deviation ◼ More phase shift for al large magnitude a and small frequency fm ◼ In general, for non-sinusoidal m(t) ◼ ◼ W is Bandwidth of m(t) p p = k a max ( ) max ( ) , f p p f k m t k m t W = = f f m k a f =