
2013-3-6 Chapter 12 Ideal Gas Mixture and Psychrometric Applications Learning Outcomes Describe ideal gas mixture composition in terms of mass fractions and mole fractions. Use the Dalton model to relate pressure,volume, and temperature and to calculate changes in U,H, and S for ideal gas mixtures Apply mass,energy,and entropy balances to systems involving ideal gas mixtures,including mixing processes. 1
2013-3-6 1 Chapter 12 Ideal Gas Mixture and Psychrometric Applications Learning Outcomes ►Describe ideal gas mixture composition in terms of mass fractions and mole fractions. ►Use the Dalton model to relate pressure, volume, and temperature and to calculate changes in U, H, and S for ideal gas mixtures. ►Apply mass, energy, and entropy balances to systems involving ideal gas mixtures, including mixing processes
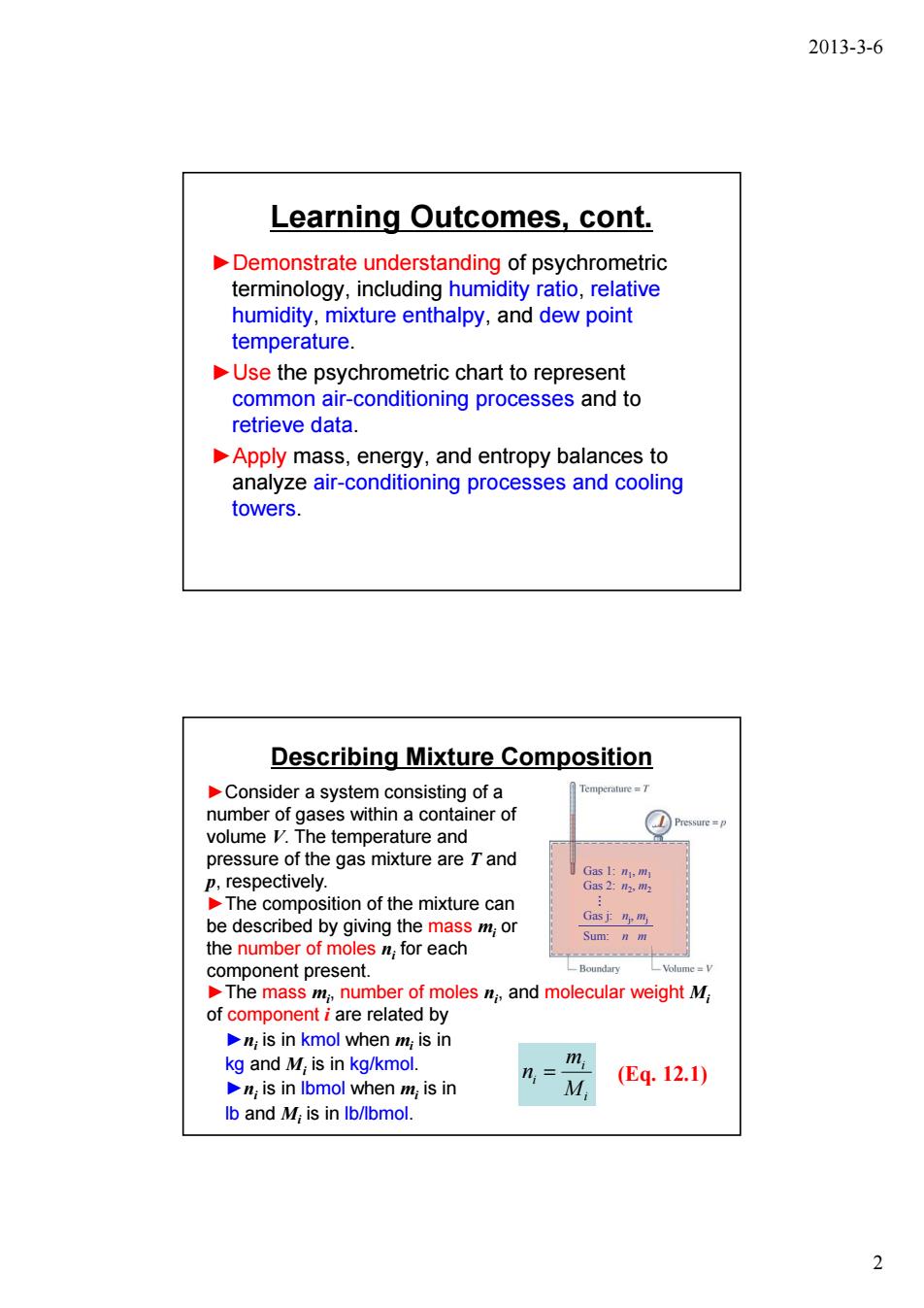
2013-3-6 Learning Outcomes,cont. Demonstrate understanding of psychrometric terminology,including humidity ratio,relative humidity,mixture enthalpy,and dew point temperature. Use the psychrometric chart to represent common air-conditioning processes and to retrieve data. Apply mass,energy,and entropy balances to analyze air-conditioning processes and cooling towers. Describing Mixture Composition Consider a system consisting of a number of gases within a container of volume V.The te e perature and pressure of the gas mixture areand mixture can he des the numb by giving for each s m;or present ber of moles and molecular weightM e related by is in kmol when m;is in kg and M,is in kg/kmol. is in lbmol when m;is in (Eq.12.1) lb and M,is in Ib/lbmol. 2
2013-3-6 2 Learning Outcomes, cont. ►Demonstrate understanding of psychrometric terminology, including humidity ratio, relative humidity, mixture enthalpy, and dew point temperature. ►Use the psychrometric chart to represent common air-conditioning processes and to retrieve data. ►Apply mass, energy, and entropy balances to analyze air-conditioning processes and cooling towers. Describing Mixture Composition (Eq. 12.1) ►The mass mi , number of moles ni , and molecular weight Mi of component i are related by i i i M m n = ►Consider a system consisting of a number of gases within a container of volume V. The temperature and pressure of the gas mixture are T and p, respectively. ►The composition of the mixture can be described by giving the mass mi or the number of moles ni for each component present. ►ni is in kmol when mi is in kg and Mi is in kg/kmol. ►ni is in lbmol when mi is in lb and Mi is in lb/lbmol. Gas 1: n1, m1 Gas 2: n2, m2 Gas j: nj , mj Sum: n m …

2013-3-6 Describing Mixture Composition The mass fraction is the relative amount of each component in the mixture.The mass fractionf;of component i is n时= (Eq.12.3) m where m is the total mass of mixture The sum of the mass fractions of all components in a mixture equals unity. Describing Mixture Composition Alternatively,the mole fraction can be used to describe the relative amount of each component in the mixture.The 丹 mole fractiony;of component i is (Eq.12.6) where n is the total moles of mixture The sum of the mole fractions of all components in a mixture equals unity. The apparent (or average)molecular weight M of a mixture is determined as M=yM a mole-fraction average of the 1 component molecular weights: (Eq.12.9) 3
2013-3-6 3 Describing Mixture Composition ►The mass fraction is the relative amount of each component in the mixture. The mass fraction mfi of component i is (Eq. 12.3) m m mf i i = ►The sum of the mass fractions of all components in a mixture equals unity. where m is the total mass of mixture. Describing Mixture Composition ►Alternatively, the mole fraction can be used to describe the relative amount of each component in the mixture. The mole fraction yi of component i is n n y i i = (Eq. 12.6) i j i M ∑ yi M = = 1 (Eq. 12.9) ►The sum of the mole fractions of all components in a mixture equals unity. ►The apparent (or average) molecular weight M of a mixture is determined as a mole-fraction average of the component molecular weights: where n is the total moles of mixture

2013-3-6 Describing Mixture Composition Example:The molar analysis of a gas mixture is 50%N2,35%CO2,and 15%02.Determine (a)the apparent molecular weight of the mixture and (b)the analysis in terms of mass fractions. Solution: (a)The apparent molecular weight of the mixture is found using Eq.12.9 and molecular weights (rounded)from Table A-1 M=0.50(28)+0.35(44)+0.1532)=34.2kg/kmol Describing Mixture Composition ent,in kmol,is equal to its mole fraction,as shown in column (ii). Column(i)lists the respective molecular weights. Column (iv)gives the mass of each component,in kg per kmole of mixture,obtained using=nM,(Eq.12.1). hemasactionsnlsedaspercenagesncohecnatioial e values in column(iv)by the column 0.50 14 40.94 CO, 025 15.4 45.03 0.15 1404 4
2013-3-6 4 Describing Mixture Composition Example: The molar analysis of a gas mixture is 50% N2, 35% CO2, and 15% O2. Determine (a) the apparent molecular weight of the mixture and (b) the analysis in terms of mass fractions. (a) The apparent molecular weight of the mixture is found using Eq. 12.9 and molecular weights (rounded) from Table A-1 M = 0.50( ) 28 + 0.35(44)+ 0.15(32) = 34.2 kg/kmol Solution: Describing Mixture Composition ►Then, the amount ni of each component, in kmol, is equal to its mole fraction, as shown in column (ii). ►Column (iii) lists the respective molecular weights. ►Column (iv) gives the mass mi of each component, in kg per kmole of mixture, obtained using mi = ni Mi (Eq. 12.1). ►The mass fractions, listed as percentages in column (v), are obtained by dividing the values in column (iv) by the column total and multiplying by 100. (i) Component (ii) ni × (iii) Mi = (iv) mi (v) mfi % N2 0.50 × 28 = 14 40.94 CO2 0.35 × 44 = 15.4 45.03 O2 0.15 × 32 = 4.8 14.04 1.00 34.2 100 (b) Although the actual amount of mixture is not known, the calculations can be based on any convenient amount. We use 1 kmol of mixture
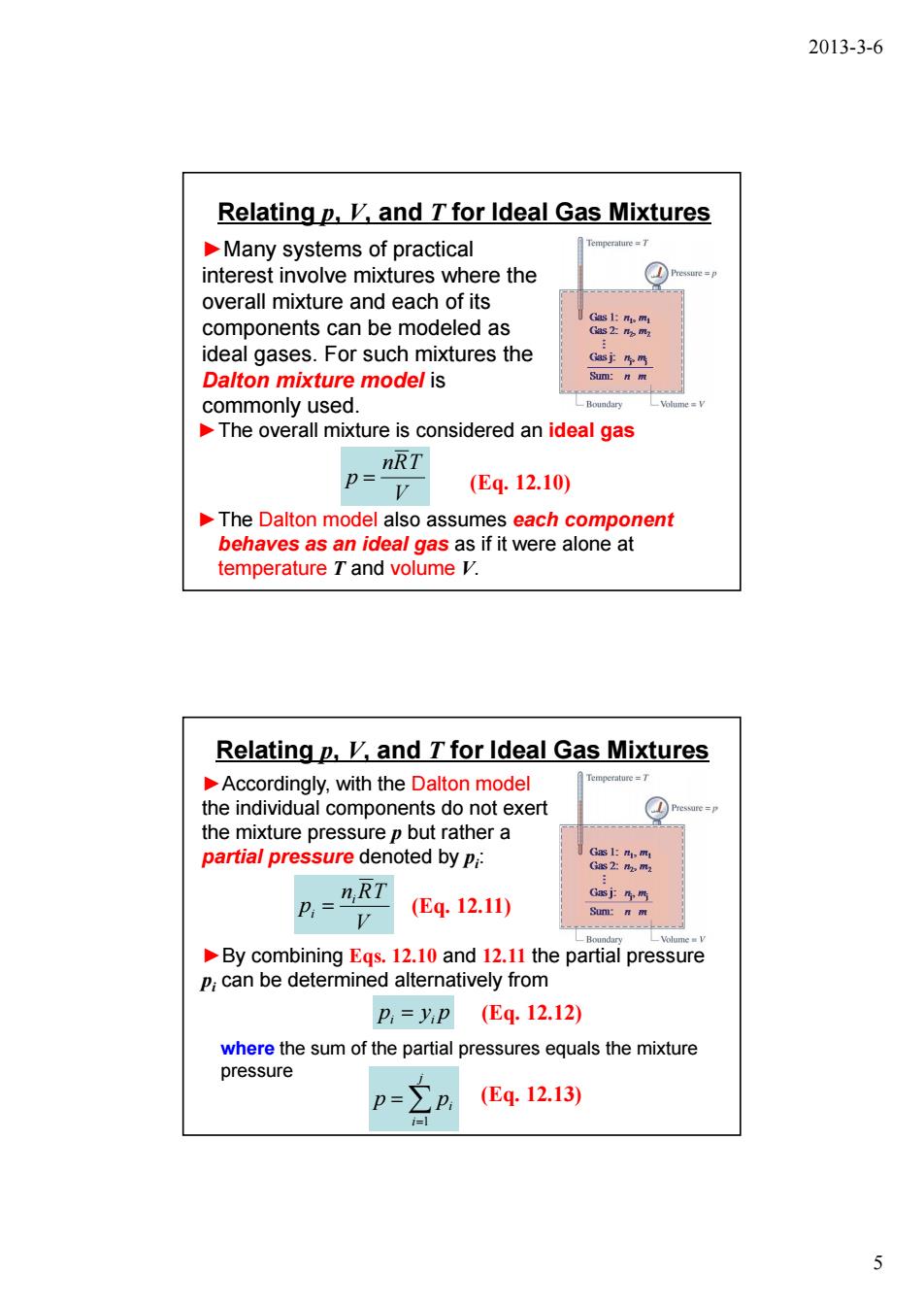
2013-3-6 Relatingp,K,and T for Ideal Gas Mixtures Many systems of practical interest involve mixtures where the overall mixture and each of its components can be modeled as ideal gases.For such mixtures the Dalton mixture model is commonly used. The overall mixture is considered an ideal gas P=IRT V (E4.12.10) The Dalton model also assumes each component behaves as an ideal gas as if it were alone at temperature T and volume V. Relating p,K,and T for Ideal Gas Mixtures Accordingly,with the Dalton model the individual components do not exert the mixture pressure p but rather a partial pressure denoted by Pi: (E4.12.11) V By combining Egs.12.10 and 12.11 the partial pressure P;can be determined alternatively from p,=yP(Eq.12.12) where the sum of the partial pressures equals the mixture pressure p=Ep (Eq.12.13) 5
2013-3-6 5 Relating p, V, and T for Ideal Gas Mixtures ►Many systems of practical interest involve mixtures where the overall mixture and each of its components can be modeled as ideal gases. For such mixtures the Dalton mixture model is commonly used. ►The overall mixture is considered an ideal gas V nRT p = (Eq. 12.10) ►The Dalton model also assumes each component behaves as an ideal gas as if it were alone at temperature T and volume V. Relating p, V, and T for Ideal Gas Mixtures ►Accordingly, with the Dalton model the individual components do not exert the mixture pressure p but rather a partial pressure denoted by pi : V n RT p i i = (Eq. 12.11) p y p i = i (Eq. 12.12) ►By combining Eqs. 12.10 and 12.11 the partial pressure pi can be determined alternatively from where the sum of the partial pressures equals the mixture pressure ∑ (Eq. 12.13) = = j i p pi 1