同济大学：《空气动力学》课程电子教案（课件讲稿）Chapter 3 Fundamentals of Inviscid Inviscid, Incompressible Flow

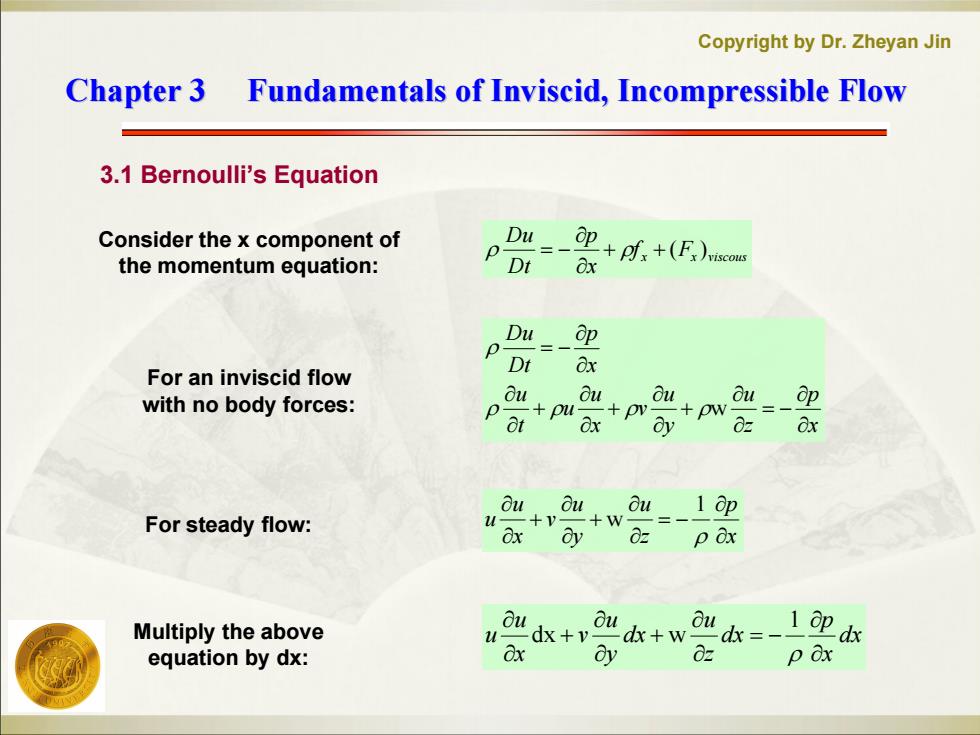
Copyright by Dr.Zheyan Jin Chapter 3 Fundamentals of Inviscid,Incompressible Flow 3.1 Bernoulli's Equation Consider the x component of the momentum equation: Du=_0p+pf,+(F.vw Dt dx Du=-卫 For an inviscid flow Dt dx with no body forces: p+pm0+pm0tv-卫 Ox dy du 1 ap For steady flow: p ox Multiply the above ou dx+v u ou dx+w dx = 1卫dk equation by dx: C D Ox

Copyright by Dr. Zheyan Jin Chapter 3 Fundamentals of Chapter 3 Fundamentals of Inviscid Inviscid, Incompressible Flow , Incompressible Flow 3.1 Bernoulli’s Equation For steady flow: Consider the x component of the momentum equation: x p z u y u v x u u              1 w x p z u y u v x u u t u x p Dt Du                       w  x Fx viscous f x p Dt Du   ( )       For an inviscid flow with no body forces: Multiply the above equation by dx: dx x p dx z u dx y u v x u u              1 dx w

Copyright by Dr.Zheyan Jin Chapter 3 Fundamentals of Inviscid,Incompressible Flow 3.1 Bernoulli's Equation Consider the streamline in three-dimensional space: wdy-vdz=0 udz-wdx=0 vdx-udy =0 ou dxu u ou dyu =-1pk We can further change Ox ay 0z pox this equation: ou dxy ou dy* db)=-12dh pOx The differential of u is: du= ou dx 8 ou dy aud也 oy dz The equation can be 1 written as: udu =-d(u2)=-- 1卫k 2 pox

Copyright by Dr. Zheyan Jin Chapter 3 Fundamentals of Chapter 3 Fundamentals of Inviscid Inviscid, Incompressible Flow , Incompressible Flow 3.1 Bernoulli’s Equation The differential of u is: Consider the streamline in three-dimensional space: dx x p dz z u dy y u dx x u u dx x p dz z u dy y u dx u x u u                           1 ( ) 1 u dz z u dy y u dx x u du          dx x p udu d u       1 ( ) 2 1 2 We can further change this equation: wdy  vdz  0 udz  wdx  0 vdx  udy  0 The equation can be written as :

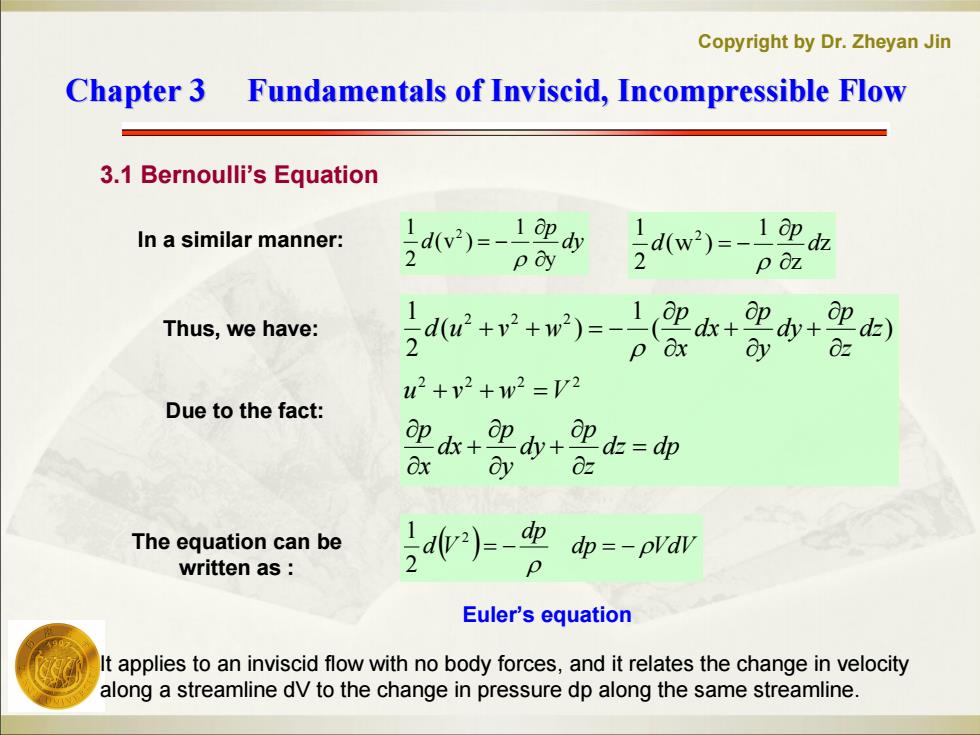
Copyright by Dr.Zheyan Jin Chapter 3 Fundamentals of Inviscid,Incompressible Flow 3.1 Bernoulli's Equation In a similar manner: 2w)=-12d w2)=-192d poy 2 P OZ 1 Thus,we have: w+2+w)=0++ Oy op d2) 2+v2+w2=V2 Due to the fact: p+吧d山+ 卫dk=dp The equation can be dp=-pVdv written as p Euler's equation It applies to an inviscid flow with no body forces,and it relates the change in velocity along a streamline dV to the change in pressure dp along the same streamline

Copyright by Dr. Zheyan Jin Chapter 3 Fundamentals of Chapter 3 Fundamentals of Inviscid Inviscid, Incompressible Flow , Incompressible Flow 3.1 Bernoulli’s Equation Due to the fact: In a similar manner:   dp VdV dp d V       2 2 1 dz dp z p dy y p dx x p u v w V dz z p dy y p dx x p d u v w                         2 2 2 2 2 2 2 ( ) 1 ( ) 2 1  Thus, w e have: The equation can be written as : dy p d y 1 (v ) 2 1 2      z z 1 ( w ) 2 1 2 d p d      Euler’s equation It applies to an inviscid flow with no body forces, and it relates the change in velocity along a streamline dV to the change in pressure dp along the same streamline

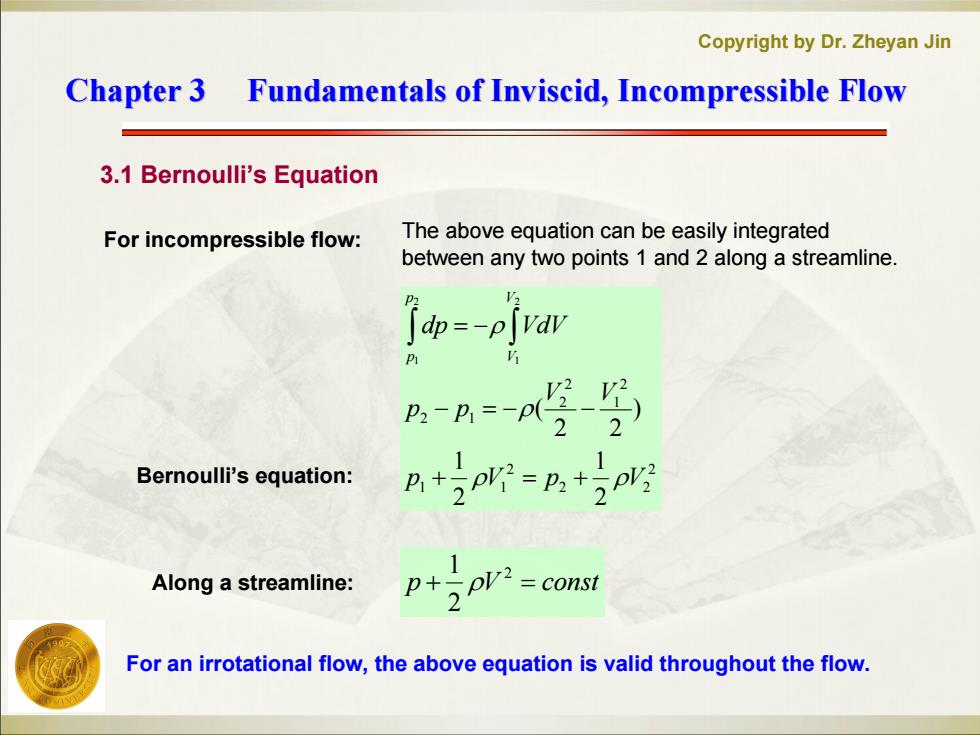
Copyright by Dr.Zheyan Jin Chapter 3 Fundamentals of Inviscid,Incompressible Flow 3.1 Bernoulli's Equation For incompressible flow: The above equation can be easily integrated between any two points 1 and 2 along a streamline. V2 Sdp=-p[vdv V2 P,-=-p( Bernoulli's equation: n+2p=n+p Along a streamline: =const For an irrotational flow,the above equation is valid throughout the flow

Copyright by Dr. Zheyan Jin Chapter 3 Fundamentals of Chapter 3 Fundamentals of Inviscid Inviscid, Incompressible Flow , Incompressible Flow 3.1 Bernoulli’s Equation Bernoulli’s equation: For incompressible flow: 2 2 2 2 1 1 2 1 2 2 2 1 2 1 2 1 ) 2 2 ( 2 1 2 1 p V p V V V p p dp VdV V V p p                Along a streamline: p  V  const 2 2 1  For an irrotational flow, the above equation is valid throughout the flow. The above equation can be easily integrated between any two points 1 and 2 along a streamline

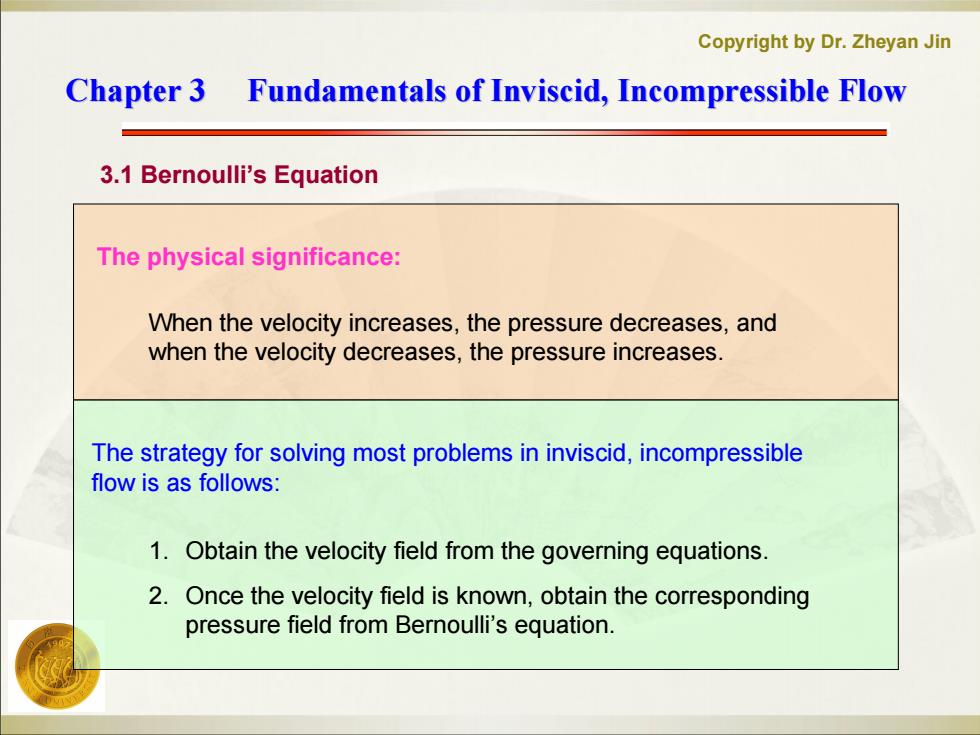
Copyright by Dr.Zheyan Jin Chapter 3 Fundamentals of Inviscid,Incompressible Flow 3.1 Bernoulli's Equation The physical significance: When the velocity increases,the pressure decreases,and when the velocity decreases,the pressure increases. The strategy for solving most problems in inviscid,incompressible flow is as follows: 1.Obtain the velocity field from the governing equations. 2.Once the velocity field is known,obtain the corresponding pressure field from Bernoulli's equation

Copyright by Dr. Zheyan Jin Chapter 3 Fundamentals of Chapter 3 Fundamentals of Inviscid Inviscid, Incompressible Flow , Incompressible Flow 3.1 Bernoulli’s Equation The strategy for solving most problems in inviscid, incompressible flow is as follows: The physical significance: 1. Obtain the velocity field from the governing equations. 2. Once the velocity field is known, obtain the corresponding pressure field from Bernoulli’s equation. When the velocity increases, the pressure decreases, and when the velocity decreases, the pressure increases