《实用统计软件》课程教学资源（阅读材料）图像合成方面应用的一个介绍 Monte Carlo Integration

Monte Carlo Integration COS 323 Acknowledgment:Tom Funkhouser

Monte Carlo Integration COS 323 Acknowledgment: Tom Funkhouser

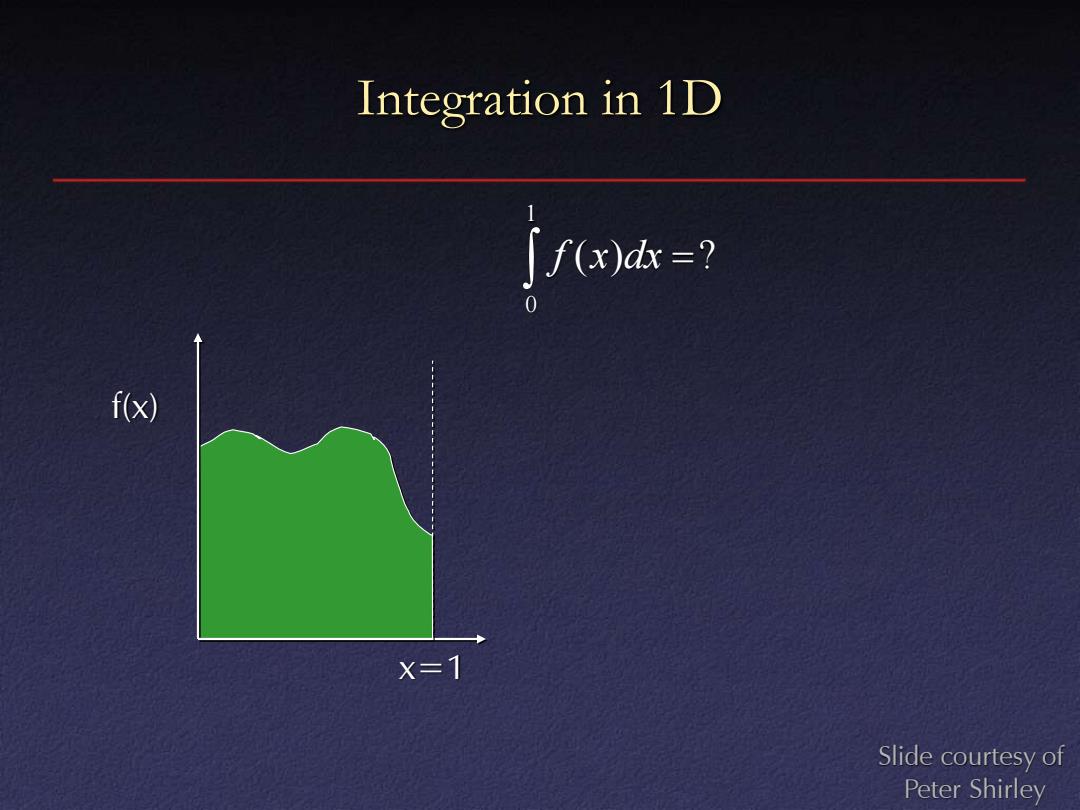
Integration in 1D ∫f(x)k=? f(x) X=1 Slide courtesy of Peter Shirley

Integration in 1D x=1 f(x) ( ) ? 1 0  f x dx = Slide courtesy of Peter Shirley

We can approximate ja寸g6a f(x) 8x) X=1 Slide courtesy of Peter Shirley

We can approximate x=1 f(x) g(x)   = 1 0 1 0 f (x)dx g(x)dx Slide courtesy of Peter Shirley

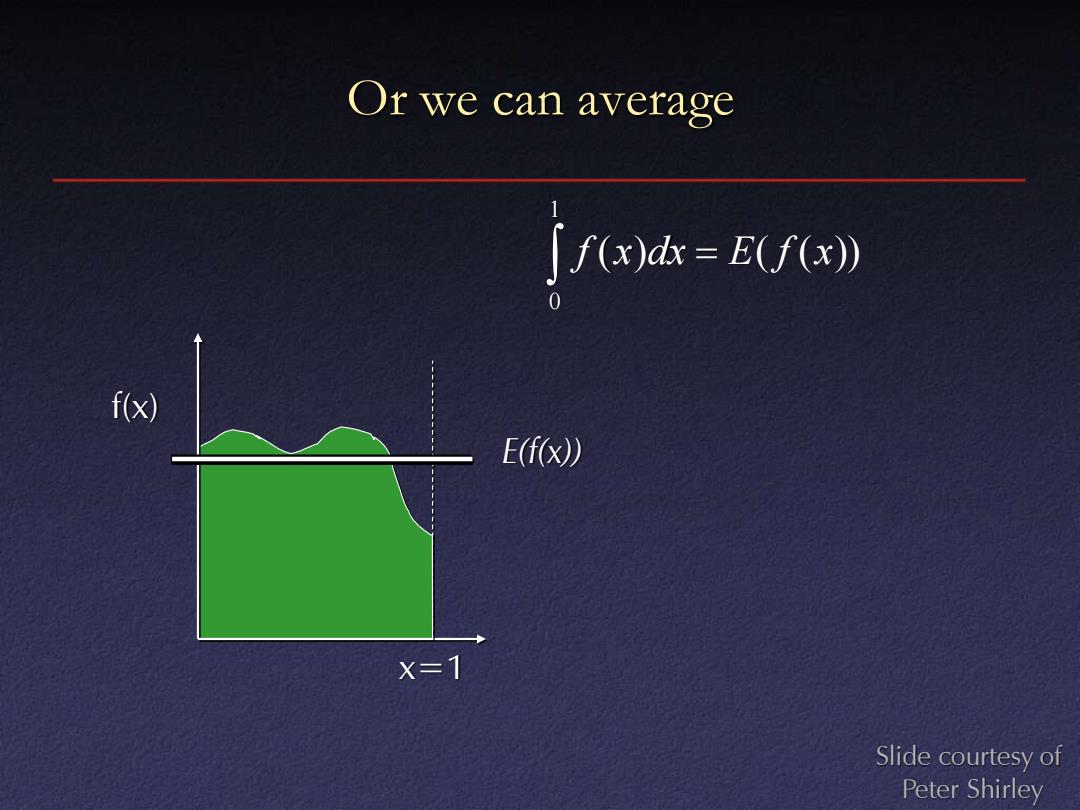
Or we can average 「fx)k=E(fx》 0 f(x) E(f(x)) X=1 Slide courtesy of Peter Shirley

Or we can average x=1 f(x) E(f(x)) ( ) ( ( )) 1 0 f x dx = E f x  Slide courtesy of Peter Shirley

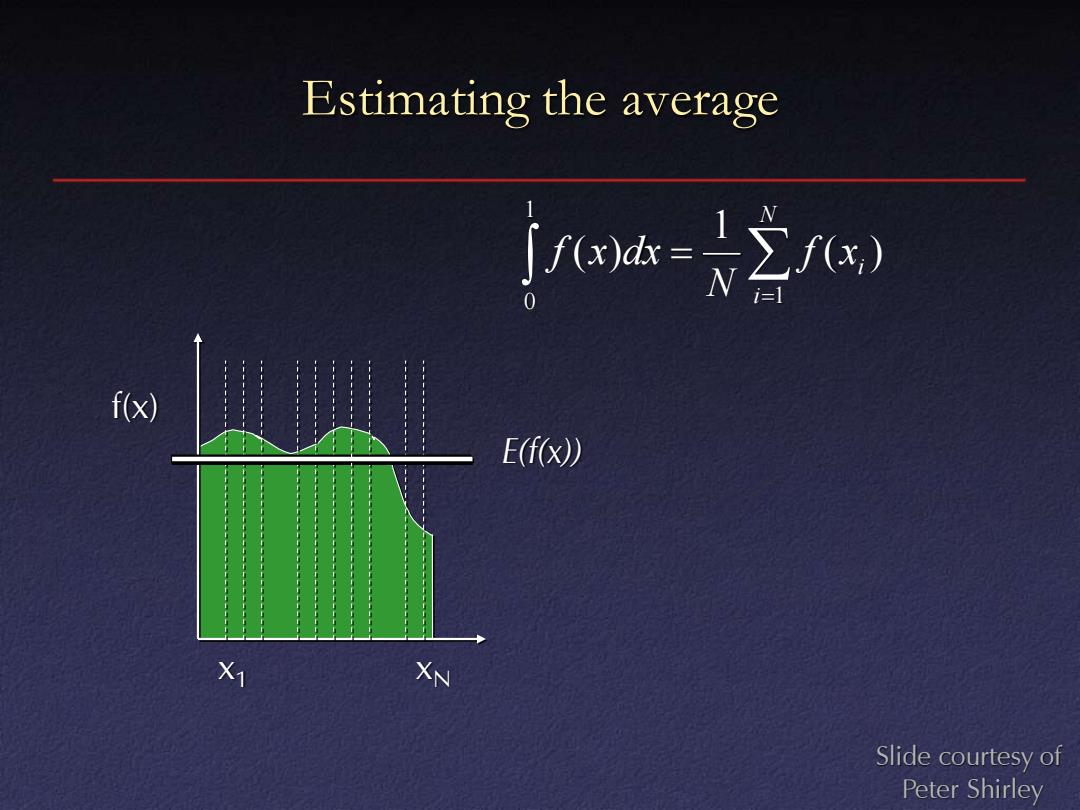
Estimating the average j3=之W f(x) E(f(x)) X1 XN Slide courtesy of Peter Shirley

Estimating the average x1 f(x) xN  = = N i i f x N f x dx 1 1 0 ( ) 1 ( ) E(f(x)) Slide courtesy of Peter Shirley