
Risk,Return,and Equilibrium:Empirical Tests STOR ® Eugene F.Fama;James D.MacBeth The Journal of Political Economy,Vol.81,No.3 (May -Jun.,1973),607-636. Stable URL: http://links.jstor.org/sici?sici=0022-3808%28197305%2F06%2981%3A3%3C607%3ARRAEET%3E2.0.CO%3B2-J The Journal of Political Economy is currently published by The University of Chicago Press. Your use of the JSTOR archive indicates your acceptance of JSTOR's Terms and Conditions of Use,available at http://www.jstor.org/about/terms.html.JSTOR's Terms and Conditions of Use provides,in part,that unless you have obtained prior permission,you may not download an entire issue of a journal or multiple copies of articles,and you may use content in the JSTOR archive only for your personal,non-commercial use. Please contact the publisher regarding any further use of this work.Publisher contact information may be obtained at http://www.jstor.org/journals/ucpress.html. Each copy of any part of a JSTOR transmission must contain the same copyright notice that appears on the screen or printed page of such transmission. For more information on JSTOR contact jstor-info@umich.edu. ©2003 JSTOR http://www.jstor.org/ Mon Nov1701:55:302003

Risk,Return,and Equilibrium: Empirical Tests Eugene F.Fama and James D.MacBeth University of Chicago This paper tests the relationship between average return and risk for New York Stock Exchange common stocks.The theoretical basis of the tests is the "two-parameter"'portfolio model and models of market equilibrium derived from the two-parameter portfolio model.We can- not reject the hypothesis of these models that the pricing of common stocks reflects the attempts of risk-averse investors to hold portfolios that are "efficient"in terms of expected value and dispersion of return. Moreover,the observed "fair game"properties of the coefficients and residuals of the risk-return regressions are consistent with an "efficient capital market"-that is,a market where prices of securities fully reflect available information. I.Theoretical Background In the two-parameter portfolio model of Tobin (1958),Markowitz (1959), and Fama (19656),the capital market is assumed to be perfect in the sense that investors are price takers and there are neither transactions costs nor information costs.Distributions of one-period percentage returns on all assets and portfolios are assumed to be normal or to conform to some other two-parameter member of the symmetric stable class.Investors are assumed to be risk averse and to behave as if they choose among portfolios on the basis of maximum expected utility.A perfect capital market,investor risk aversion,and two-parameter return distributions imply the important "efficient set theorem":The optimal portfolio for any investor must be efficient in the sense that no other portfolio with the same or higher expected return has lower dispersion of return.1 Received August 24,1971.Final version received for publication September 2,1972. Research supported by a grant from the National Science Foundation.The com- ments of Professors F.Black,L.Fisher,N.Gonedes,M.Jensen,M.Miller,R.Officer, H.Roberts,R.Roll,and M.Scholes are gratefully acknowledged.A special note of thanks is due to Black,Jensen,and Officer. I Although the choice of dispersion parameter is arbitrary,the standard deviation 607
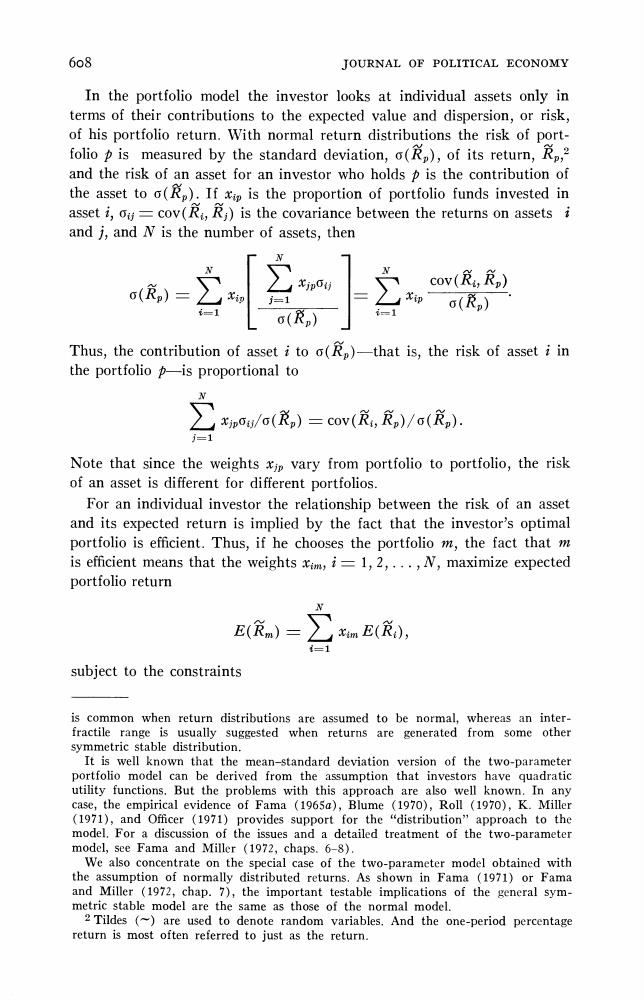
6o8 JOURNAL OF POLITICAL ECONOMY In the portfolio model the investor looks at individual assets only in terms of their contributions to the expected value and dispersion,or risk, of his portfolio return.With normal return distributions the risk of port- folio is measured by the standard deviation,()of its return,p,? and the risk of an asset for an investor who holds is the contribution of the asset to (R).If is the proportion of portfolio funds invested in asset i,oy=cov(Rj)is the covariance between the returns on assets i and j,and N is the number of assets,then (成,)= cov(Ri,R) () (R) Thus,the contribution of asset i to o(Rp)-that is,the risk of asset i in the portfolio pis proportional to xjpag/a(Rp)=cov(Ri,Rp)/a(Rp). j=1 Note that since the weights ip vary from portfolio to portfolio,the risk of an asset is different for different portfolios. For an individual investor the relationship between the risk of an asset and its expected return is implied by the fact that the investor's optimal portfolio is efficient.Thus,if he chooses the portfolio m,the fact that m is efficient means that the weightsx,=1,2,...,N,maximize expected portfolio return E(nm) xmE(), subject to the constraints is common when return distributions are assumed to be normal,whereas an inter- fractile range is usually suggested when returns are generated from some other symmetric stable distribution. It is well known that the mean-standard deviation version of the two-parameter portfolio model can be derived from the assumption that investors have quadratic utility functions.But the problems with this approach are also well known.In any case,the empirical evidence of Fama (1965a),Blume (1970),Roll (1970),K.Miller (1971),and Officer (1971)provides support for the "distribution"approach to the model.For a discussion of the issues and a detailed treatment of the two-parameter model,see Fama and Miller (1972,chaps.6-8). We also concentrate on the special case of the two-parameter model obtained with the assumption of normally distributed returns.As shown in Fama (1971)or Fama and Miller (1972,chap.7),the important testable implications of the general sym- metric stable model are the same as those of the normal model. 2 Tildes (~are used to denote random variables.And the one-period percentage return is most often referred to just as the return

RISK,RETURN,AND EQUILIBRIUM 609 o(p)=o(rm)and ∑m=1 i Lagrangian methods can then be used to show that the weights xim must be chosen in such a way that for any asset i in m E(范)一E(n)=Sm (1) where Sm is the rate of change of E(Rp)with respect to a change in (R)at the point on the efficient set corresponding to portfolio If there are nonnegativity constraints on the weights (that is,if short selling is prohibited),then (1)only holds for assets i such that xim>0. Although equation (1)is just a condition on the weights xim that is re- quired for portfolio efficiency,it can be interpreted as the relationship be- tween the risk of asset i in portfolio i and the expected return on the asset. The equation says that the difference between the expected return on the asset and the expected return on the portfolio is proportional to the differ- ence between the risk of the asset and the risk of the portfolio.The pro- portionality factor is Sm,the slope of the efficient set at the point corres- ponding to the portfolio m.And the risk of the asset is its contribution to total portfolio risk,(m). II.Testable Implications Suppose now that we posit a market of risk-averse investors who make portfolio decisions period by period according to the two-parameter model.3 We are concerned with determining what this implies for observable properties of security and portfolio returns.We consider two categories of implications.First,there are conditions on expected returns that are im- plied by the fact that in a two-parameter world investors hold efficient portfolios.Second,there are conditions on the behavior of returns through time that are implied by the assumption of the two-parameter model that the capital market is perfect or frictionless in the sense that there are neither transactions costs nor information costs. A.Expected Returns The implications of the two-parameter model for expected returns derive from the efficiency condition or expected return-risk relationship of equa- tion (1).First,it is convenient to rewrite (1)as 3A multiperiod version of the two-parameter model is in Fama (1970a)or Fama and Miller (1972,chap.8)

610 JOURNAL OF POLITICAL ECONOMY E(R)=[E(Rm)一Smo(Rm】十Smo(Rm)B, (2) where cov(Ri,R) XjmGij =1 cov(r,哀m)/o(n) (3) 2(Rm) a2(Rm) o(Rm) The parameter B.can be interpreted as the risk of asset i in the portfolio m,measured relative to (R),the total risk of m.The intercept in (2), E()=E(m)一SmG(m), (4) is the expected return on a security whose return is uncorrelated with R-that is,a zero-B security.Since B=0 implies that a security con- tributes nothing to (Rm),it is appropriate to say that it is riskless in this portfolio.It is well to note from (3),however,that since xim oi=xam o2(R)is just one of the N terms in Bi,B=0 does not imply that security i has zero variance of return. From (4),it follows that Sm= E(R)一E(Ro) a(Rm) (5) so that (2)can be rewritten E(R)=E()+[E(Rm)-E()]B: (6) In words,the expected return on security i is E(R),the expected return on a security that is riskless in the portfolio m,plus a risk premium that is B:times the difference between E(R)and E(Ro). Equation (6)has three testable implications:(C1)The relationship between the expected return on a security and its risk in any efficient port- folio m is linear.(C2)B;is a complete measure of the risk of security i in the efficient portfolio m:no other measure of the risk of i appears in (6). (C3)In a market of risk-averse investors,higher risk should be associated with higher expected return:that is,E(R)-E(R)>0. The importance of condition C3 is obvious.The importance of C1 and C2 should become clear as the discussion proceeds.At this point suffice it to say that if C1 and C2 do not hold,market returns do not reflect the attempts of investors to hold efficient portfolios:Some assets are syste- matically underpriced or overpriced relative to what is implied by the expected return-risk or efficiency equation (6). B.Market Equilibrium and the Efficiency of the Market Portjolio To test conditions C1-C3 we must identify some efficient portfolio m. This in turn requires specification of the characteristic of market equi-