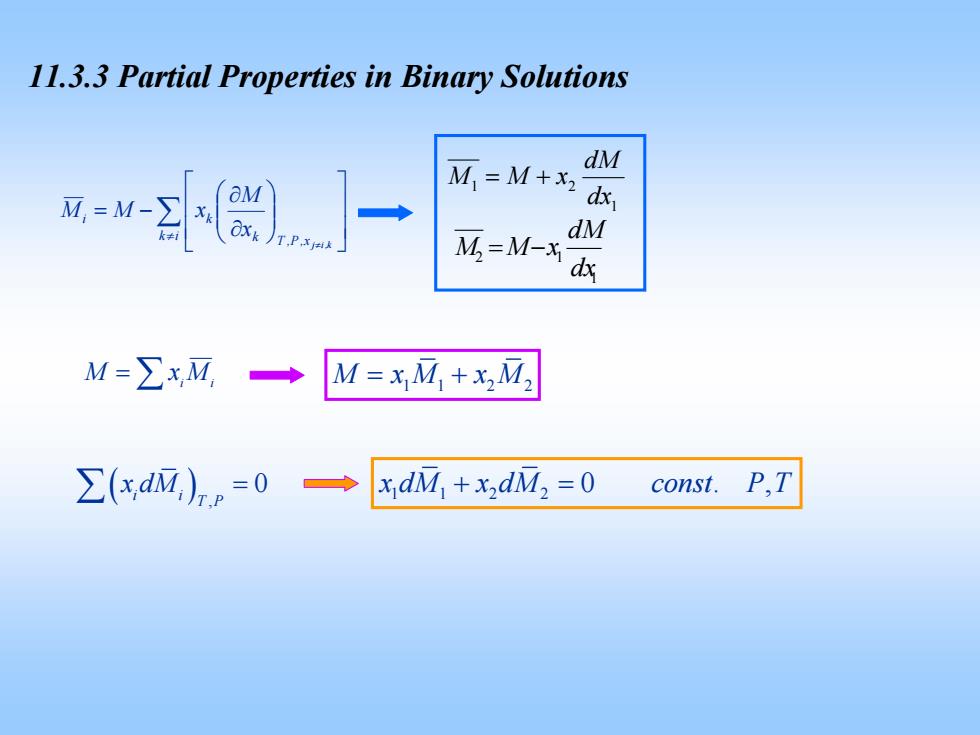
11.3.3 Partial Properties in Binary Solutions =w- M.=M+x d dM OXk )T.P M=M-X dM M=∑xM, M=xM+xM2 ∑(xdM,)p=0→ xdM+xdM,=0 const.P,T
11.3.3 Partial Properties in Binary Solutions ∑≠ ⎥ ⎥ ⎦ ⎤ ⎢ ⎢ ⎣ ⎡ ⎟ ⎟ ⎠ ⎞ ⎜ ⎜ ⎝ ⎛ ∂ ∂ −= ≠ ik k x,P,T i k k,ij x M xMM 1 2 1 dx dM −= xMM 1 1 2 dx dM += xMM = ∑ MxM ii M 11 2 2 = + xM xM ( ) , 0 i i T P ∑ x dM = 112 2 x dM x dM const P T + = 0
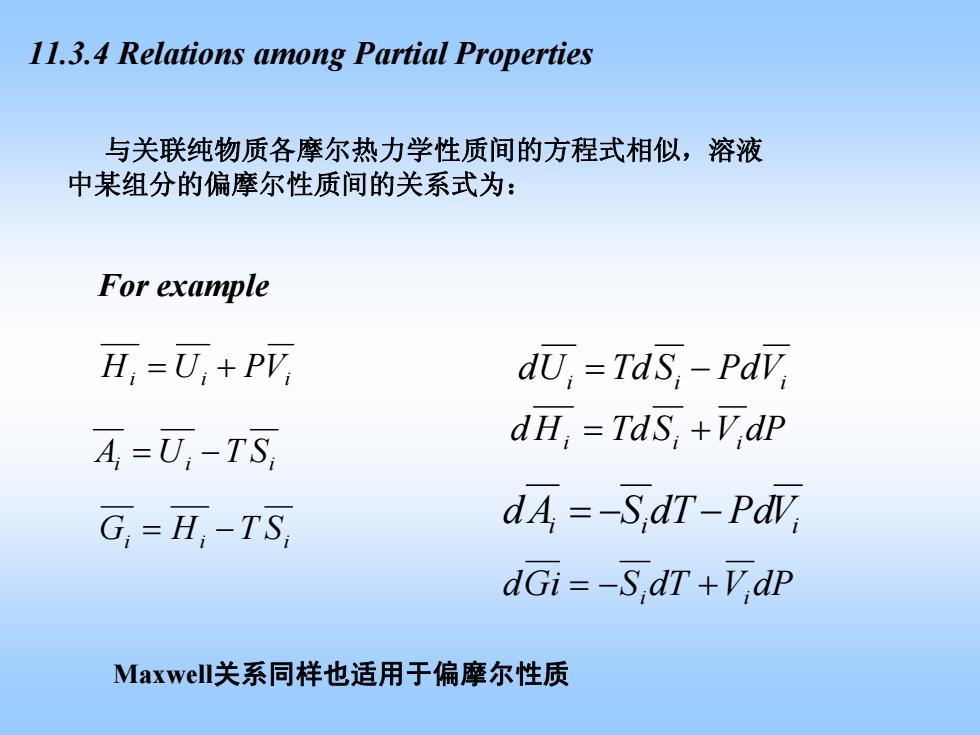
11.3.4 Relations among Partial Properties 与关联纯物质各摩尔热力学性质间的方程式相似,溶液 中某组分的偏摩尔性质间的关系式为: For example H,=可,+P7 dU,TdS,-PdV, A,=可-TS dH,TdS,+V,dp G,=H;-TS, dA=-S,dT-PaV dGi=-S,dT+V,dP Maxwell关系同样也适用于偏摩尔性质
11.3.4 Relations among Partial Properties += VPUH iii −= STUA iii −= STHG iii i i −= VPdSTdUd i dPVSTdHd i += ii ii −−= VPddTSAd i i +−= idPVdTSGid For example 与关联纯物质各摩尔热力学性质间的方程式相似,溶液 中某组分的偏摩尔性质间的关系式为: Maxwell关系同样也适用于偏摩尔性质
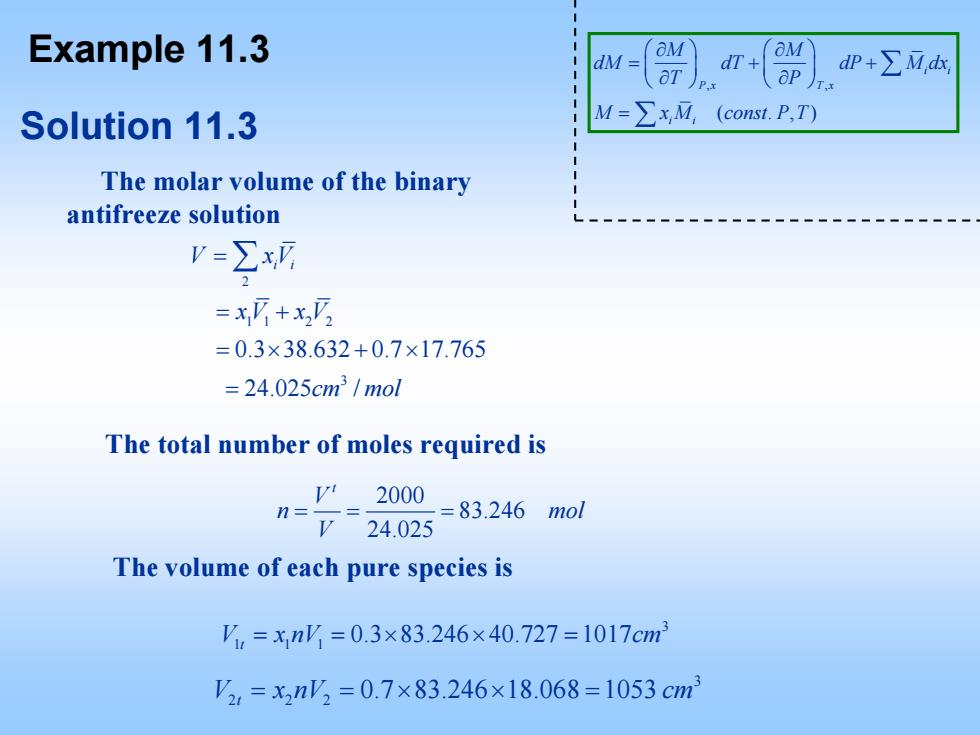
Example 11.3 OM dM= dT dP+∑M,d Solution 11.3 M=∑xM (const.P,T) The molar volume of the binary antifreeze solution V=∑x =x+x, =0.3×38.632+0.7×17.765 =24.025cm/mol The total number of moles required is n==2000 =724.025 =83.246mol The volume of each pure species is ',=xn=0.3×83.246×40.727=1017cm2 V2,=x2n'2=0.7×83.246×18.068=1053cm3
Solution 11.3 The molar volume of the binary antifreeze solution 2 11 2 2 3 0.3 38.632 0.7 17.765 24.025 / V xVi i xV xV cm mol = = + =× +× = ∑ Example 11.3 , , ( .,) i i P x T x i i M M dM dT dP M dx T P M x M const P T ⎛⎞ ⎛⎞ ∂ ∂ = ++ ⎜⎟ ⎜⎟ ⎝⎠ ⎝⎠ ∂ ∂ = ∑ ∑ The total number of moles required is 2000 83.246 24.025 t V n mol V == = The volume of each pure species is 3 1 11 0.3 83.246 40.727 1017 V x nV t = =× × = cm 3 2 22 0.7 83.246 18.068 1053 V x nV t = =× × = cm

Example 11.4 M,=M-∑ OM Solution 11.4 、Oxk,Px (a) H=400x+600x2+xx24(10x+20x2) J/mol x2=1-x7 H=400x+600(1-x)+x(1-x)[40x+20(1-x)] H=600-180x-20x3J/mol (A④) d班=-180-60x dx =0- 万,=H+k 瓦1=600-180x-20x+(1-x)(-180-60x2)) 豆1=420-60x2+40x3J/mol (B) H=H-x H 五2=600-180x-20x-x(-180-60x) 豆2=600+40xJ/mol (C)
x2 = 1-x1 ( ) 3 1 1 H =− − 600 180 20 / x x J mol A H x xx x x x = + −+ − + − 400 600 1 1 40 20 1 1 11 1 1 1 ( ) ( )⎡ ( )⎤ ⎣ ⎦ Solution 11.4 Example 11.4 H x x x x x x J mol =++ + 400 600 4 10 20 / 1 2 12 1 2 ( ) ∑ ≠ ⎥⎥⎦⎤ ⎢⎢⎣⎡ ⎟⎟⎠⎞ ⎜⎜⎝⎛ ∂∂ −= ≠ ik k x,P,T i k k,ij xM xMM ( ) 1 1 1 1 2 1 dxdH xH dxdH xHH −+=+= 2 1 1 180 60 dH x dx =− − ( )( ) 3 2 1 11 1 1 H xx x x = − − +− − − 600 180 20 1 180 60 2 3 1 11 H x x J mol B =− + 420 60 40 / ( ) 1 2 1 dx dH −= xHH ( ) 3 2 2 1 1 1 1 H x xx x = − − −− − 600 180 20 180 60 3 2 1 H = + 600 40 / x J mol C( ) (a)

Another method M a(nM) H=600-180x-20x3 T.P.n 70=600n-180xn-20x a(nH) On; JT.P.nj nH=600n-180n-20写 L i, a(nH) 3-2an 8n -60-180-20×3-207 on n n=n+n on=1 On 1 on On2 H1=420-20×3x+40x 瓦2 「a(nH) =600」 2-20n -2 on T.P,m nz 3 五2=600+40x
( ) 2 2 3 1 1 2 3 1 1 1 , , 1 1 2 600 180 20 3 20 T Pn nH n n H n n n n nn n ⎡ ⎤ ∂ ∂ − ∂ = = − −× − ⎢ ⎥ ∂ ∂ ∂ ⎣ ⎦ Another method 3 1 1 H xx =− − 600 180 20 3 3 1 1 2 600 180 20 n nH n x n x n =− − 3 1 1 2 600 180 20 n nH n n n =−− = + nnn 21 1 1 1 2 = ∂ ∂ = ∂ ∂ n n n n ( ) n,P,T j i i n nM M ⎟⎟⎠⎞ ⎜⎜⎝⎛ ∂ ∂ = ( ) , , j i i T Pn nH H n ⎡ ⎤ ∂ = ⎢ ⎥ ∂ ⎣ ⎦ 2 3 1 1 1 H = −× + 420 20 3 40 x x ( ) 1 3 2 1 3 2 22 , , 2 600 20 T Pn nH n n H n n nn n ⎡ ⎤ ∂ ∂ − ∂ = =− ⎢ ⎥ ∂ ∂∂ ⎣ ⎦ 3 2 1 H x = + 600 40